Equations/Inequalities
Quadratics
Polynomials
Graphs
Functions
Miscellaneous
100
Solve the system of equations.
3.4x + 2.5y = 11.8
6.8x – 2.7y = 8.2
(2, 2)
100
f(x) = -2x^2 + 12x - 13
When in this form, you can easily determine the ...
minimum
maximum
y-intercept
x-intercepts
y-intercept
100
Name the polynomial.
3x^3 + 2xy - 4y^2
Cubic trinomial
100
What are the solutions?
-1 and 5
100
A function is provided by the equation
b(t) = -t^2 - 3t + 10
What is b(-2)?
12
100
Which of the following sets of ordered pairs represent a function? Select all that apply.
a) (1, 2), (1, 3), (1, 4), (1, 5)
b) (2, 3), (-2, 4), (-5, 6), (5, 2)
c) (3, 4), (-2, 4), (-1, 4), (4, 4)
d) (2, 5), (3, 4), (-2, 6), (2, 7)
e) (5, 2), (-6, 3), (-2, 2), (-1, -3)
B, C, E
200
Simple interest can be calculated using the formula
I=Prtwhere P is the principal amount invested, r is the interest rate, and t is the time in years it is invested.
Solve the equation for the rate.
I/(Pt)
200
Jennifer kicks a soccer ball into the air. The height of the ball in feet can be approximated using the equation
h(t) = 30t - 15t^2
where t is time in seconds. To determine how high the ball gets, she would need to calculate what?maximum
200
If a large rectangle has a length of 3x + 4 and a width of x - 5 and a small rectangle has a length of 2x + 2 and width of x - 3, what is the difference between the large and the small areas?
x^2 - 7x - 14
200
Give the equation in slope-intercept form for the following graph:
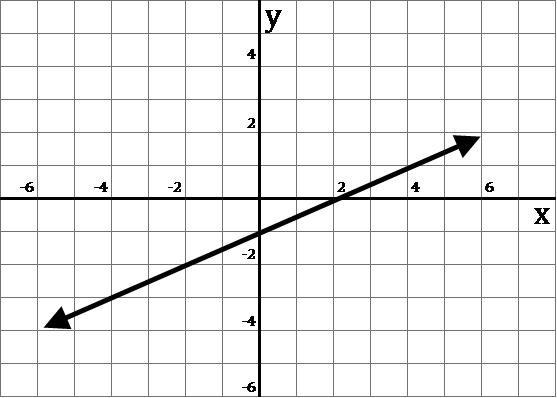
y = 1/2x - 1
200
Complete the table for the function
f(x) = (x + 2) / (x - 4)
x f(x)
-2 ?
-1 ?
1 ?
2 ?
0, -1/5, -1
200
What is the rate of change during the interval 20 ≤ x ≤ 30? x values go by 5's (it keeps cutting the numbers off, sorry :))
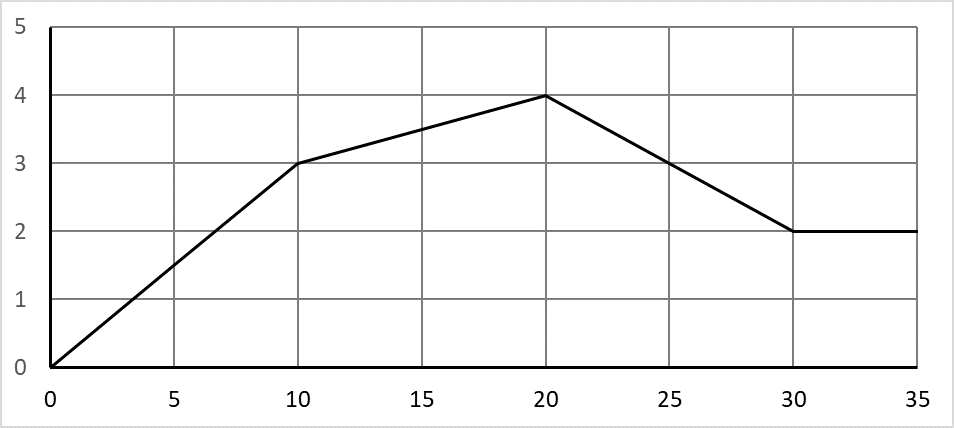
-1/5
300
Solve for x.
-2(x + 3) ≥ 3x + 9
x <= -3
300
Shannon is given the quadratic equation
g(x) = x^2 + 6x + 8
She is asked to find the vertex. What is the vertex she should have found?(-3, -1)
300
What is the perimeter of the polygon shown?
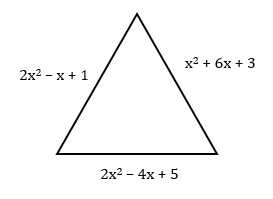
5x^2 + x + 9
300
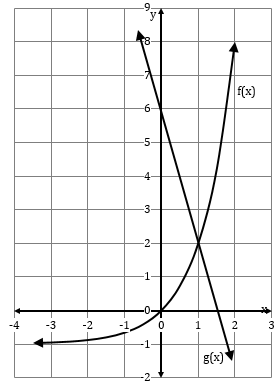
The function y = f(x) is shown on the graph below as a solid line. The function has been transformed in the form of f(x + k) and is shown by the dashed line. What is the value of k?
-4
300
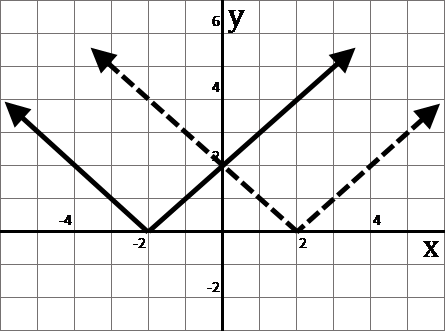
A table of values is given for the function f(x). Another function can be represented by the equation g(x) = 3x – 5. Which function has the greatest rate of change?
x f(x)
-4 -9
-2 -6
0 -3
2 0
4 3
g(x)
300
Solve
(a^2b^3)^4 / (a^2b^4)
a^6b^8
400
Stephen goes to the fair and buys two funnel cakes and one drink. His total bill is $6.75. A few days later, he goes with a friend and buys three funnel cakes and two drinks. This time his total bill is $11.
Write two equations that could be used to determine how much each funnel cake and drink costs. Use f for the cost of a funnel cake and d for the cost of a drink.2f + d = 6.75
3f + 2d = 11
400
Scott is given the quadratic function
f(x) = x^2 + 9x + 20
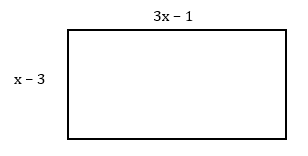
and asked to find the two zeros. What are the zeros as ordered pairs?(-4, 0) and (-5, 0)
400
What is the area of this rectangle?
3x^2 - 10x + 3
400
Give the solutions to the system.
no solution
400
A table of values is given for a function f(x). Complete the table for f(x) + 1.
x f(x) f(x) + 1
-4 5 ?
-2 7 ?
2 1 ?
4 -2 ?
6, 8, 2, -1
400
Give the integer answer and the exponent form of ∜81
3
81^(1/4)
500
Tony is doing a proof for solving the equation
-3(2x + 1) = 33. Some of his work is shown. What
is the property used in step 3?
1. -3(2x + 1) = 33
2. ?
3. -6x = 36
Addition Property of Equality
500
The height of the ball in feet can be approximated using the equation
h = 30t - 15t^2
where t is time in seconds. How long does it take for the ball to hit the ground?2 seconds
500
What is the factored form of
25m^4 - 36n^2
(5m^2 - 6n)(5m^2 + 6n)
500
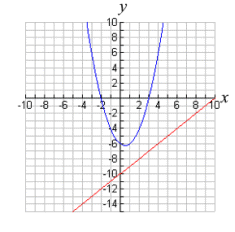
Using the graph, what is the positive solution to f(x) = g(x)?
x = 1
500
A golf ball company’s costs consist of two things. The factory costs $1550 a day to run and the materials for each golf ball is $1.20. The function that models the cost is C(g) = 1550 + 1.20g where g represents the number of golf balls produced. The domain for this function is:
A. All real numbers
B. g ≥ 0
C. All positive integers and zero
D. All positive real numbers
C
500
Population is changing exponentially. The amount of people in thousands each year of a city can be represented by the expression
25.75(1.025)^y
Does this exponential equation represent growth or decay?What is the percent rate of change each year? (Round to the nearest tenth if necessary)
growth, 2.5%