Jumping
Free Falling
Throwing/Launching
Writing Equations from Graphs
Converting to Standard Form
Area Models
100
A toy rocket travels vertically upward from ground level, and can be modeled by the equation below. How far did the rocket travel?
f(x)=-2x(x-8)
8 feet
100
What is the velocity of a free-falling object?
0 ft/sec
100
A baseball player hit a ball with a velocity if 146 feet per second and contacted the ball at a height of 3 feet above the ground. What is the starting height of the ball?
3 ft
100
Write the equation of the graph in factored form.
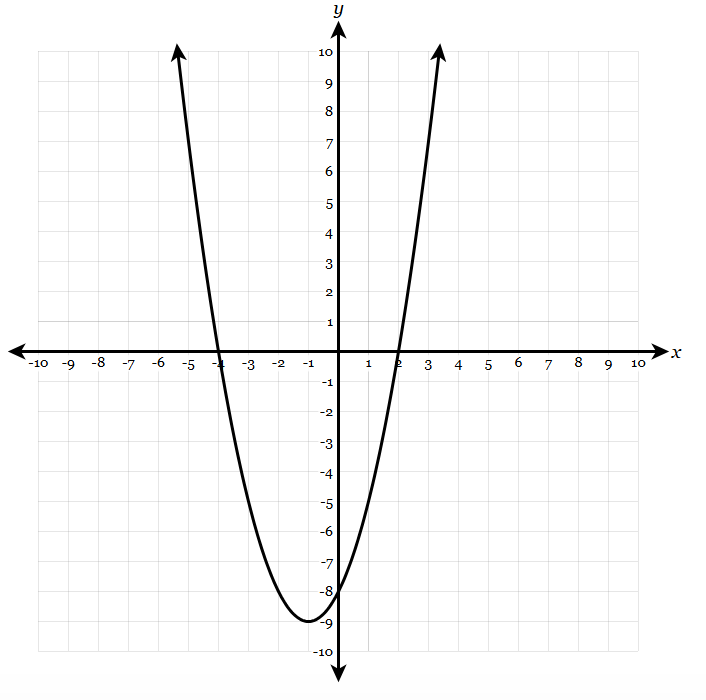
y=(x+4)(x-2)
100
Convert the following to standard form:
y=(x+3)(x-4)
y=x^2 -x-12
100
What is the formula for area?
A=lw
200
A toy rocket travels vertically upward from ground level, and can be modeled by the equation below, where x is the horizontal distance in feet and y is the height in feet. What is the label (INCLUDE UNITS) for the x-axis?
f(x)=-2x(x-8)
Distance (in feet)
200
AJ dropped his phone off of a bridge that was 80 feet above the water's surface. Write the equation to model this situation.
h=-16t^2+80
200
A baseball player hit a ball with a velocity if 146 feet per second and contacted the ball at a height of 3 feet above the ground. If the ball was caught by an opposing player in the outfield at a height of 6 feet above the ground, how long was the ball in the air? Write an equation to model this situation.
h=-16t^2+146t+3
or
6=-16t^2+146t+3
200
Write the equation of the graph in standard form.
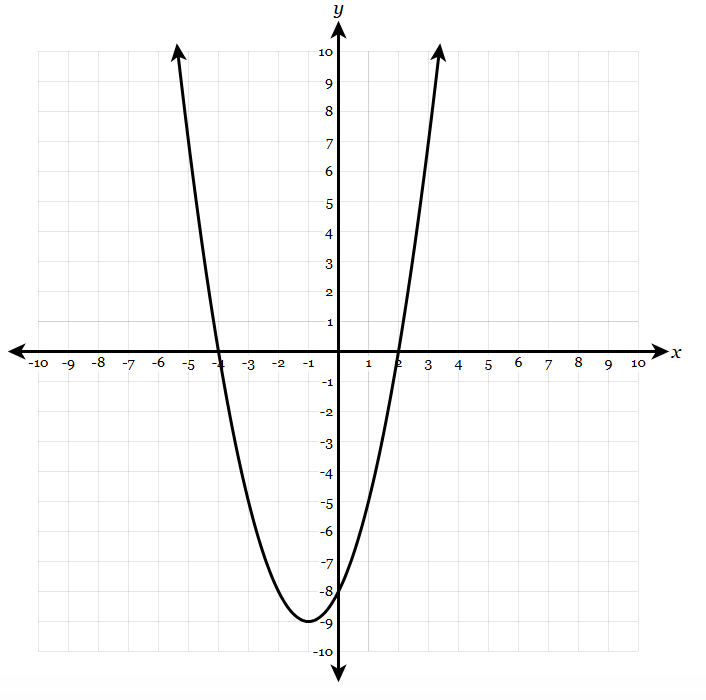
y=x^2+2x-8
200
Convert the following to standard form:
y=(x-6)^2
y=x^2-12x+36
200
The dimensions of a rectangular room are given below. If the area of the room is 27 ft2, find the length of the room.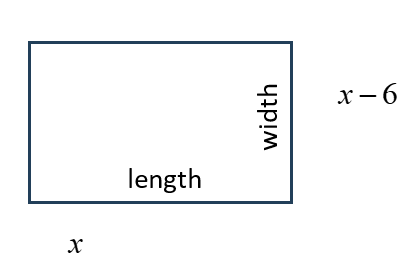 
length = 9ft
300
A toy rocket travels vertically upward from ground level, and can be modeled by the equation below, where x is the horizontal distance in feet and y is the height in feet. What is the label (INCLUDE UNITS) for the y-axis?
f(x)=-2x(x-8)
Height (or vertical distance) in feet
300
AJ dropped his phone off of a bridge that was 80 feet above the water's surface. After how many seconds will his phone hit the water? Round to the nearest hundredth.
2.24 seconds.
300
A baseball player hit a ball with a velocity if 146 feet per second and contacted the ball at a height of 3 feet above the ground. After 2 seconds, how high was the ball?
231 feet
300
Write the equation of the graph in standard form.
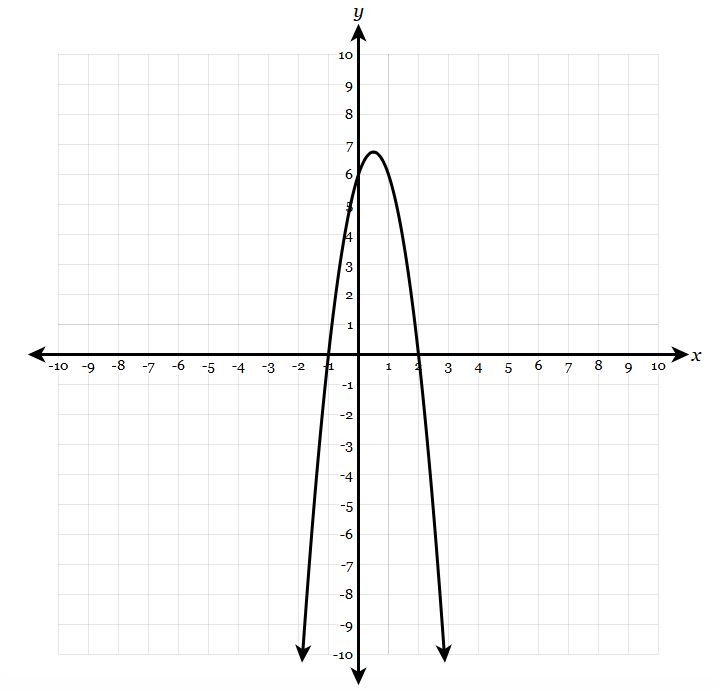
y=-3x^2+3x+6
300
Convert the following to standard form:
y=1/2(x+6)(x-2)
y=1/2x+2x-6
300
The dimensions of a rectangular room are given below. If the area of the room is 27 ft2, find the width of the room.
width = 3 ft
400
A toy rocket travels vertically upward from ground level, and can be modeled by the equation below, where x is the horizontal distance in feet and y is the height in feet. When the rocket has traveled a horizontal distance of 3.5 feet, how high in the air is it?
f(x)=-2x(x-8)
31.5 feet
400
A flowerpot fell off of an 8-story balcony many feet in the air and crashed on the ground below. If it took 3.4 seconds to hit the ground, at what height did it fall from?
184.96 feet
400
A baseball player hit a ball with a velocity if 146 feet per second and contacted the ball at a height of 3 feet above the ground. If the ball was caught by an opposing player in the outfield at a height of 6 feet above the ground, how long was the ball in the air?
9.1 seconds
400
Write the equation of the graph in vertex form.
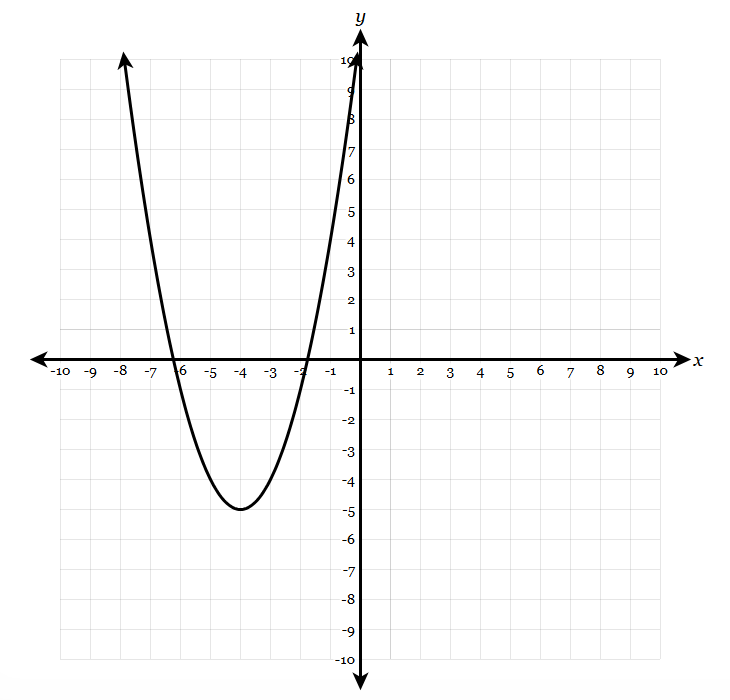
y=(x+4)^2-5
400
Convert the following to standard form:
y=(x-4)^2+3
y=x^2-8x+19
400
The area of a rectangular garden is 21 ft2. If the width is 4 less than the length, what are the dimensions of the rectangle?
length = 7 units
width = 3 units
500
A toy rocket travels vertically upward from ground level, and can be modeled by the equation below, where x is the horizontal distance in feet and y is the height in feet. How high does the rocket travel?
f(x)=-2x(x-8)
32 feet
500
A stunt man in a movie fell from a window that was 60 feet above the ground and landed on an air cushion that was 8 feet high. From the time the stunt man fell from the window, how many seconds did it take for him to hit the cushion? Round to the nearest hundredth.
1.80 seconds
500
A baseball player hit a ball with a velocity if 146 feet per second and contacted the ball at a height of 3 feet above the ground. If the ball was caught by an opposing player in the outfield at a height of 6 feet above the ground, what was the maximum height of the ball? (Round to the nearest hundredth)
336.06 ft.
500
Write the equation of the graph in vertex form.
y=-1/3(x-2)^2 +7
500
Convert the following to standard form:
-1/4(x-20)^2 -7
y=-1/4x^2+10x-107
500
The area of a rectangle is 120 ft2. If the length is 7 less than twice the width, what are the dimensions of the rectangle? Round to the nearest hundredth.
width = 9.69 ft
length = 12.38