A kids’ movie ticket costs $5.25. One day, 200 kids’ tickets were purchased. What was the total cost of those tickets?
$1050
Here are the dimensions of some rectangles. Which rectangle has an area of 12 square units and a perimeter of 14 units?
A: a length of 1 unit and a width of 12 units
B: a length of 6 units and a width of 1 unit
C: a length of 6 units and a width of 2 units
D: a length of 3 units and a width of 4 units
D!
This star was made by putting together arcs from four circles. Each small grid square represents one square unit.
Explain why the area of the star is less than 4 square units.
The star fits in a 2 by 2 square, so its area is less than 4 square units
Segment AC is a _________________________
Diameter
1/4 pi
is the area of a circle of diameter 1.
True! .5 x .5 = .25 or 1/4
A kids’ movie ticket costs $5.25. If t is the number of kids’ tickets purchased, and c is the total cost of those tickets, write an equation that relates c to t.
c = 5.25t
Colbie measured the perimeter of a square desk and found 359 cm. Kyle measured the perimeter of the same desk and found 3,579 mm. By how many millimeters do these measurements differ?
11 because 359 cm is 3,590 mm
(3590 - 3579 = 11)
The length of a string that can wrap around a basketball is 9.5 inches. What is the basketball’s radius?
1.5 in.
Segment DB is a _________________________
Radius
2pi
is the area of a circle of radius 1
False 1 x 1 = 1
A kids’ movie ticket costs $5.25. Another day, the total cost of kids’ tickets was $813.75. How many tickets were purchased that day?
155 tickets.
(813.75 / 5.25 = 155)
Sophie measured the perimeter of a square hot tub and found 588 cm. Regan measured the perimeter of the same desk and found 5,900 mm.
Why do you think Sophie and Regan may have found different measurements for the same desk?
- Sophie may have rounded her measurements of each side of the square to the nearest cm while Regan may have rounded to the nearest mm.
- The measurements are not exact and so there may have been some error in Sophie’s measurement, Regan’s measurement, or both.
A circle has a diameter of 50 cm. What is the area?
1962.5 cm or 1963.5 cm
A wheel on a car has a radius of 24 inches. How far does the car travel after 1 rotation?
150.72 in.
pi
is the circumference of a circle of radius 1
False!
2pi
Correctly calculate the perimeter of the shape below that’s composed of two squares (with side length 8 units) and two half-circles.
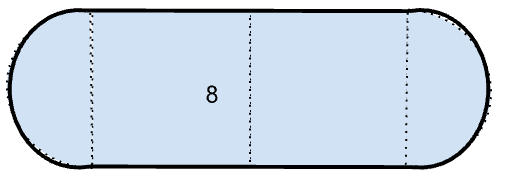
57.12 units
Each small square in the grid represents 1 square unit. Find the area of the figure in square units. Explain your reasoning.
8 square units.
Possible strategy: Draw a 3 by 6 box that just encloses the triangle, area 18 square units. The three triangles that are inside the rectangle but outside the original triangle are each right triangles with areas of 3, 3, and 4.
A rectangle is placed around a semicircle, as shown below. The width of the rectangle is 5 yards. Find the area of the shaded region to the nearest hundredth.
10.75 yd.
rectangle = 50 yd.
semi-circle = 3.14 x 25 / 2 = 39.25
50 - 39.25 = 10.75 yd
If the diameter is 20 units, the radius is ________ units.
10 units
pi
is the constant of proportionality relating the diameter of a circle to its circumference
True!
A class measured the radius and circumference of various circular objects. The results are plotted on the graph.
Does there appear to be a (somewhat) proportional relationship between the radius and circumference of a circle?
Yes!
A map of Utah is shown. Which area is closest to the area of Utah in square kilometers?

A: 274,963
B: 244,905
C: 225,808
D: 1,997
C!
The amount of trim outside a circular track is about 141.4 meters. What is the track’s diameter, rounded to the nearest hundredth?
45.03
because c = (3.14)d so you can divide 141.4 by 3.14.
A circle has a diameter of 40 cm. What is the circle’s circumference? (use 3.14 for pi)
125.6 cm
pi
is the constant of proportionality relating the radius of a circle to its area.
True!

