Unit 1
Unit 2
Unit 3
Unit 4
Unit 5
100
What is the solution to this system of equations?
-5y + 13 = x - 4
2y + 2 = 4x
x=2
y=3
100
Factor: x2 + 4x - 12
(x + 6)(x - 2)
100
Consider a box with a square base. The perimeter of the base plus the height of the box measures 125 cm. Write an equation that represents the volume of the box, V, as a function of s, the length of one side of the square base.
V= -4s3 + 125s2
100
33x+2 = 9
x= 0
100
Solve: x + 2 = √ (x+4)
x = 0
x DOES NOT equal -3
200
What is the solution to the system of three equations?
4x - 4y + 4z = -4
4x + y - 2z = 5
-3x -3y -4z = -16
(1, 3, 1)
200
What is the product of (-2 + 4i)(3 + 2i)
-14 + 8i
200
Find the 4th term of (x + 2y)5
80x2y3
200
Joe invests $500 in a savings account earning 3.2% interest compounded quarterly. Sally invests $500 in a savings account earning 2.5% compounded continuously. How much does each person have after 10 years?
Joe: $687.69
Sally: $642.01
200
Sovle 3√ (x-10) = -3
x= -17
300
If f(x)= x2 + 4 and g(x)= x-2 find f(g(x))
x2 - 4x + 8
300
Write a quadratic function that shows reflection over the x-axis, stretch by 4, left 2 and down 6
y= -4(x + 2)2 - 6
300
Factor: 8x3 + 27y3
(2x + 3y)(4x2 - 6xy + 9y2)
300
Jessica invests $1500 in a savings account that earns 3% interest compounded daily. How long will it take for Jessica to earn $600 in interest?
11.2 years
300
Find the inverse relation of the function f(x)= x2 - 2
f-1(x)= +/- √ (x+2)
400
A factory makes 12 pairs of shoes every hour it is in operation. It also costs the factory $224.23 to make each pair of shoes. Write a function C(s(h)) that shows the cost to operate the factory based on the hours it is open.
C(s(h))= 2,690.76h
400
The function h(t)= -4t2 + 16t + 20 models the height in meters of a ball t seconds after it is thrown.
What is the y-intercept, what does it represent?
When does the ball hit the ground?
What is the vertex, what does it represent?
y-intercept- (0, 20) - the height the ball started at
at 5 seconds the ball hits the ground
vertex: (2, 36) at 2 seconds, the ball is 36 meters in the air
400
Factor: 50x4 + 90x2 - 72
(5x2 + 12)(10x2 - 6)
400
Describe the domain, range, and asymptote of f(x)= log3(x+4) + 5
Domain: (-4, infinity)
Range: (-infinity, infinity)
Asymptote: x= -4
400
Find the inverse of the function f(x)= x3 + 12
f-1(x)= 3√ (x-12)
500
The school play sold student tickets and adult tickets. On day one, they sold 4 student tickets and 2 adult tickets and made $26. On day two, they sold 6 student tickets and 5 adult tickets and made $49. How much was each ticket?
student ticket- $4
adult ticket- $5
500
A parabola has diretrix x = -3, focus at (5, 3), and vertex at (1, 3). Write the equation of the parabola.
x= 1/16 (y-3)2 + 1
500
Divide: 12x3 - 11x2 + 9x + 18 / 4x + 3
3x2 - 5x + 6
500
Write an exponential equation to model the table:
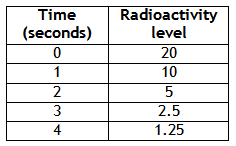
f(x)= 20(0.5)x
500
What is an appropriate domain restriction so that the inverse of f(x)= 4(x + 2)2 - 13 will be a function?
x≥ - 2 or x≤ -2