The base of a cone is a ______. Therefore, the parallel or horizontal cross section is also a ______.

circle
In order to be a triangle the sum of the 2 shorter sides must be _____________ than the third side
Greater
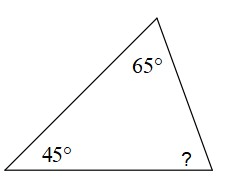 Find the missing angle
Find the missing angle
70 degrees
Find the measure of <b & <c

<b= 116 degrees
<c= 64 degrees
Vertical angles are always...
Equal or congruent
The sides of all PRISMS are _____. So, the perpendicular or vertical cross-section of this triangular prism is a _______.
rectangle
TRUE or FALSE: Will the following side lengths be a triangle?
1, 2, 5
FALSE
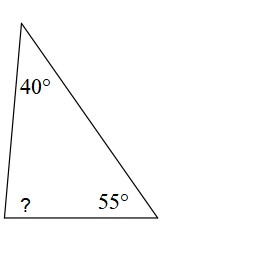
85 degrees

a: 40 degrees
b: 66 degrees
Name a pair of vertical angles
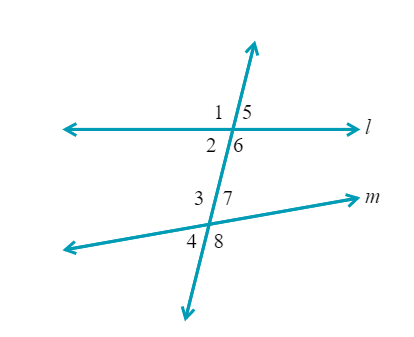
<2 & <5
<7 & <4
<3 & <8
Draw the correct shape of the cross-section pictured
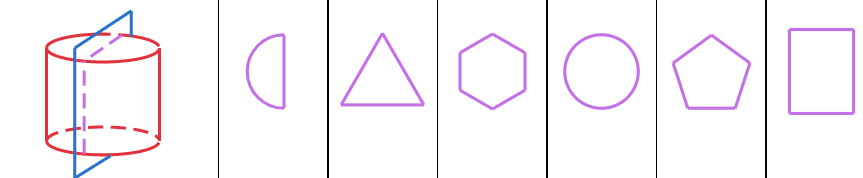
Rectangle
TRUE or FALSE: Will the following side lengths be a triangle?
28, 14, 15
TRUE
Two interior angle measures of a triangle are 74 degrees and 53 degrees. What is the measure of the third interior angle?
53 degrees
If a=65 degrees, what does b=?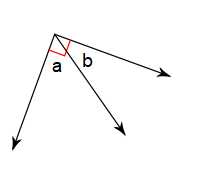
b=25
Name the sides/rays of <TQR. MUST use the correct symbol(s).
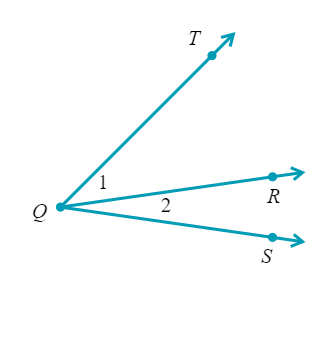
-->QT
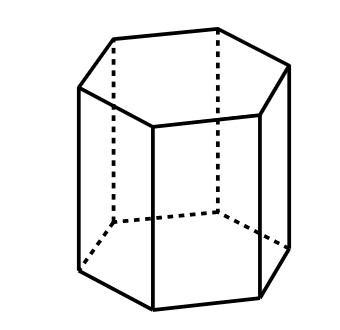
Select the type of cross-section formed when a hexagonal prism is cut perpendicular to its base.
a) Hexagon
b) Square
c) Rectangle
d) Circle
rectangle (sides are rectangles)
TRUE or FALSE: Will the following side lengths be a triangle?
12.5, 20.25, 7.75
FALSE
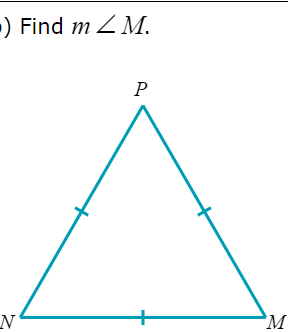
60 degrees
Solve for x: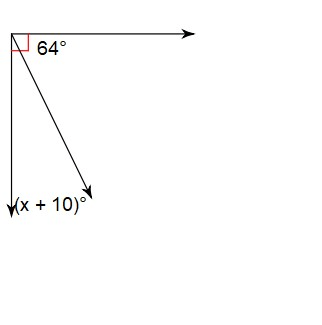
x=16
Find the missing angle
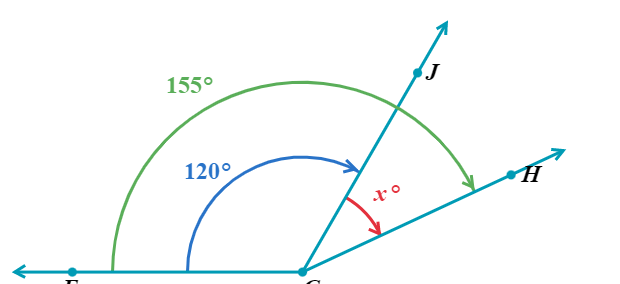
x=35 degrees
A solid is cut PERPENDICULAR to its base, forming a cross-section in the shape of a TRIANGLE.
Which of the following solids could have resulted in that cross-section?
a) Rectangular Pyramid
b) Triangular Prism
c) Right Pentagonal Prism
d) Cube
Rectangular Pyramid
Which set of side lengths CAN be a triangle?

11, 10, 18
Solve for x.
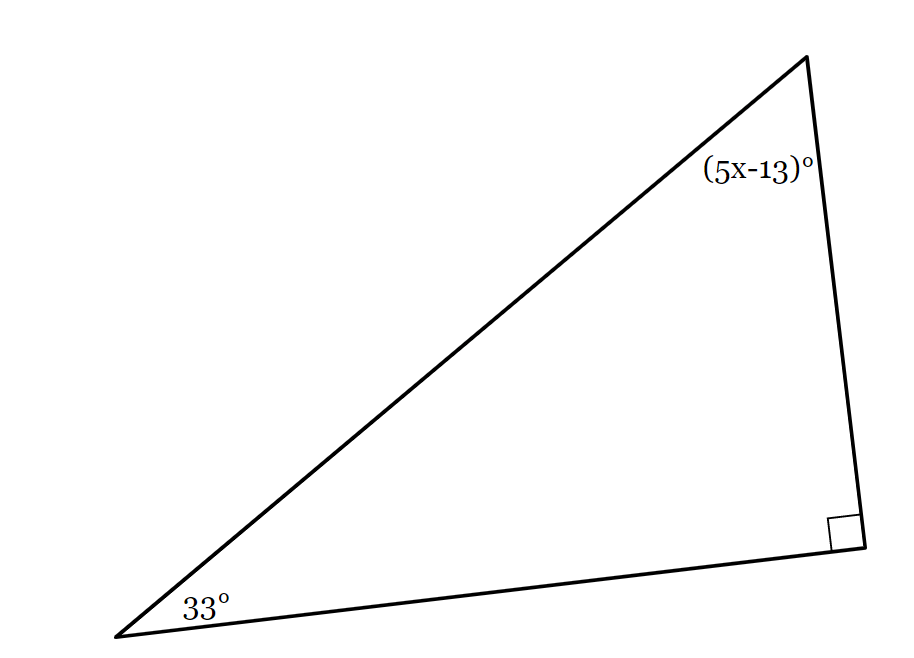
x= 14 degrees
Solve for x: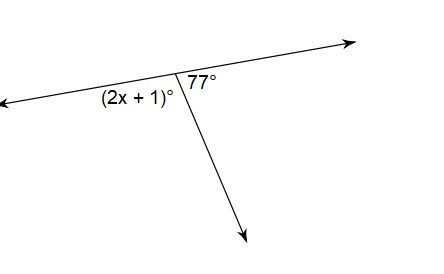
x = 51
Find x:
x=45