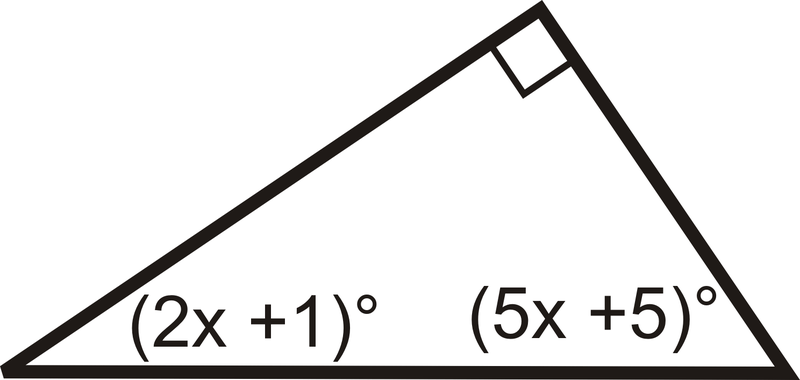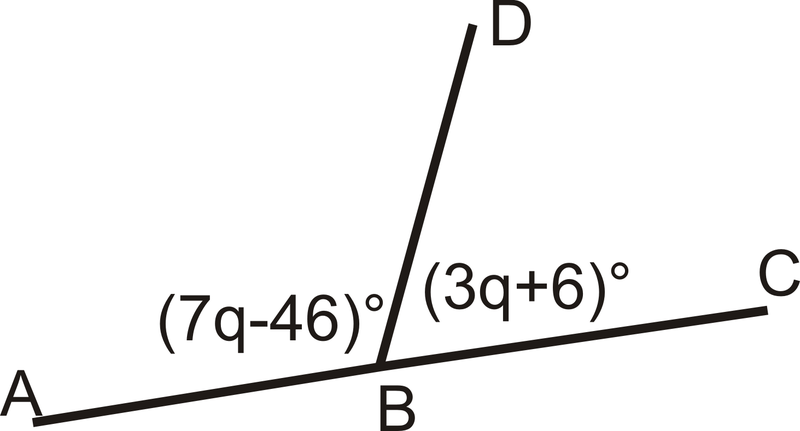Identifying Angles
Classifying Triangles
Angle Sum Theorem
Triangle Inequality
Angle Pairs
100
What is the name of the angle that measures 180 degrees?
Straight Angle
100
A triangle with 3 equal sides and angles.
equilateral triangle
100
The sum of all the interior angles of a triangle.
180o
100
State the triangle inequality theorem.
the sum of any two sides of a triangle must be greater than the third side
100
Angle Pairs that measure 90 degrees.
complementary
200
Identify the angle and determine its measurement.
Right angle which is 90o
200
Classify the triangle by its angles.
acute triangle
200
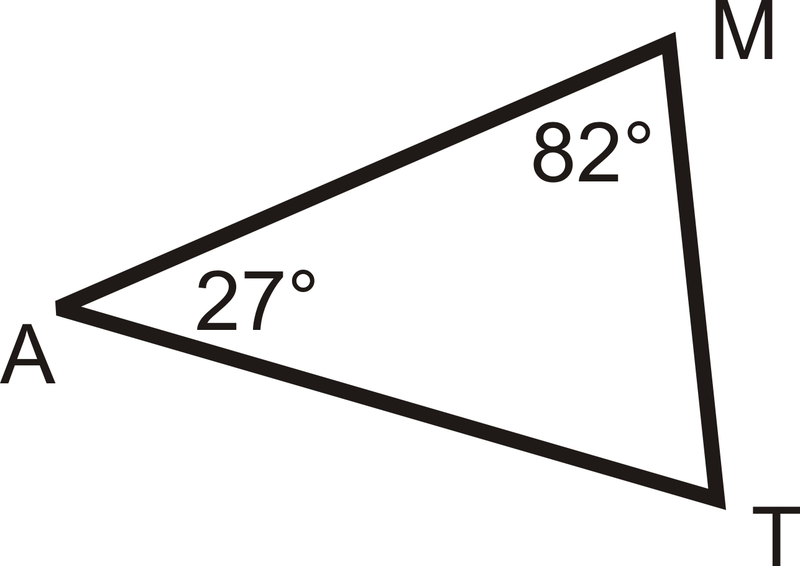
Determine the missing angle.
71o
Work:
82 + 27 + x = 180
109 + x = 180
x = 180 - 109
x = 71
200
Determine if a triangle can be drawn with the sides 11, 12, and 17.
yes
Work:
11 + 12 > 17
11 + 17 > 12
12 + 17 > 11
200
Angle Pairs that add up to 180 degrees.
supplementary
300
Identify the kind of angle.
obtuse angle
300
Classify the triangle by its sides.
isosceles triangle
300
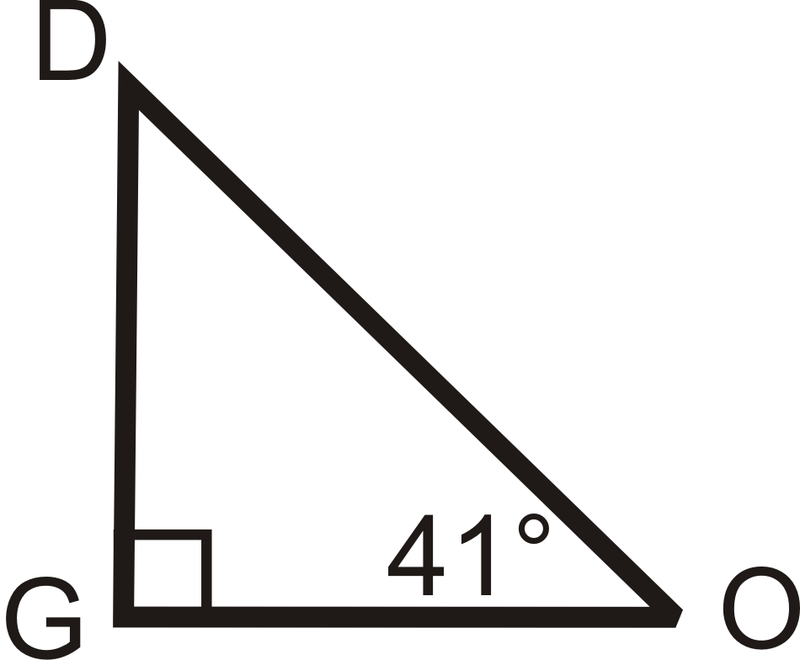
Determine the missing angle.
49o
Work:
90 + 41 + x = 180
131 + x = 180
x = 180 - 131
x = 49
300
Determine if a triangle can be drawn with the sides 4, 8, and 15.
no
Work:
4 + 8 < 15
300
Identify the angle pair relationship and solve for x.
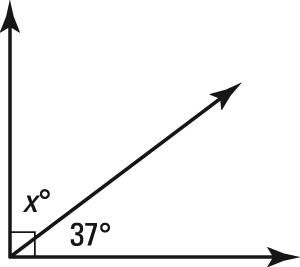
complementary, x = 53o
Work:
x + 37 = 90
x = 90 - 37
x = 53
400
The measure of an acute angle.
less than 90o
400
Classify the triangle by its sides and angles.
scalene, obtuse triangle
400
Use the angle sum rule to determine the value of x.
x = 12o
Work:
90 + 2x + 1 +5x + 5 = 180
96 + 7x = 180
7x = 84
x = 12
400
The missing side (x) must be at between ___ and ___ .
between 3 and 10
Work: 3 + 8 = 11, but if x is bigger than 10 it will become the longest side .
(and the inequality wont work)
400
Identify the angle pair relationship and solve for x.
vertical angles, x = 49
Work:
126 = x + 77
126-77 = x
49 = x
500
Identify the angle that doesn't belong.
26o
84o
13o
92o
92o angle
Work: the rest of the angles are acute (less than 90o)!
500
Classify triangle ABC by its sides, and identify the missing angle measure.
isosceles, x = 40
Work: Isosceles angles have to sides of the same measure, and two angles of the same measure. So x must be the same as the other angle, which is 40o.
500
Use the angle sum rule to determine the value of x.
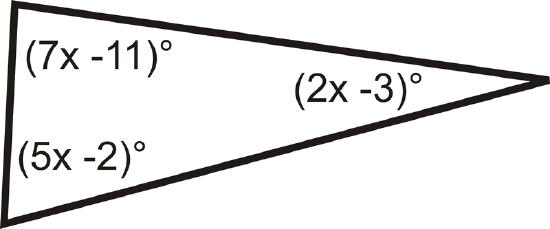
x = 14
Work:
7x - 11 + 2x - 3 + 5x - 2 = 180
14x - 16 = 180
14x = 196
x = 14
500
Identify which set of numbers could NOT make a triangle.
(a)6,8,11
(b)9,12,19
(c)7,11,18
(d)5,6,7
(c)
Work: 7 + 11 = 18
This is not greater than the other side (18) so these numbers cannot form a triangle
500
Identify the angle pair relationship and find the measure of q.
supplementary, q = 22
Work:
(7q−46) + (3q+6) = 180
10q − 40 = 180
10q = 180 + 40
10q = 220
q = 22