SSS
SAS
ASA
Types of angles
100
Are these triangles congruent? If so, tell how
SSS
100
Are these triangles congruent through SAS? Explain
Yes, they share the common side length of line segment BD.
100
Are these triangles congruent? If so, tell how
No, since the congruent sides are not in the same position.
The sides are across from different angles.
100
A pair of angles that add up to 90 degrees
What are complementary angles
200
Can we prove these triangles are congruent? If yes, tell how.
SAS
200
Are these triangles congruent through ASA? Explain
Yes (triangles share the side between the two congruent angles)
200
Congruent angles formed by intersecting lines
What are vertical angles?
300
Are these triangles congruent thru SSS? If so, write the congruence statement.
Not congruent thru SSS
300
Is this an example of triangle congruence through SAS?
No, triangles are not congruent through SAS.
300
Do we have enough information to prove that these 2 triangles are congruent?
No (angles are congruent but sides may be proportional)
300
A pair of angles are supplementary. One angle has a measure of 2x. The other angle has a measure of 3x - 15. What is the value of x?
x = 39
400
Can you prove congruence through SSS? Explain.
You can prove congruence through SSS because the shared side length is also congruent.
400
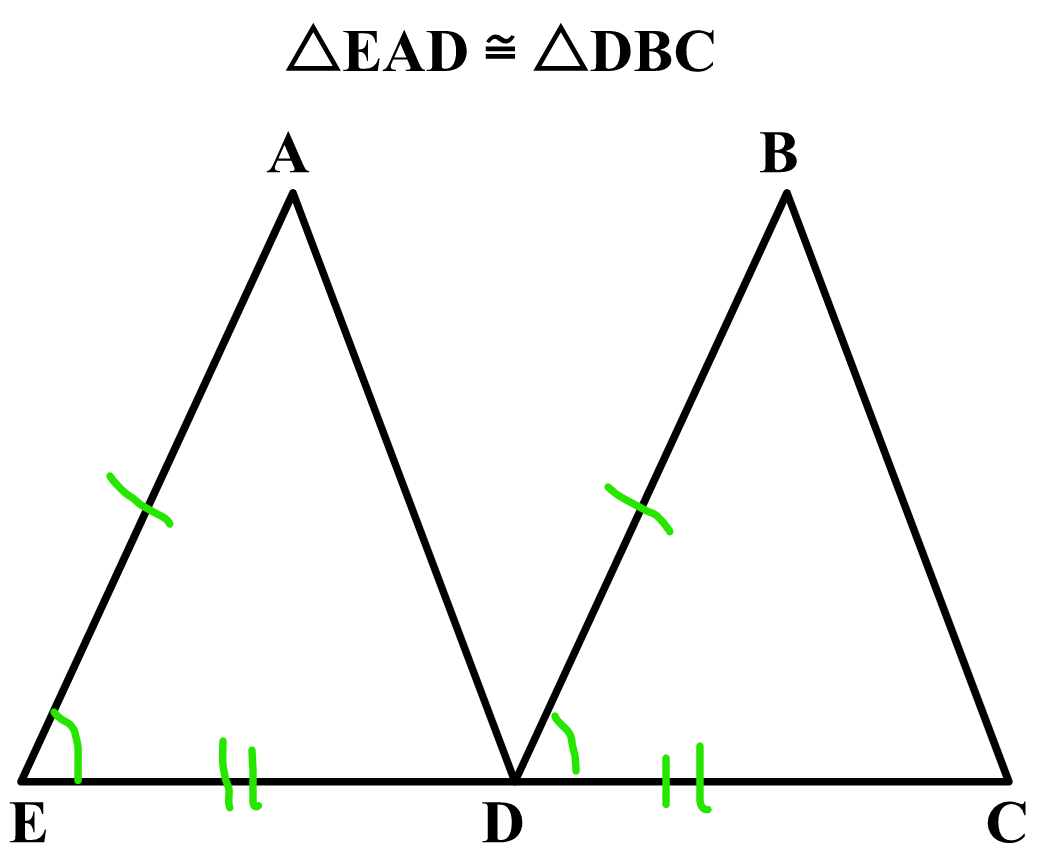
Label the triangle diagram below so that you could use SAS to prove them congruent.
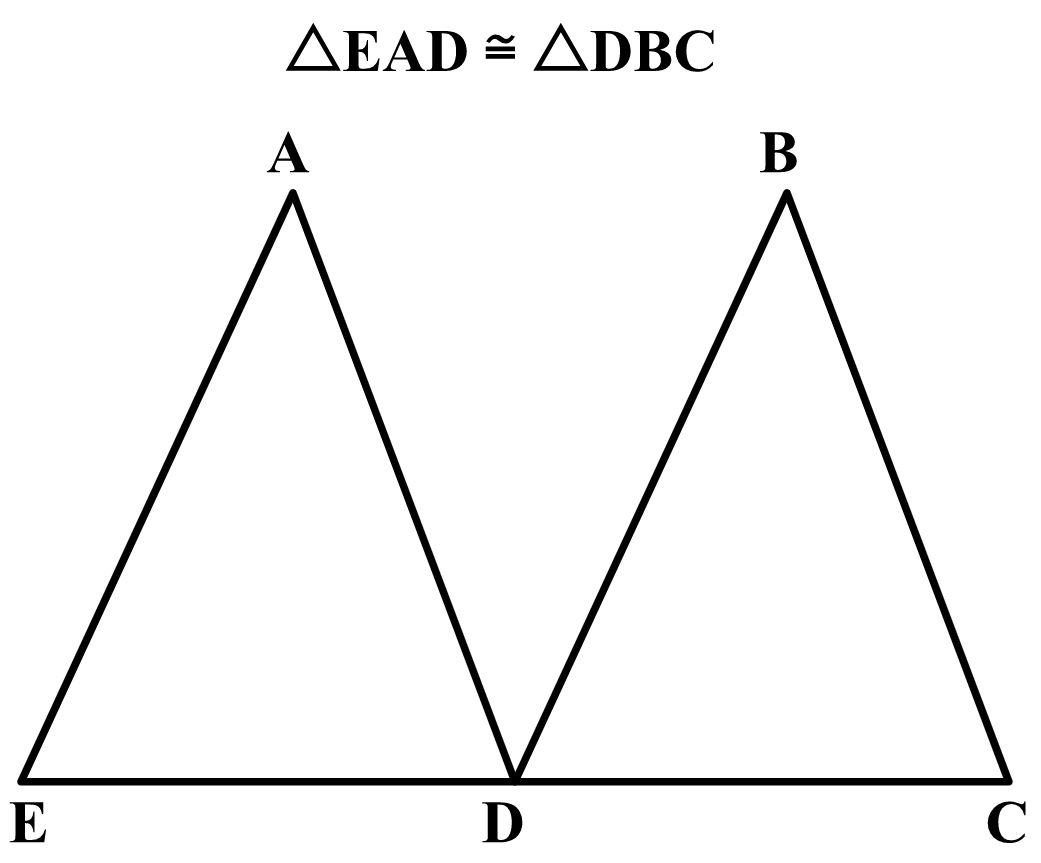
Answers may vary
400
In a pair of vertical angles, one angle measures 7x + 17. The other angle measures 67 degrees. What is the value of x?
x = 12
500
Do we have enough information to prove that these 2 triangles are congruent through SAS?
Yes, the third angle will be congruent. Since 2 angles are already congruent, the third one must also be congruent. Additionally, the triangles share the third side length.
500
Are these triangles congruent? If so, tell how
ASA
500
In a pair of complementary angles, one angle measures 4x + 8 and the other angle measures 5x + 10. How many degrees are in each of the angles?
40 and 50