What is the solution to the system
y = x + 4
y = 2x + 5
(-1,3)
Is the point (4,1) in the solution set? Why or why not?
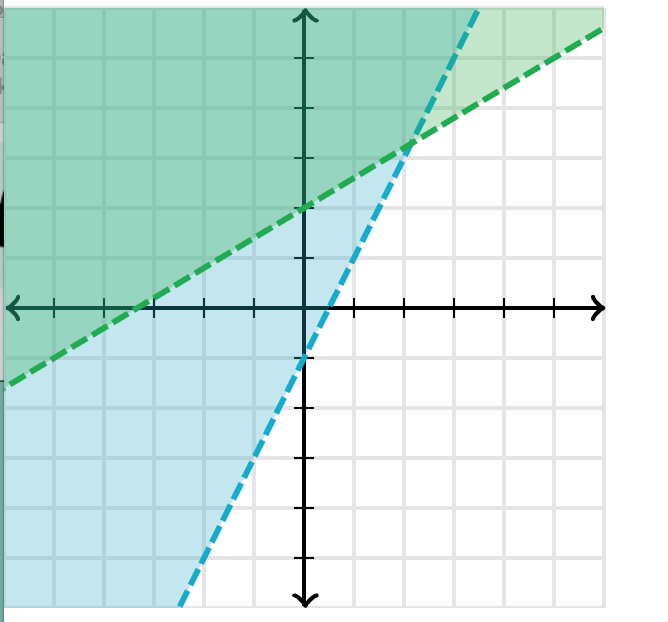
No- not in double shaded area.
Mike has two jobs as a cook and as a baby sitter. He works 'c' hours as a cook making 15 dollars an hour and 'b' hours as a baby sitter making 22 dollars an hour. This week he can work a max of 20 hours total but wants to make at least 200 dollars.
set up a system of inequalities to represent this situation.
..
Describe how you would shade y < 3x + 4.
Also would the line be solid or dotted?
Underneath and dotted.
y = 3x - 2
y = -x - 2
Name 3 points that are in the solution set.
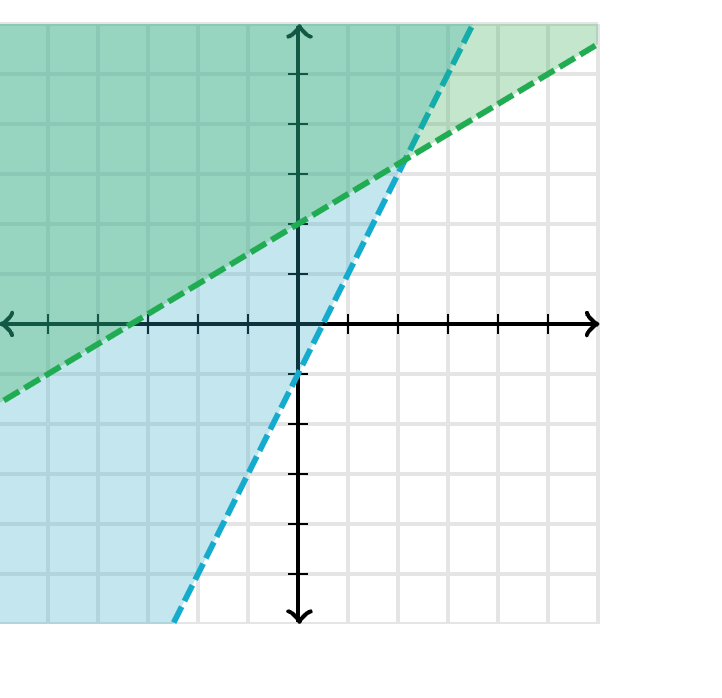
Many answers.
Jake has 30 coins in total, all dimes and quarters. In total he has 6 dollars.
Set up a system of inequalities to represent that situation.
..
Give a point that is in the system
y < x + 2 and x > 3
many answers.
y = -3
x = 5
The solution to the system:
2x + 5y =30
3x + 5y = 15
What is (-15, 12)?
A bakery sells brownies and cookies. Brownies cost 'b' dollars and cookies cost 'c' dollars. Yesterday they sold 18 brownies and 24 cookies to make a total of 81 dollars. Today they sold 10 brownies and 30 cookies to make a total of 70 dollars. Set up a system of equations.
.
Is (3, -4) a solution to 3x - y > 13
No. 13 = 13 (not greater)
y = (1/3)x - 3
2x - y = 8
Solve algebraically
y=3x-2
5x-2y=2
(2,4)
Mike has two jobs as a cook and as a baby sitter. He works 'c' hours as a cook making 15 dollars an hour and 'b' hours as a baby sitter making 22 dollars an hour. This week he can work a max of 20 hours total but wants to make at least 200 dollars.
Can he work for 14 hours as a cook and 5 as a baby sitter. Show work
Yes
y - 3x = 3
y = 3x - 2
(1/3)x + (1/4)y = 10
(1/3)x - (1/2)y = 4
Jake has 30 coins in total, all dimes and quarters. In total he has 6 dollars.
Set up a system of inequalities to represent that situation.
How many of each coin does he have?
20 Q and 10 D