If, y=a(b)x , what does 'a' represent?
a=initial amount or y-intercept
If b>1, the function is an exponential ____________ function.
growth
Rabbit populations can double (increase by 100%) every month. If there are 10 rabbits on a farm, how many rabbits will be on the farm after 4 months?
160
What is the percent growth/decay rate?
y=5(0.5)^x
50%
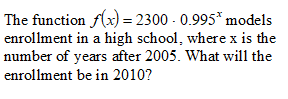
2243
What is the initial value for the function
f(x)=2(3)x
2
If 0<b<1, the function is an exponential ____________ function.
decay
An ______________ is a line that a curve approaches as it tends to infinity.
asymptote
What is the percent growth/decay rate?
y=5(1.3)^x
30%
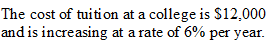
What will be the cost in 17 years?
$32,313.27
What is the y-intercept and range?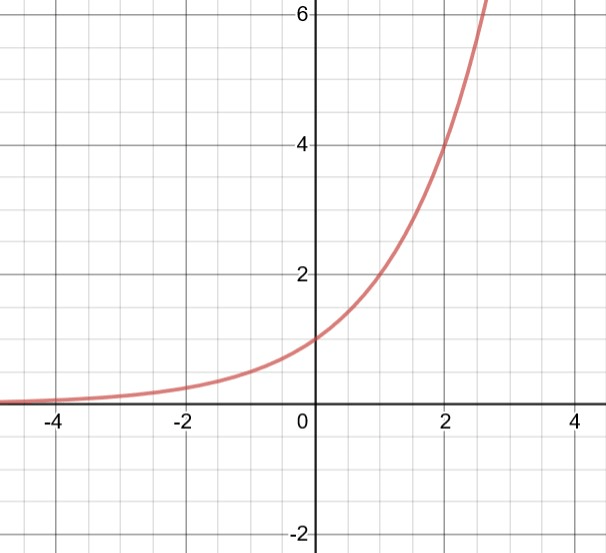
y-intercept = (0, 1)
range y > 0
f(x)=2(.93)x
Does this functions represent exponential growth or decay? What is the percent growth/decay rate?
Exponential Decay
Multiplier .93
1-.93 = .07, which means 7% rate
Check (1-.07)= (.93)
f(x)=-4(1.07)x
Does this functions represent exponential growth or decay? What's the percent growth/decay factor?
Exponential Growth. 7%.
What is the percent growth/decay rate?
y=(0.01)^x
99% Decay
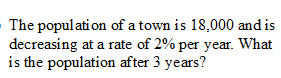
16,941 people
What's the multiplier?
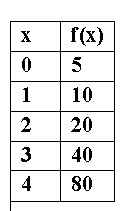
b=2
Write an equation to represent a town with an initial population of 1,400 people and an annual growth rate of 1.25%
f(x)= 1400 (1 + 0.0125)x
f(x)= 1400(1.0125)x
Does the graph represent exponential growth or decay?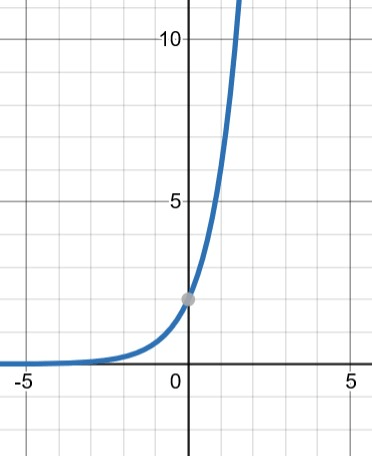
Exponential Growth
Is this exponential growth or decay?
What is the percent growth/decay rate?
y=1/2(0.7)^x
Decay. 30%
Is the function exponential growth or decay? What is the multiplier (common ratio)? 
Decay (.50) or
1/2
What is the difference between linear functions and exponential functions?
Linear functions have a constant difference or rate of change
Exponential functions have a multiplier or common ratio
Ms. Wiggins purchased a car for 26,400 and every year it decays by 12%. What can she expect the value of her car to be after 3.5 years?
f(x)=26400(.88)3.5=$16,876.92
Annual sales of a fast food restaurant are $530,000 and increasing at a rate of 5%. What will the annual sales be in 6 years?
530,000(1.05)6 =$710,250.69
What is the percent growth/decay rate?
y=60(1.33)^x
33% Growth
If you put $500.00 into an account and the account earns 3% interest each year. How much will be in the account after 5 years?
$579.64