Are You Having Fun(ction)?
Am I a function?
What's my function?
Key Features of Graphs
Domain & Range
Miscellaneous
100
This word is used to describe the set of all x-values for a function.
What is the domain?
100
What is No, not a function? It does not pass the vertical line test.
100
f(-3) if f(x) = 2x +1
What it -5 ?
100
Function gives the weight "w" of a dog, in pounds, when it is m months old. Which statement represents the meaning of the equation
w(10) = 30
in this situation?
The dog weighs 30 pounds at 10 months old.
100
Find the DOMAIN:
{(-2, 4), (3, 2), (2, 10)}
What is {-2, 3, 2}?
100
f(n)=2n+1
g(n)=3n
Find f(n)+g(n)
5n+1
200
This word is used to describe the set of all y-values of a function.
What is the range?
200
What is Yes?
200
Let f(x) = x2 + x + 1; find f(-5).
What is 21?
200
The shaded region is increasing, decreasing, or constant.
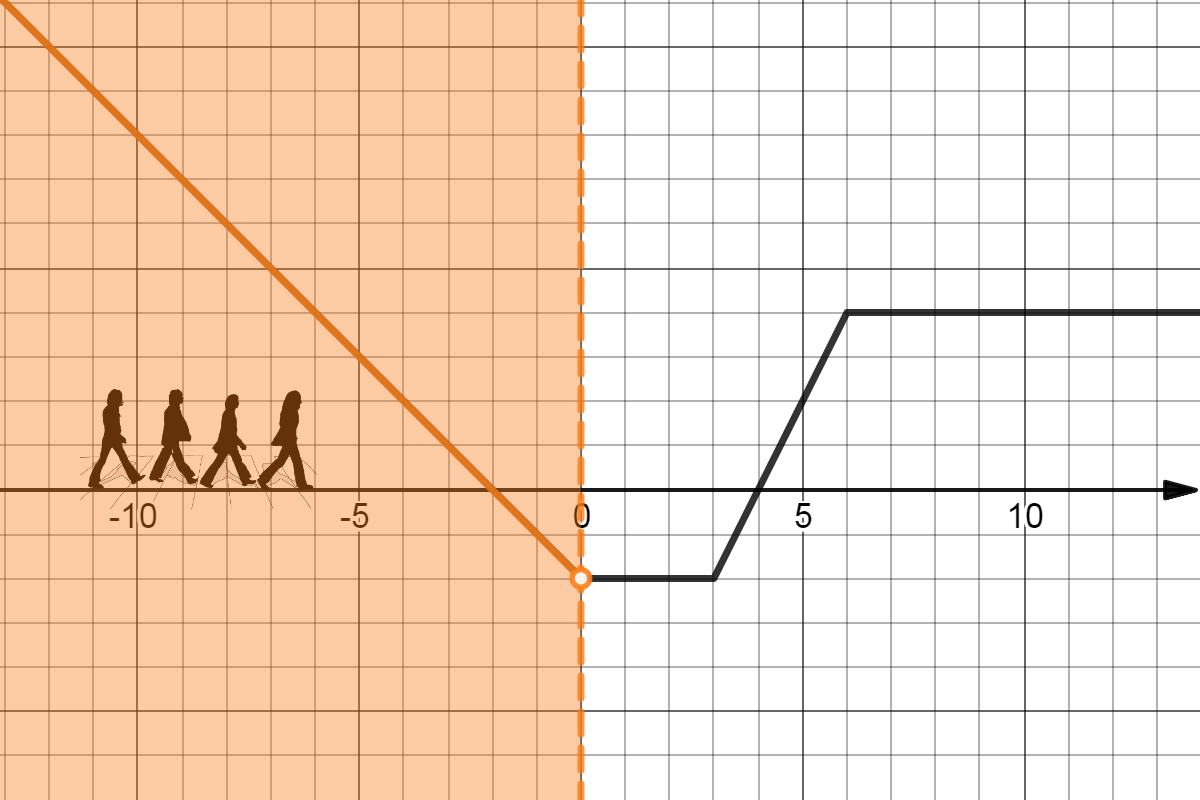
Decreasing Interval
200
What is the range? {(2, 7), (2, 8), (5, 11)}
The range is {7, 8, 11}
200
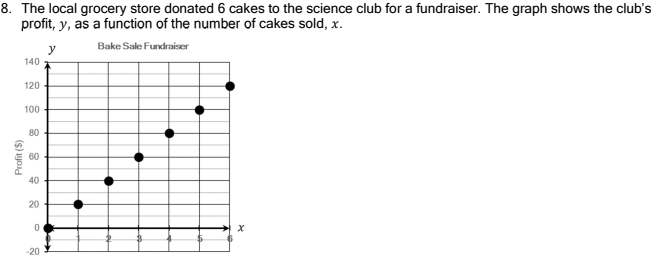
What is the RANGE of the situation?
{0,20,40,60,80,100,120}
300
What is the maximum value of a graph?
The largest y- value of the graph.
300
The mapping diagram shows a function or not.
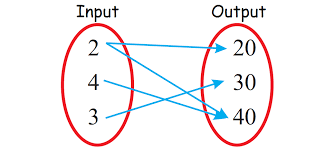
What is No, not a function?
300
Based on the graph of the function, what is f(4)?
f(4) = -2
300
What is the first increasing interval for this graph? What two points (x-values)?
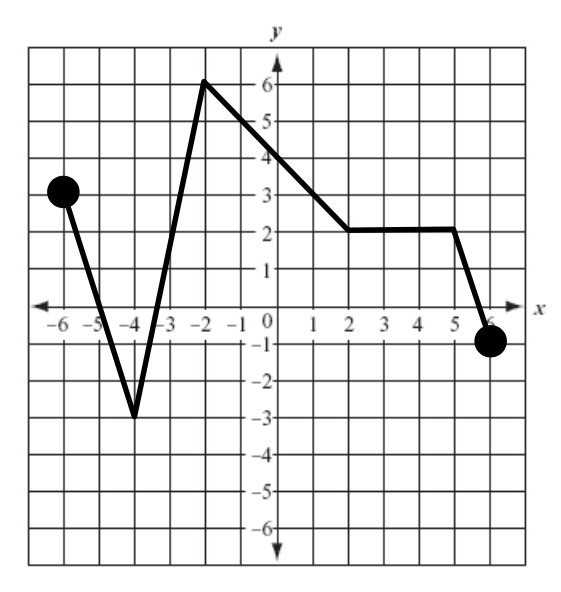
[-4,-2]
300
Find DOMAIN:
all real numbers
(neg infinity, pos infinity)
300
f(x)=2x+1
g(x)=2x-5
Find
f(x)-g(x)
6
400
How do you determine if a GRAPH is a function or not?
What is the Vertical Line Test?
400
Does the following table represent a function?
x y
1 2
2 5
6 2
2 9
5 1
What is No, because the input (x) of 2 has two different outputs (y)?
400
Evaluate this function for P(x)=20
F(x)= 5x+5
P(3)=20
400
The y-intercept of the graph.
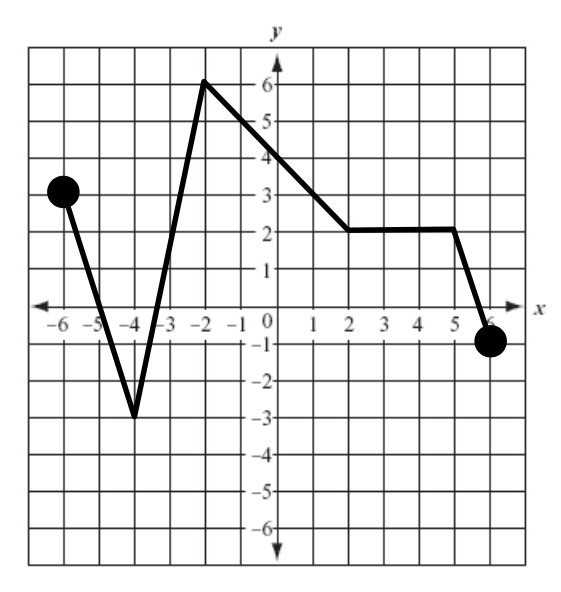
What is (0, 4)?
400
Find the DOMAIN:
-5<=x<=8 or (-5,8]
400
Anna drops a ball from a third-story window. This equation represents the approximate height, h, in meters, of the ball above the ground after it falls for t seconds.
h(t) = -5t^2 + 45
Evaluate h(2). Enter your answer as a number.
25
500
Is this a function? {(2, 3), (3, 7), (4, 10)}
Yes
500
Is this a function and why?
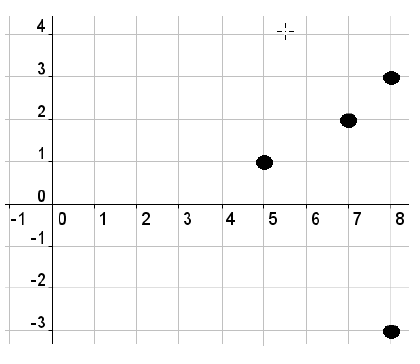
What is no, because x's repeat at 8?
500
Find f(4) =
0
500
f(x) = 2x^2 + 12x + 16
Use function notation "F(x) = ?" to represent this statement.
4 hours after she left, the temperature was 57 degrees.
f(4) = 57
500
Find the RANGE:
-3<=y<=2 or (-3, 2]
500
f(x) = 4x + 1
Find
f(3)
f(3) = 13