Domain and Range
Completing the Square
Function Transformation
Graphs and their Roots
100
The range of :
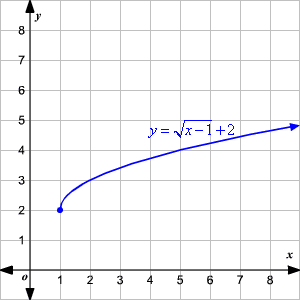
What is y >= 2 or some variation?
100
Representation of the Vertex Form in Algebra Tiles
What is y = (x+3)2 + 2
100
The parent function is y = x2. The transformation that result in the function y = x2 + 3
Vertical shift up 3 units or some variation
100
The number of unique roots in this equation
What is three?
200
The Domain of
What is x >= 1 or some variation.
200
Algebra Tile Representation of theVertex Form Equation
What is f(x) = 2(x+3)2 - 7
200
The present function is y = g(x). The transformation that results in the new function y = g(x-4)
What is a translation right by 4 units
200
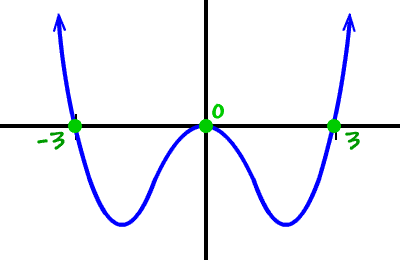The total number of factors in this equation. Be careful.
What is 4?
300
The Range of f(x) = (x-2)2 + 3
What I y >= 3 or some variation?
300
The vertex form of y = x2 -12x +40
What is (x - 6)2 + 4
300
The present function is y = r(x). The transformation that results in the new function y = -r(x)
What is reflection on the x-axis?
300
The number of times this function cross the x-axis. f(x) = (x - 2)(x - 3)(2x - 4)3
What is 3?
400
The Domian of f(x) = (x-3)2 + 4
What is all real numbers of some variation?
400
The vertex form of y = x2 + 7x + 8
What is (x + 3.5)2 - 4.25
400
The present function is y = b(x). The transformation that results in the new function y = 0.5*b(x-4)
What is. translation right 4 units and a vertical shrink by a factor of 1/2.
400
The lowest possible degree of this polynomial
What is 6?
500
the domain of f(x) = 2/(x - 3)
What is all real numbers expect for 3 or some variation.
500
The vertex form of y = 5x2 + 20x + 11
What is 5(x+2)2 - 9
500
The present function is y = k(x). The transformation that results in the new function y = k(-x-4) + 5.
A translation up 5 units right 4 units and reflected across the y-axis.
500
The lowest possible degree of this polynomial
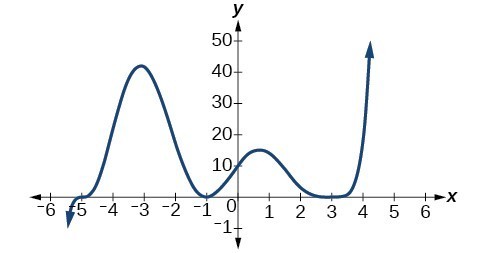
What is 7?