Congruence
Proofs 1
Proofs 2
Transformations
Definition
100
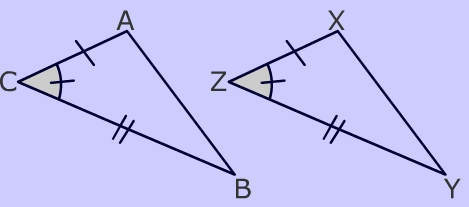
Name the method used to prove the triangles are congruent.
SAS
100
Name the five ways to prove triangles are congruent.
SSS, SAS, AAS, ASA, SSA (with the opposite side of given angle is longer)
100
Name what CPCTC stands for
Corresponding Parts of Congruent Triangles are Congruent.
100
When geometric shape is flipped across a line and what is the line called?
reflection and line of reflection
100
Something that cuts a geometric object into two equal parts
bisector
200
Are these triangles congruent? If so, name the method.
Yes and ASA
200
Name the reason that segment QR is congruence to segment QR.
The segments are congruent to themselves. Reflexive property
200
Name the important feature to remember when naming congruent figures?
Write them in corresponding order
200
When a geometric shape is slided from one place to another
translation
200
Two lines that meet at a right angle
perpendicular lines
300
Yes or No? Is it possible to prove the triangles above are congruent by ASA?
no (should be SSS)
300
Name the reason used to prove
angle
ACB is congruent to
angle
ECD.
The vertical angle theorem.
300
AK is the angle bisector of angle JKN. Name the two congruent angles in corresponding order.
angleJKA congangleNKA
300
When a geometric shape is moved in a circular motion
rotation
300
A line that intersects two parallel lines
transversal
400
triangle ABC congtriangleJKI
400
These are used to show that two figures are congruent by mapping one onto the other.
Rigid motions
400
Something we already proved and accept
Theorem
400
When a geometric figure is increased or decreased in size
dilation
400
Definition of congruent figures
Figures that have the same size and shape.
500
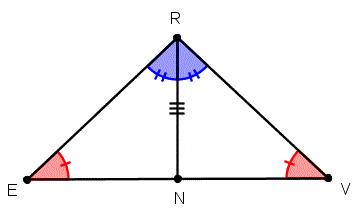
Using the markings on the triangles name the method used to prove the triangles are congruent and write a congruence statement.
AAS
triangleENR congtriangleVNR
500
Name the two methods that don't work to prove triangles are congruent.
AAA and SSA (with opposite side of given angle is shorter)
500
What theorem does this statement show?
In triangleFGH and triangleABC,
if bar(FG) cong bar(AB), bar(GH) cong bar(BC), and angleG cong angleB
then triangleFGH cong triangleABC.
Side Angle Side Theorem
500
This is preserved in all rigid transformations (except dilation)
size
500
Rectangles and squares have these feature in common
congruent diagonals and angles