Geometry Proof Basics
Triangle Properties
SSS, SAS, ASA, HL & AAS Theorems
Name that Reason
Miscellaneous
100
The main statements we say at the beginning of a proof?
What is the given information?
100
How do we know that <AEC=<DEB below?
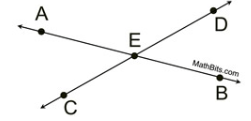
What are vertical angles?
100
Side Side Side
What is SSS
100
What is the "Reason" for Statement #1 below?
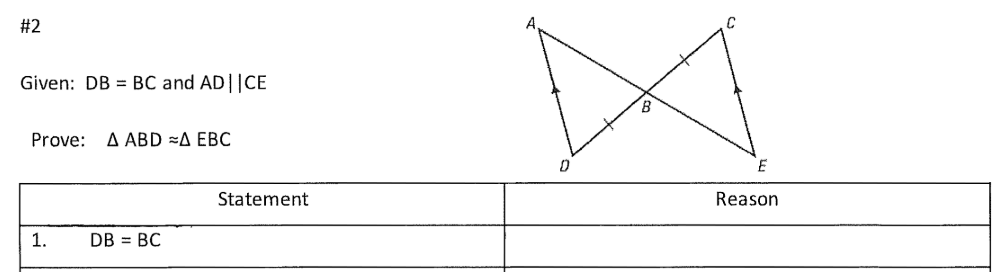
What is "given"?
100
The number of sides on a heptagon
What is seven?
200
The method of writing out the steps of a proof in complete sentences.
What is a paragraph proof?
200
How do we know that CA=CA below?
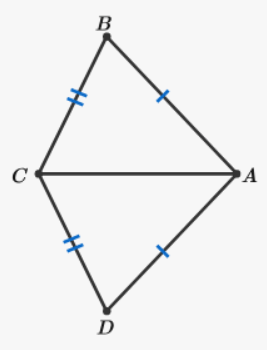
Line segments are congruent to themselves. Reflexive Property.
200
If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then these two triangles are congruent.
What is SAS
200
What is the reason for statement #3 below?
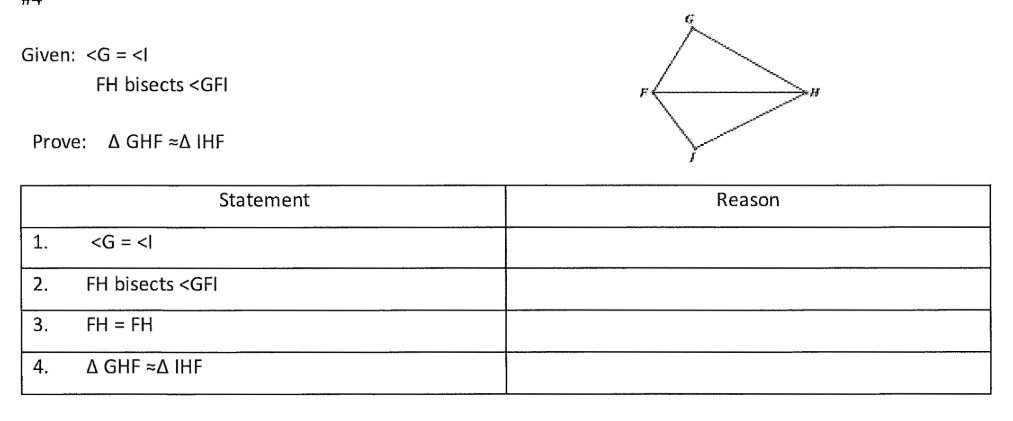
Line Segments are congruent to themselves. Reflexive Property
200
Name the 4 main triangle congruence theorems we learned.
(Not including SSA)
What are SSS, SAS, AAS, ASA?
300
A statement that looks like below is:
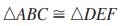
What is a Congruence Statement?
300
Name an angle that point "E" is the vertex of?
1) <AEC (or <CEA)
2) <DEB (or <BED)
3) <AED (or <DEA)
4) <CEB (or <BEC>
NOTE:
300
If two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then these two triangles are congruent.
What is ASA
300
What is the reason for statement #3 below?
What are vertical angles?
300
Given the congruence statement below, what side on the 2nd triangle is congruent to side CB?
What is FE?
400
What do we call the side across from the right angle in a right triangle?
What is the hypotenuse?
400
In the triangle below, if "D" is the midpoint of AC, what is AD congruent to?
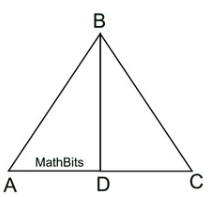
What is DC?
400
If two angles and the non-included side one triangle are congruent to two angles and the non-included angle of another triangle, then these two triangles are congruent.
What is AAS
400
What is the reason for statement #2 below?
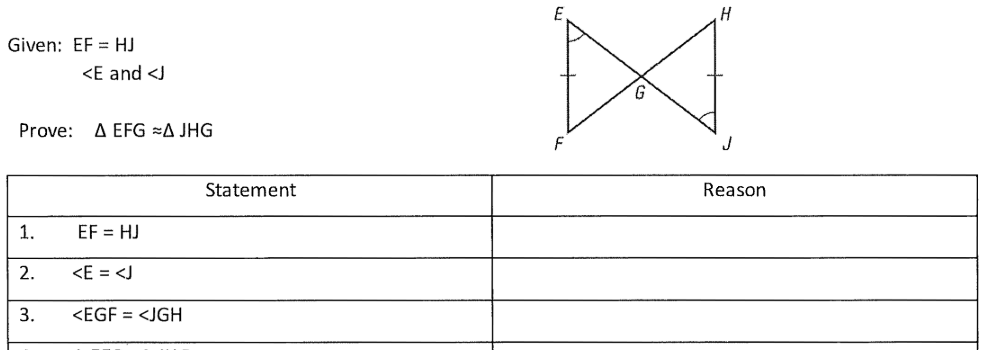
Alternate interior angles
400
What congruence theorem can be used to prove the triangles below are congruent?
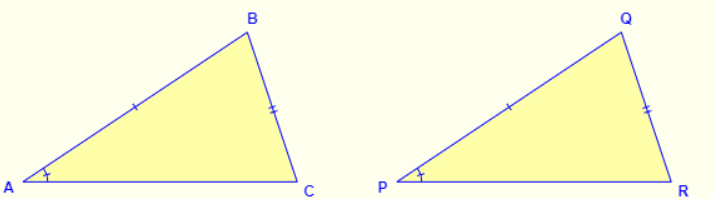
Not congruent.
SSA is not a congruence theorem.
500
Other than "given", name 3 other things you can list as a justification in a geometry proof?
Answers vary.
e.g. midpoint, bisector, alternate interior angles, reflexive property, vertical angles or AAS/SSS/SAS/ASA/HL, etc.
500
IF DB bisects <B, what angle is <DBC congruent to?
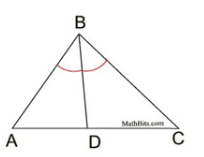
<DBA or <ABD
500
What theorems can you use to prove right triangles are congruent?
ALL of them!!
500
In the triangles below, AD || CE. Name 2 angles that must be congruent.
hint: DC and AE are transversals
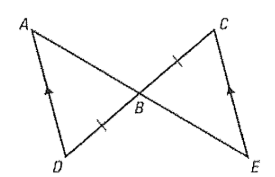
What is <A and <E OR <D and <C?
Alternate interior angles.
500
Name TWO congruence theorems that can be used to prove the triangles below are congruent.
What are SAS, AAS?