Diagonals
Interior and Exterior Angles
Changing Perimeter and Area
Area of Composite Polygons
100
A square has a diagonal of 10 cm. Find the length of its other diagonal.
10 cm
100
A convex polygon has 12 sides. Find the sum of its exterior angles.
360º
100
Find the perimeter of a triangle that is twice the size of the one below.
(7cm+5cm+6cm)xx2=36cm
100
The area of the whole figure is 100 sq. mi. The area of the rectangle is 50 sq. mi, and the area of the square is 25 sq. mi. Find the area of the triangle.
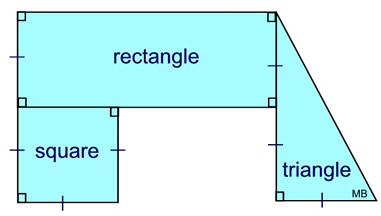
A_T=25mi^2
200
ABCD is a rhombus.
m/_ABC = 62º.
"Find " m/_ACB.
m/_ACB = 59º
200
"The sum of the interior angles of a polygon is "bb "sum" = (n-2)180º
Find the sum of the interior angles of a pentagon.
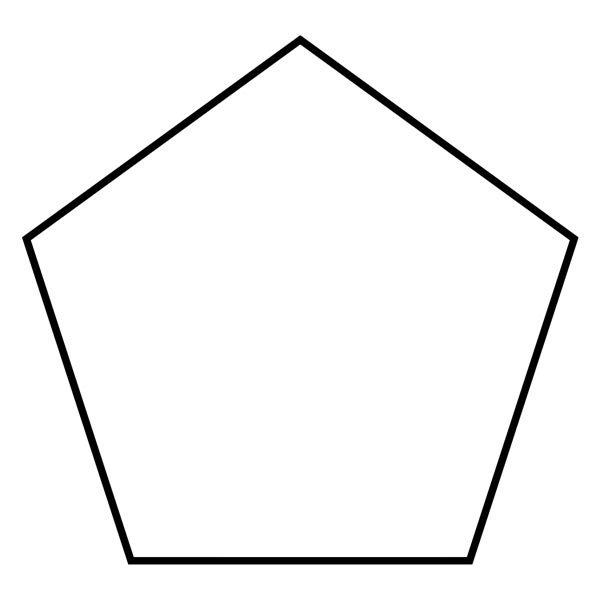
bb "sum" = (n-2)180º = (5-2)180º = 540º
200
A_("Parallelogram")=bxxh
James is building a patio in his backyard in the shape of a parallelogram.
James needs to know the area of his patio so that he knows how much paint to purchase. If each can of paint covers 25 sq. ft, how many cans of paint must James buy?
bb "Area"=17ftxx24ft=408ft^2
frac(408ft^2)(25ft^2)=16.32
"James must buy at least 17 cans of paint to cover his patio."
200
Find the area of the figure:
A_("Parallelogram")=bxxh
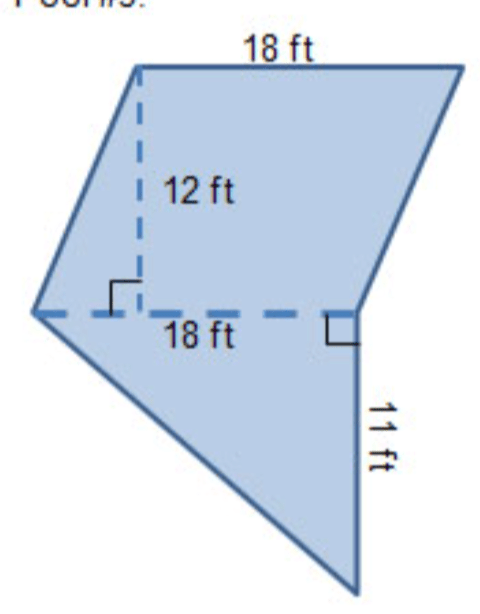
A_P = 12ftxx18ft =216ft^2
A_T = frac(18ftxx11ft)(2)=99ft^2
A = A_P+A_T=216ft^2+99ft^2 = 315ft^2
300
ABCD is a parallelogram.
"If " VT=16 and SU=12, "find ET."
ET = 8
300
The sum of the exterior angles of a regular polygon is 360º.
Find the measure of each exterior angle of the regular hexagon below.
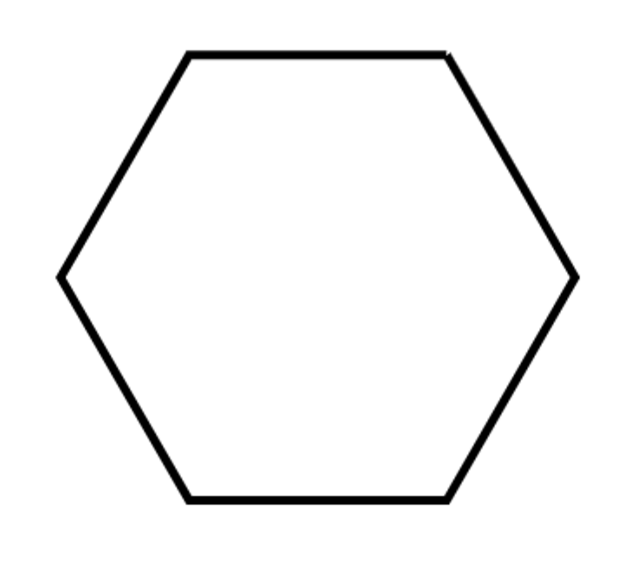
frac(360º)(6)=60º
300
If the length of the longer diagonal of the kite is reduced by 5, what is the area of the kite?
A_("Kite")=frac(pxxq)(2) " where p and diagonals"
11+4-5=10
A_("Kite")=frac(pxxq)(2)=frac(10xx7)(2)=35units^2
300
A_("Trapezoid")=frac(b_1+b_2)(2)xxh
Find the area of the figure.
A_T=frac(13"in"+24"in")(2)xx4"in" = 74"in"^2
A_R=7"in"xx24"in"=168"in"^2
A = 74"in"^2 + 168"in"^2 = 242"in"^2
400
James is building a rectangular patio in his backyard. He measures the length of the patio to be 12 feet and the width to be 9 feet. Answer the following question about this patio. Use the proper units of measure.
James wants to check if the patio is perfectly rectangular by measuring its diagonal. What is the length of the diagonal?
(Hint: Use the Pythagorean Theorem.)
The diagonal is 15 feet.
400
"The sum of the interior angles of a regular polygon is " bb "sum"=(n-2)180º
Find the measures of each interior angle of a regular octagon.
"sum"=(n-2)180º=(8-2)180º=1080º
"each"=frac("sum")(n)=frac(1080º)(8)=135º
400
Hexagon P is similar to Hexagon Q. The sides of Hexagon P are four times the length of the corresponding sides of Hexagon Q. If the perimeter of Hexagon P is 200 inches, what is the perimeter of Hexagon Q?
P_Q=frac(200"inches")(4)=50"inches"
400
The area of kite ABCD is 96 sq. meters. If AO = BO = 4 meters, find the area of triangle BCD.
A_(BCD) = A_(Kite) - A_(ABD)=96m^2-((4xx4)/2)xx2m^2=80m^2
500
APTR is a trapezoid.
m/_TPA = 60º
m/_PTD = 85º
m/_TRA = 110º
"Find "m/_RTD.
"Hint: only 2 of the given angle measures are useful."
m/_RTD = 35º
500
"The sum of the exterior angles of a regular polygon is 360º."
"The measure of one exterior angle of a regular polygon is 36°."
"How many sides does the polygon have?"
n=frac(360º)(36)=10
500
"The area of a square is " 196 yards^2.
"The length of two opposite sides of the square are reduced by " 2yards, "each."
"(making the square a rectangle)."
"Find the new area."
196yards^2=s^2
s=14yards
s-2=12yards
"2 sides are 14 yards, and 2 sides are 12 yards."
A=14yardsxx12yards = 168yards^2
500
Find the area of the regular polygon below.
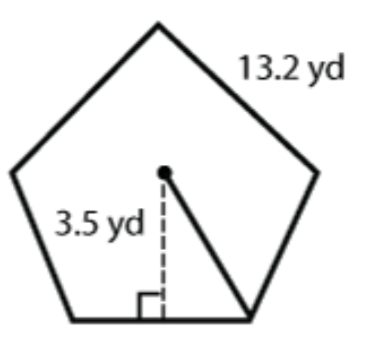
A=(Pa)/2
A=(Pa)/2=(66xx3.5)/2=115.5yd^2