What is the range of a discrete function with the following points: (-1,2), (2, 0), (4, 10), (6, 12)
Range: {0, 2, 10, 12}
Where is the function increasing? Write in interval notation.
(-3,2.5)
Find the relative maximum(s).
3.5
Is the following function even, odd or neither
Odd
Is the following function a 1 to 1 function? If not, why?
No. The output 0, has 2 inputs 0 and -3
Find the range. Write as set-builder notation.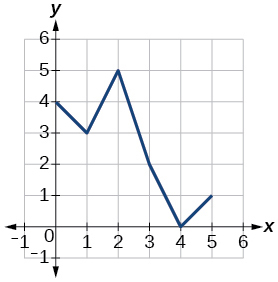
0≤y≤5
Find the interval(s) of constant. Use set-builder.

x<-1
Choose the graph which correctly shows a function and its inverse graphed on the same coordinate plane.
B
Is the function even, odd or neither?

Neither
f(x)
goes through the points (1,2) (2,3) (3,4) and (4,5). What is
f^-1(2)
f^-1(2)=1
Find the domain. Write as interval notation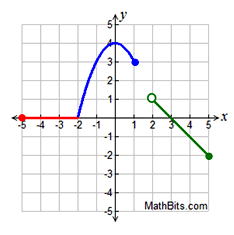
[-5,1]⋃(2,5]
Find the interval(s) of decreasing. Use interval notation.
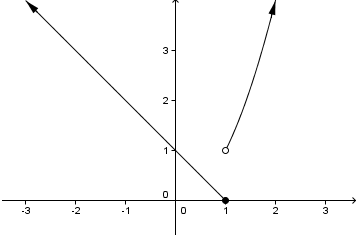
(-∞,1]
Find the x-intercept(s).

-2
Given the function
f(x)
and
g(x)
below, tell whether each function is even odd or neither.
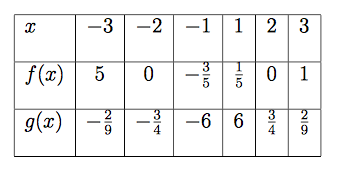
f(x)
Neither.
g(x)
Odd.
Given the mapping diagram below, find the inverse
3 --> 2
6 --> 5
10 --> 4
20 --> 1
Find the range of the function. Write as interval notation.
[-4,3]
What is the positive interval? Write as interval notation.
(-∞,0)⋃(0,∞)
Find a negative interval. Write as set-builder.

-2<x<0
Determine if the function is even, odd or neither.
h(x)=x^3(2x^5-x)+3
h(x)=x^3(2x^5-x)+3
h(x)=2x^8-x^4+3
Even.
Find the inverse
f(x)=-x^2-1
f^-1(x)=sqrt(-x-1)
Find the domain of the function. Write as set-builder.
-4≤x≤4
Find the interval(s) of increase. Use set-builder.
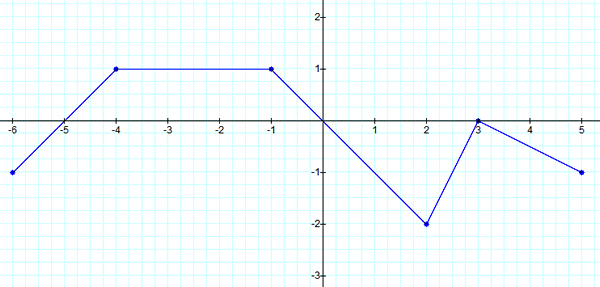
-6≤x<-4 or 3<x≤5
Given the function, find the relative minimum(s).
3 and 0
Determine if the function is even, odd or neither.
(x^2+4)/(x^3-x)
((-x)^2+4)/((-x)^3-(-x))
(x^2+4)/(-x^3+x)
(x^2+4)/(-(x^3-x))
-(x^2+4)/(x^3-x)
Odd.
The function
f(x)
represents the number of books read by Maddie in terms of (dependent on)the months. What are the domain and range for
f^-1(x)
For
f(x)
Domain: # of months
Range: # of books read
So for
f^-1(x)
Domain: # of books read
Range: # of months

