The standard form of quadratics
y=ax^2+bx+c
The vertex form of quadratics
y=a(x-h)^2+k
The factored form of quadratics
y=a(x-p)(x-q)
Rewrite the following in standard form:
(x-4)(x+5)=0
x^2+x-20=0
What is the vertex ?
(3,-1)
The value of y -intercept of quadratics in standard form
y=c
How does the value of 'h' transform the graph?
The graph shifts left or right
The factored form of
x^2-6x+8
(x-2)(x-4)
What is the vertex of the equation
y= (x-12)^2+7 ?
(12, 7)
Quadratic equations take the shape of a what letter when graphed.
u
The y value of y = x^2 + 2x + 1 at
x=-4
y=9
What is the imaginary straight line called that divides a parabola into two equal parts? (x=h)
Axis of Symmetry
Find the vertex of:
3(x+4)(x-2)=0
(-1, -27)
When x2 is changed to x2 -3, how does the graph shift?
Shifts down 3 units.
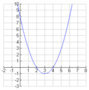
How many solutions does this graph have?
No solution
The following is a perfect square trinomial. How can you rewrite this in factored form?
y=a^2 + 2ab + b^2
y=(a+b)^2
The vertex of
-2 (x + 4)^2 + 2
(-4,2)
What is the imaginary straight line called that divides a parabola into two equal parts? (x=h)
Axis of symmetry
A parabola has a vertex at (-3,2). Where is the axis of symmetry?
x = -3
Name the zero(s)
0 and 4
Rewrite the following in factored form using the difference of squares.
y=a^2-b^2
y=(a-b)(a+b)
The y -intercept of
y = 2(x+3)^2 - 8
(0,10)
Find the x intercepts of:
(x-3)(x+5)=0
(3,0) and (-5,0)
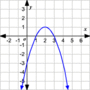
Does the graph of
-2(x + 5)^2 + 2
have a minimum or a maximum?
Maximum
What are the solution(s)?
1 and 3