Rules of Exponents
Word Problem
Graphs
Solve
Random
100
An equivalent expression to x3/5 is:
Rewrite
(root5(x))^3
root5(x^3)
100
A group of friends wants to go to the amusement park. They have $284.25 to spend on parking and admission. Parking is $9.25, and tickets cost $27.50 per person, including tax. How many people can go to the amusement park?
27.5x+9.25=284.25
x=10 people
100
Graph
y=3/4x-4
100
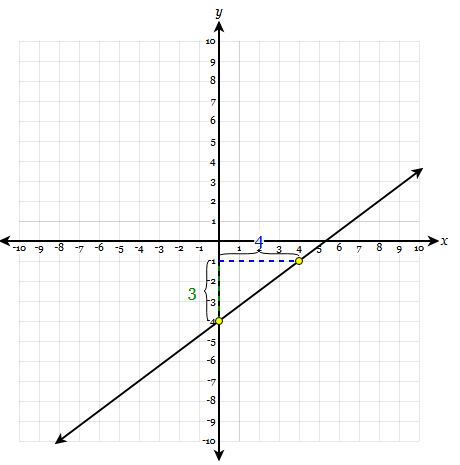
Solve the system
3x+2y=15
y=6x
x=1
y=6
100
Which of the following tables represents a function?
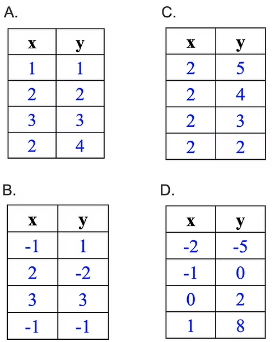
D
200
Rewrite in form, xa
root7(x^3)
x3/7
200
Caleb buys cookies and potatoes at the store. He pays a total of $41.50. He pays a total of $5.18 for the cookies. He buys 8 bags of potatoes that each cost the same amount. How much does each bag of potatoes cost?
8x+5.18=41.50
x=$4.54/bag of potatoes
200
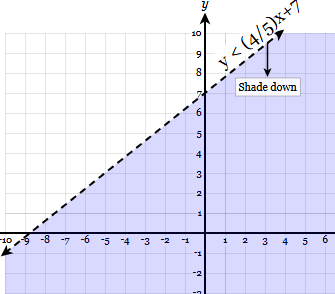
Graph
-4x+5y<35
200
Solve the system
x=4y-6
-4x+3y=-28
x=10
y= 4
200
Find solution(s) of the given graph
(-2, -4) & (0,-2)
300
Write an expression equivalent to
m^12/m^4
in the form, mn
m8
300
The drama club is selling tickets to their play to raise money for the show's expenses. Each student ticket sells for $7 and each adult ticket sells for $9. There was a total of $543 in revenue from the sale of 67 total tickets. Write a system of equations that could be used to determine the number of student tickets sold and the number of adult tickets sold. Define the variables that you use to write the system. Equations Only
s= students
a= adults
7s+9a=543
s+a=67
300
Sarah buys passes for amusement park rides. The total cost C depends on how many passes P she buys. The table below shows the relationship. What is the average cost per ride when Sarah buys 5 passes?
$6
300
Solve system of equation
-x+4y=25
-2x-2y=-10
x=-1
y= 6
300
Describe Transformation
(x,y) to (x+2, -y+1)
Left 2
up 1
Reflected about the x-axis
400
Write an expression equivalent to
(2x^2y^2)(-4x^5y^6)
in the form Axmyn
-8x7y8
400
At a particular restaurant, each mini hotdog has 60 calories and each mozzarella stick has 70 calories. A combination meal with mini hotdogs and mozzarella sticks is shown to have 1080 total calories and 5 more mini hotdogs than mozzarella sticks. Write a system of equations that could be used to determine the number of mini hotdogs in the combination meal and the number of mozzarella sticks in the combination meal. Define the variables that you use to write the system. Equations Only
h = hotdogs m = mozzarella sticks
60 h + 70 m = 1080
h = m + 5
400
Craig is a teen who mows lawns in his neighborhood. He keeps track of how long it takes him to mow each lawn. What is the average time per lawn for the first 4 lawns?
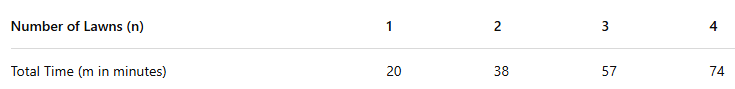
18 minutes per lawn
400
Solve system of equations
8x+6y=28
9x+3y=39
x=5
y=-2
400
Consider the right triangle. Write the correct ratio for Sin (U)=
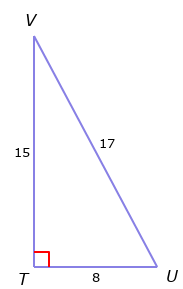
15/17
500
Write an expression that is equivalent to
root3(5y^4) times root3(10y^2)
501/3y2
500
Tallulah and her children went into a movie theater and she bought $73.50 worth of bags of popcorn and candies. Each bag of popcorn costs $6.50 and each candy costs $3.50. She bought a total of 15 bags of popcorn and candies altogether. Determine the number of bags of popcorn and the number of candies that Tallulah bought. Solve for both variables
6.50b+3.50c=73.50
b+c=15
b= 7 popcorn
c= 8 candies
500
A manager wanted to analyze the online shoe sales for his business. He collected data for the number of pairs of shoes sold each hour over a 14-hour time period. He created a graph to model the data. Determine the average rate of change between the first and sixth hours.
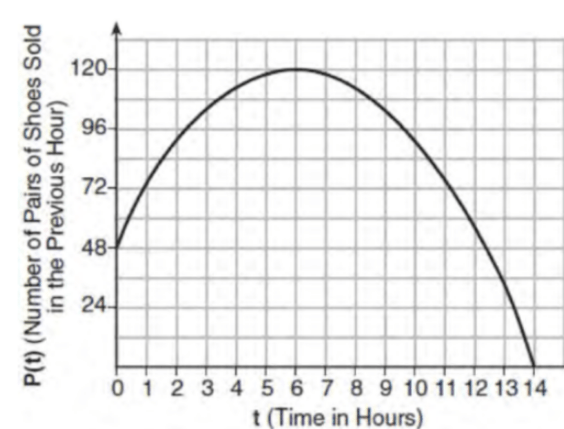
Avg. is 9 shoes per hour
500
Solve system of equations
x-5y=-9
-5x+8y=-6
x = 6
y = 3
500
True or False: The graph below models the height of a remote-control helicopter over 20 seconds during flight. The minimum height of the helicopter is at 20 seconds.
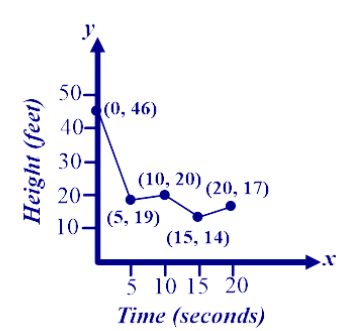
False: the lowest height is at 15 seconds.