Normal Distribution
All About
Z-Scores
Z-Scores
Hypothesis Testing
Is It Significant?
100
Draw a normal distribution.
100
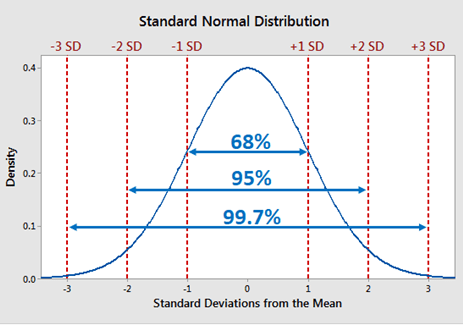
The z-score represents how far away from the ______ a data value lies.
Mean
100
You are flipping a coin to see if it is fair. Complete the following statement about your Null Hypothesis. H0: P(Heads) = ___________
0.5 or 50%
100
The z-score of a data value is -1.86. Is the value significantly HIGH, significantly LOW, NEITHER, or BOTH with 95% confidence?
NEITHER because is is between -2 and +2
200
In a normal distribution, what percent of data values lie with +/- 2 standard deviations of the mean?
95%
200
A z-score calculates the number of ______________ that a value is away from the mean.
Standard deviations
200
We conduct an experiment and get a result that has a z-score of +1.59. Should we REJECT or NOT REJECT the null hypothesis with 95% confidence?
NOT REJECT because the z-score is less than +2
200
Write a z-score for a result that is significantly low with 95% confidence.
Anything <= -2
300
Describe the relationship between the Mean, Median, and Mode of a normal distribution.
They are all equal to each other.
300
If the z-score of a data value is zero, then the value must be _________________
Equal to the mean
300
To reject a null hypothesis with 95% confidence, what range(s) of values could you have for the z-score of your outcome?
Greater than +2 or less than -2.
300
Khloe scored a 60 on her psychology quiz, but the class as a whole averaged a 40. If the standard deviation was 8, is her result significantly HIGH, significantly LOW, NEITHER, or BOTH with 95% confidence?
HIGH. The z-score is 2.5.
400
In a normal distribution, what percent of values lie between the mean and +1 standard deviation?
34%
400
Alicia scored a 70 on her psychology quiz, but the class as a whole averaged a 40. If the standard deviation was 10, what was Alicia’s z-score?
3
400
You run an experiment to see if boys and girls have the same SAT scores. Boys average 1,088 and girls average 1,067. Should you reject your null hypothesis?
NO. You don't have the standard deviation to calculate a z-score.
400
You have a null hypothesis that a coin is fair. You flip it 36 times and flip Heads 10 times. Is this result significant with 95% confidence? SHOW YOUR CALCULATIONS.
Mean = 18. S.D. = 3. z-score = -2.66. Significantly low.
500
TRUE or FALSE: The heights of all people in the world is approximately normally distributed. EXPLAIN YOUR REASONING.
FALSE. The distribution will be left-skewed because of babies and children.
500
You flip a coin 100 times and flip Heads 58 times. What is the z-score for this outcome?
Mean = 50. S.D. = 5. Z-Score = 1.6
500
TRUE or FALSE: We can prove with 100% certainty that a coin is not fair. EXPLAIN YOUR REASONING.
FALSE. We can reject a null hypothesis with 95% (or more) confidence, but never with 100% certainty.
500
Jose ran an experiment and his result has a z-score of +2.68. Is his result significant with 99.7% confidence? EXPLAIN YOUR REASONING
NO. 99.7% means you must be 3 standard deviations above the mean.