All about Systems of Equations
Solving Systems of Linear Equations: Graphing
Solving Systems of Linear Equations: Substitution1
Solve using any of three methods
Applications of Systems
100
What is a the linear equation we use? (slope-intercept form)
y=mx+b
100
14) What is the solution to the system of linear equations?
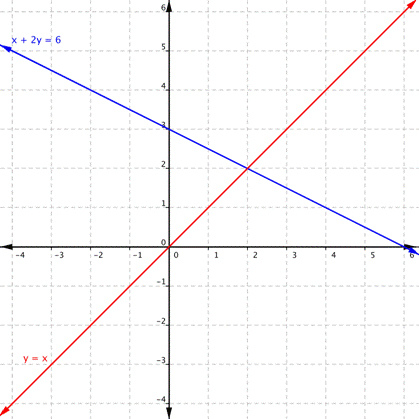
(2,2)
100
If we were to do substitution and have a graph of the same system of equations would we get the same solution?
Yes.
Every equation graphs a specific line so no matter how you solve, you get the same solution!
(Graphs are definitely easier, but we don't always have graphed systems!)
100
Solve the systems of equations using substitution:
y=3x-10
x=2
(2,-4)
100
What is the first step when solving word problems with systems of linear equations?
Write a let statement for each variable.
200
True or False:
It does not matter which method you use when solving systems of linear equations because they will all give you the same answer.
True
200
What is the solution?
(-1,1)
200
What is the first step when solving a system of linear equations using the SUBSTITUTION method?
Isolate the variable
200
Solve the systems of equations using substitution:
y=2x-10
y=4x+8
(-9,-28)
200
Find the value of two numbers if their sum is 12 and their difference is 4
4 and 8
300
What does it mean to "find the solution" to a system of linear equations?
It means to find the point of intersection
300
Solve Using Graphing:
y = 5/3x + 2
y = -3
300
What is your new (one) equation after performing substitution?
y=2x
3x+y=20
3x+(2x)=20 or
5x=20
300
Solve the systems of equations using substitution:
y = -2x - 9
3x -6y = 9
(-3, -3)
300
The school that Stefan goes to is selling tickets to a choral performance. On the first day of ticket sales the school sold 3 senior citizen tickets and 1 child ticket for a total of $38. The school took in $52 on the second day by selling 3 senior citizen tickets and 2 child tickets. Find the price of a senior citizen ticket and the price of a child ticket.
senior citizen ticket: $8, child ticket: $14
400
At least how many linear equations will be in a system of linear equations?
At least 2 linear equations
400
Solve the systems of linear equations by graphing:
400
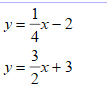
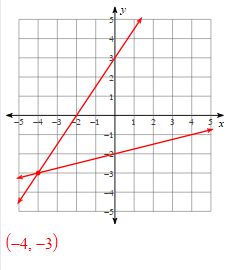
How would you isolate x in this equation?
6y+x=20
subtract 6x from both sides to get:
x=20-6x
400
Solve the systems of equations using substitution:
2x - y = 6
x = y + 5
(1, -4)
400
Mrs. Pastala tells you that the next test is worth 100 points and contains 38 problems. Multiple-choice questions are worth 3 points and word problems are worth 4 points. How many of each type of questions are in there?
(30,8)
8 word problems
30 Multiple Choice
500
How do we write solutions to systems of linear equations?
(x,y)
500
Solve this by graphing:
2x - y = 4
x + 3y = 3
(3,2)
500
Use the substitution method to solve the system of linear equations:
y=2x
3x+y=20
Solution: (4,8)
500
Use the Elimination method to solve the system of linear equations:
−8x − 10y = 24
6x + 5y = 2
(7,-8)
500
Adult tickets for the school musical sold for $3.50 and student tickets sold for $2.50. On a given night, 321 tickets were sold for $937.50. How many of each kind of ticket were sold?
(135, 186)
adults-135
Children- 186