Graphing +
Substitution +
Elimination+
Surprise
Misc (fastest)
100
What is the solution?
(-1,1)
100
Solve the systems of equations using substitution:
-3x + 4y = -2
y = -5
(-6, -5)
100
Solve the systems of equations using Elimination:
14x + 2y = 26
-14x - 6y = -50
(1, 6)
100
What strategy would you use and explain why?
14x + 2y = 26
-14x - 6y = -50
elimination because the coefficients of x will cancel out.
100
Do the Check:Is the given point a solution to the system of equations?
Point: (2,6)
x + y = 8
3x - y = 0
Yes
200
How many solutions are there?
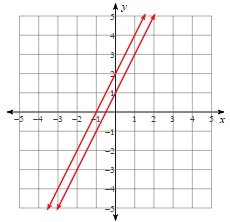
No Solutions
200
Solve the systems of equations using substitution:
-5x - 5y = 10
y = -4x -17
(-5, 3)
200
Solve the systems of equations using Elimination:
-3x - 5y = 2
3x + 5y = 7
No Solution
200
What strategy would you use and explain why?
-5x - 5y = 10
y = -4x -17
substitution because y is already solved for.
200
Do the Check: Is the given point a solution to the system of equations?
Point: (1/2, -2)
6x + 5y = -7
2x - 4y = -8
No
300
Solve Using Graphing:
y = 5/3x + 2
y = -3
300
Solve the systems of equations using substitution:
y = -2x - 9
3x -6y = 9
(-3, -3)
300
Solve the systems of equations using Elimination:
-6x - 10y = 4
6x + 10y = -4
Infinitely Many
300
Is there 1 solution, No solution, or Infinite solutions for the system of linear equations below? AND JUSTIFY WHY
3x - y = 19
-3x + y = 10
No Solutions because it is a false statement.
300
What type of lines have no solutions?
What are Parallel lines.
400
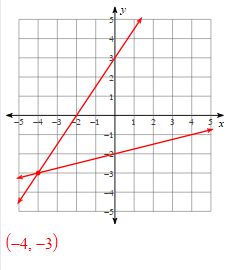
Solve the systems of linear equations by graphing:
4y = x - 8
-3x + 2y = 6
400
Solve the systems of equations using substitution:
-8x + y = -7
16x - 2y = 14
Infinitely Many Solutions
400
Solve the systems of equations using Elimination:
-5x + 7y = -7
-2x - 2y = 2
(0, -1)
400
Name two forms of a linear equation?
slope-intercept
point-slope
standard form
400
a negative added with another negative will give you a _________.
Negative
500
To show a solution when graphing a linear inequality in 2 variables, you do this.
Shade one side of the line. (above or below)
500
A line that has a slope of zero is this type of line.
horizontal line
500
Perpendicular lines form these types of angles.
Right angles or 90 degrees
500
When 2 lines intersect, these 2 special angles are formed.
Vertical angles
500
What should you do after finding a solution?
What is check your answer?