Solve by Graphing
Solve by Substitution
Solve by Elimination
Linear Inequalities
100
What is the solution?
(-1,1)
100
Solve the systems of equations using substitution:
-3x + 4y = -2
y = -5
(-6, -5)
100
Solve the systems of equations using Elimination:
14x + 2y = 26
-14x - 6y = -50
(1, 6)
100
Solid line means....
the solution can be on the line
greater than or equal to, less than or equal to
200
How many solutions are there?
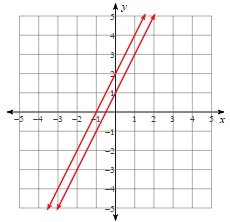
No Solutions
200
Solve the systems of equations using substitution:
-5x - 5y = 10
y = -4x -17
(-5, 3)
200
Solve the systems of equations using Elimination:
-3x - 5y = 2
3x + 5y = 7
No Solution
200
Dashed Line means....
< or >
300
Solve Using Graphing:
y = 5/3x + 2
y = -3
300
Solve the systems of equations using substitution:
y = -2x - 9
3x -6y = 9
(-3, -3)
300
Solve the systems of equations using Elimination:
-6x - 10y = 4
6x + 10y = 0
No Solution
300
Is (1,1) a solution to this inequality?
y< 3/2x + 7
Yes
400
How many solutions are there?
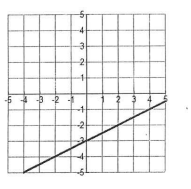
Infinitely Many Solutions
400
Solve the systems of equations using substitution:
-8x - 5y = -24
-x + y = 10
(-2, 8)
400
Solve the systems of equations using Elimination:
-3x - 24y = -66
3x + 4y = -14
(-10, 4)
400
How do you find the solution set to systems of linear inequalities?
where the shaded regions intersect after graphing
500
Solve the systems of linear equations by graphing:
500
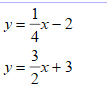
Solve the systems of equations using substitution:
-8x + y = -7
16x - 2y = 14
Infinitely Many Solutions
500
Solve the systems of equations using Elimination:
-15x + 6y = -36
8x - 6y = 22
(2, -1)
500
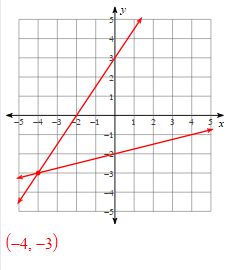
Solve this linear inequality
y< x+1
y> -2x
see boards