Angles and Triangles
Translations
Rotations
Reflections
Identifying Rules
Recognize the transformation
100
Can the following lengths create a triangle? How do you know?
10.4 INCHES, 4.7 INCHES, 12.0 INCHES
Yes, because the two smallest sides added together are larger than the third side.
100
The word that describes translating.
Slide
100
The word that describes rotating
turning
100
This word is another way of saying reflecting
Flip
100
This rule describe translating up 6 units.
(x, y+6)
100
The graph depicts a rigid transformation. What is the rule? Give the words and the notation.
1 unit right 2 units down: (x + 1, y - 2)
200
What's the measurement of the missing angle? 62.5, 35.4
82.1
200
Adding to the x-coordinate moves a figure in this direction.
to the right
200
If both coordinates change signs what transformation occurred?
180 degree rotation
200
This coordinate stays the same when a figure is reflected over the y-axis.
the y-coordinate.
200
Describe this transformation rule: (x, + or - y)
reflect over the x-axis?
200
The given graph depicts a rigid transformation.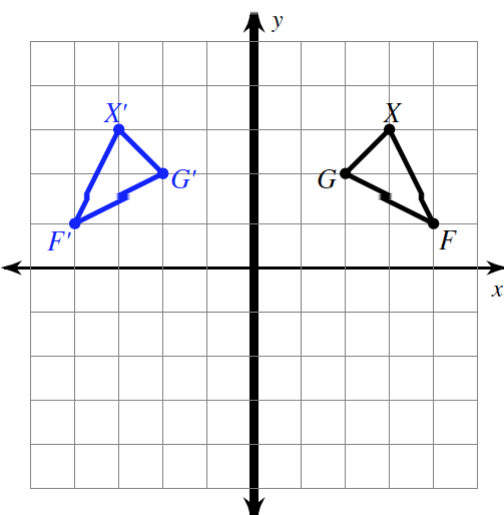
What is a reflection in the y-axis?
300
Find the missing angle below.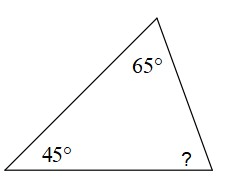
70 degrees
300
If (1, 6) is translated to the left 3 and down 1, what are the new coordinates?
(-2, 5)
300
The segment below has been rotated 90o in what direction?
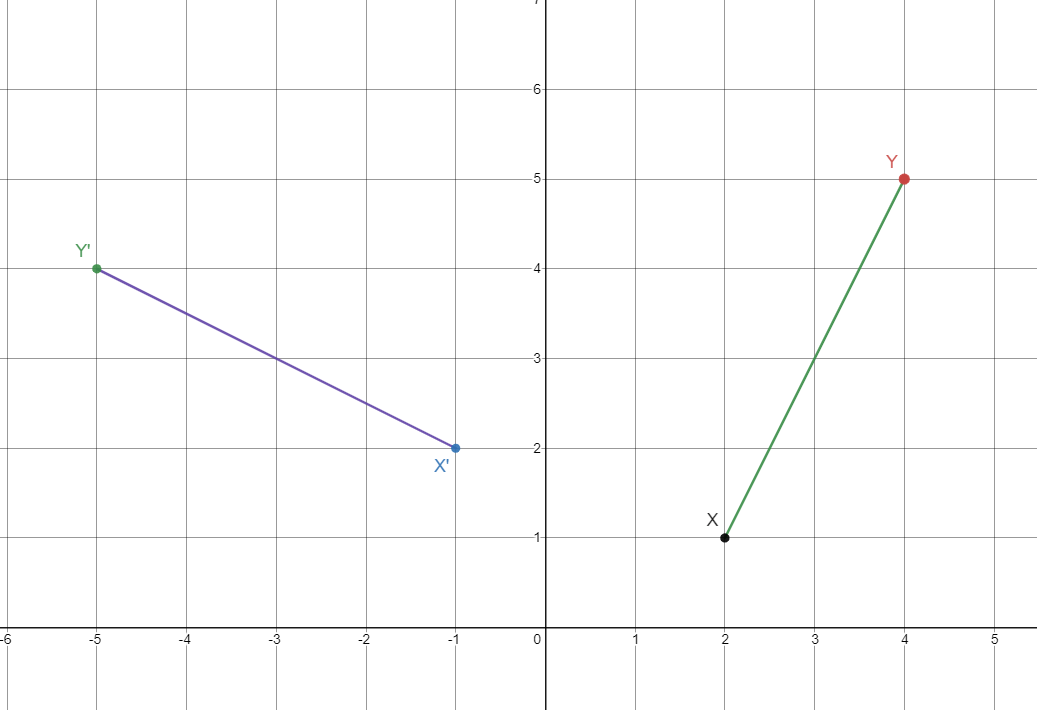
counter clockwise
300
This coordinate changes when a figure is reflected over the x-axis.
the y-coordinate
300
If (1,5) becomes (3,0) what is the transformation rule?
(x + 2, y - 5)
300
The given graph depict multiple transformations of the pre-image *. This image depicts a Reflection of * across the y-axis.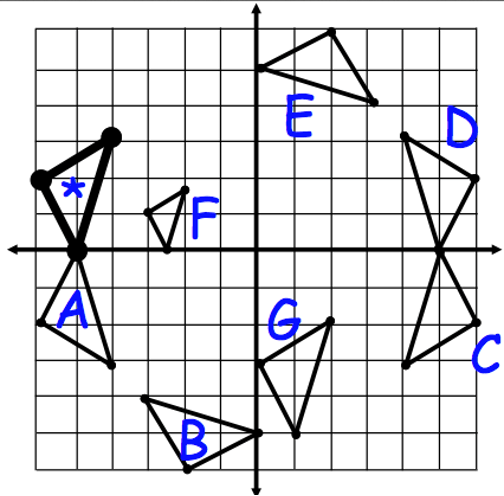
What is D?
400
Are these triangles congruent?
How do you know?
They are congruent, because all of the sides have a corresponding congruent side, therefore only one triangle can be made. All other triangles with the same side measures are congruent.
400
This is what happens to the coordinates if a figure translates up and to the left.
subtract from the x-coordinate and add to the y-coordinate?
400
This is the coordinate M' after the figure below is rotated 180o clockwise.
(-6, -6)?
400
The figure has been reflected over this line.
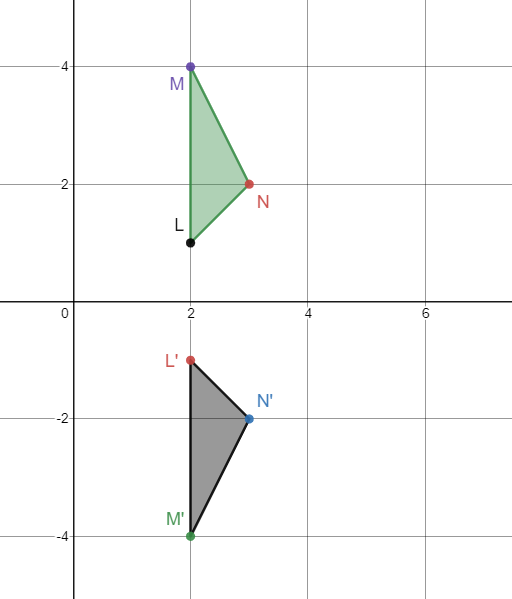
the x-axis
400
This notation (symbol) is how we indicate the figure from the image.
Prime Mark A"
400
The given graph depicts a rigid transformation.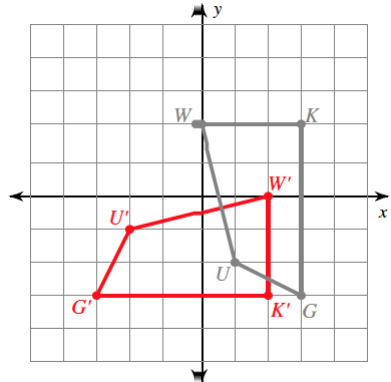
What is a 90-degree clockwise rotaition?
500
Can the following lengths create a triangle? How do you know?
8.4 INCHES, 2.2 INCHES, 12.0 INCHES
No, because the two shortest sides added together are less than the third side. They will be too small.
500
In words, what is the translation rule for the figure to image below?
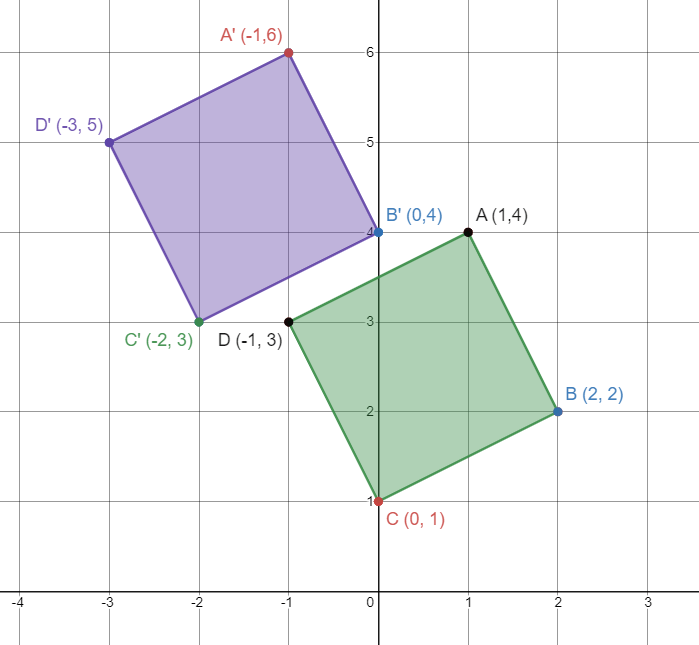
left two units, up two units
500
This clockwise rotation is the same as rotating 90o counterclockwise.
270o clockwise
500
Reflect the triangle ABC over Y-axis with side lengths AB =3 units, BC = 4 units and AC = 5 Units. After reflection What is the length of BC?
4 Units.
500
Name the four types of Transformations.
Translation, reflections, rotations, dilations
500
The given graph shows rigid transformations of the pre-image . Triangle * will have a Rotations of 90-degrees counterclockwise about the origin. Where will the new figure be?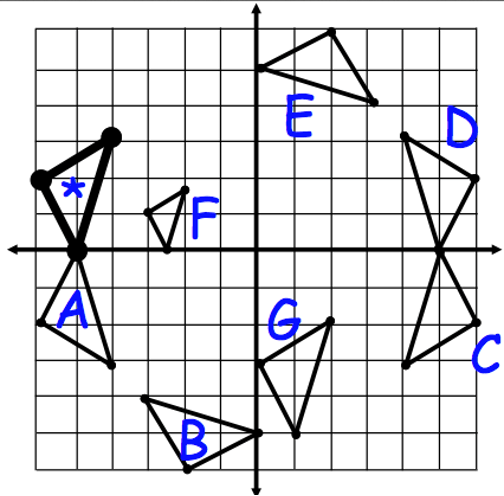 
What is figure B?