f(x)=a(1/(x-h))+k
How does 'k' affect your graph?
Vertical Shift
What is the acronym to help solve this 1/(2x)-:3/2
K.C.F (Keep Change Flip)
When adding and subtracting fractions, what has to be the same between the two fractions?
It has to have the same denominator.
What is the first thing you need to find when solving rational equations?
LCD
What are Holes/Removable Discontinuity in the following example:
(x+2)/((x+2)(x-5))
Holes are factors that you cancel out, and visually, there will be a hole in the graph.
Hole x=-2
f(x)=a(1/(x-h))+k
How does 'h' affect your your graph?
It controls the horizontal shift.
What happens when you have 1/0 ?
Undefined
If given two fractions without a common denominator, what do we have to find between the two denominators?
Ex: 1/(3x)+1/6
LCD (Lowest Common Denominator)
Once you find the LCD, what do you do with it?
Multiply it to each fraction in the numerator
Find the remainder of (2x^3-5x^2-x+3)-:(x+3)
-93
f(x)=a(1/(x-h))+k
How does 'a' affect your graph?
It controls whether the graph compresses or stretches.
What are the excluded values/ "restrictions of x" of the following 1/((x+3)(x-5))
x!=-3,5
What would be the LCD here:
1/(4x)+3/(8)
LCD:8x
What is the LCD here:
1/(2x)+5/8=14/8
LCD: 8x
Find the remainder of the following (5x^3+x^2-7)-:(x+1)
-11
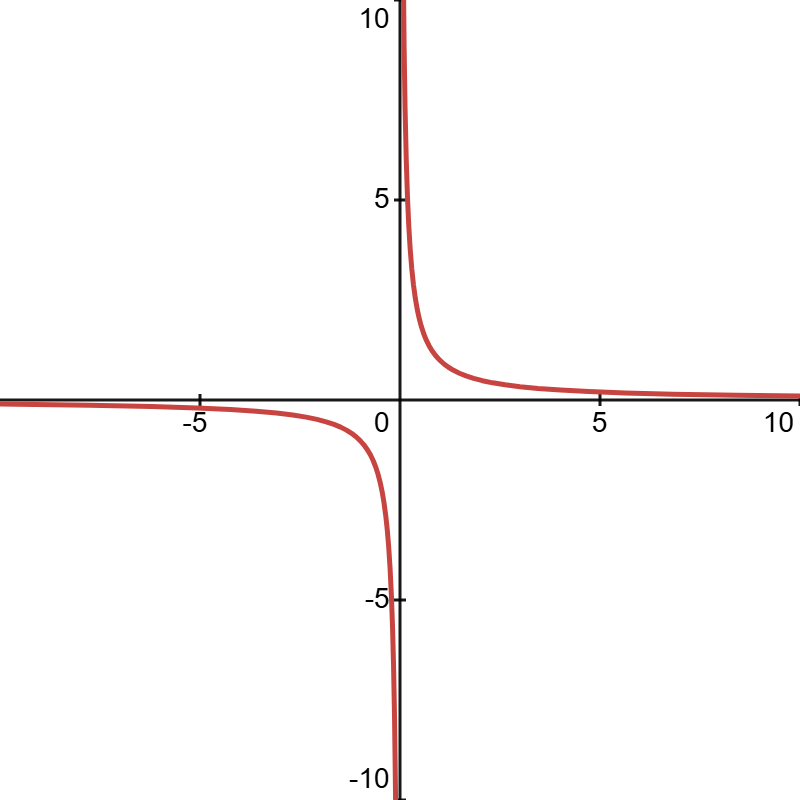
Whether on your notebook or drawing on your table with a marker, provide a rough sketch of what f(x)=1/x looks like.
Multiply the following and find the restrictions of x:
((x-2)/(x+5))((x+5)/4)
(x-2)/(4)
x!=-5
Simplify and find the restrictions of "x"
(x+3)/(x-5)+(7)/1
x!=5
(8(x-4))/(x-5)
Solve for x in the following rational equation:
1/(4x)-2/3=6/(2x)
x=-33/8
How do you find Horizontal and Vertical Asymptotes?
Example 1/(x-4)
H.A. if the degree of the denominator is bigger then y=0.
V.A. you set the denominator equal to zero and solve for x=4
Given: f(x)=1/(x-2)
What transformation occurred compared to the parent graph f(x)=1/x ?
There is a horizontal shift, two units to the right.
Find the restrictions of "x" and multiply
((x+2)/(x-1))(2+(x^2-9)/(x+3))
x+2
x!=1,-3
Simplify and find the Excluded Values (restricted x values)
1/(x-4)-(x+2)/(x^2-16)
x!=4,-4
2/((x+4)(x-4))
Solve for x for the rational equation.
7/(x^2-5x-24)+6/(x+3)=5/(x-8)
x=56
Emma and Jake are setting up for a concert event. If Emma works alone, she can set up all the sound equipment in 15 minutes. Jake, who has some experience with the equipment as well, could do the same job in 30 minutes on his own. If they work together to set up, how long will it take them to finish?
x=10