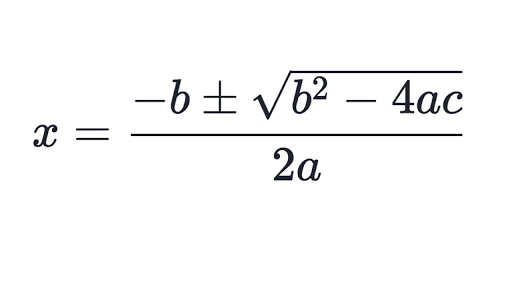What does i2 equals to?
-1
What is the Discriminant formula?
(b)2-4(a)(c)
What is the Quadratic Formula?
Determine if (x-3) is a factor of x3-7x2+16x-13. If not what is the remainder?
No the remainder is -1
Fill in the Blanks.
When we divide two polynomials and the remainder is_____ then the divisor is a _______ of the dividend.
Zero and Factor
√-121
11i
What is the Discriminant, the number and type of solutions?
5x2+8x=-1+2x
Discriminant-16
Number & Type-2 Real
Is this in Standard form? If not rewrite it in the correct form.
4x2-42=-5x
4x2+5x-42=0
Determine if (x-5) is a factor of 5x3-24x2-10x+25. If not what is the remainder? If so what is the solution?
Yes, solution is x=5
Determine if the following divisors are factors using synthetic division. List the Remainder, if its a factor and the solution is.
(3x3+11x2-21x) ÷ (x+5)
Remainder: 5
Is (x+5) a factor: No
What is the solution: N/A
6i√-49
-42
How many types of solutions will I have with this number -8?
2 complex
Solve the problem using the Quadratic Formula to find the solutions/Zeros.
3x2+6x+3=0
x=-1
Find "All" the factors & solutions of
(x3-x2-10x-8) ÷(x+2)
(x+2), (x+1), (x-4)
Use the discriminant formula to find the discriminant, number of, and type of solutions.
-9v2-6v-10=-9
Discriminant: 0
Number & Type: 1 Real
(5+4i) + (3-2i)
8+2i
What is the Discriminant, the number and type of solutions?
3x2+12x+4=-6x2
Discriminant-0
Number & Type-1 Real
Solve the problem using the Quadratic Formula to find the solutions/Zeros.
4x2+5=-12x
X=-1/2
X=-5/2
Divide using Synthetic Division and write the new standard form.
(x3-12x2+17x+30) ÷ (x-10)
x2-2x-3
Use the quadratic formula to find the solutions.
-6p2=-9p-135
x=5
x=-9/2
Must show work!
(1+4i)(3-6i)
27+6i
What is the Discriminant, the number and type of solutions?
2x2+4x+2=x2-3
Discriminant- -4
Number & Type- 2 complex
Solve the problem using the Quadratic Formula to find the solutions/Zeros.
-2x2+17=-4x2
X=1+√67i/4
X=1-√67i/4
Use Synthetic Division to find all the factors and all the roots.
x3-12x2+41x-30 Given roots x=5
All Roots: x=5, x=1, x=6
All Factors: (x-5), (x-1), (x-6)
Must show work!
(3+2i)2
5+12i