To "flip" a figure over a line
Reflection
True or False? Dilations always make a figure smaller.
False
Is a dilation of 7/5 an enlargement or reduction?
Enlargement (Gets bigger)
Give the dimensions of another rectangle that is similar to these two rectangles.
When is our Unit 3 test over Dilations and Sequence Transformations
January 10th
To "slide" a figure.
Translation
A dilation might change the size of a figure's side lengths but never the _______.
angles or shape
What transformations can be used to prove similarity.
List them all!
1) Translations
2) Rotations
3) Reflections
4) Dilations
Point A has the coordinates (4, -3). What is the scale factor used if the new coordinates are (2, - 3/2)?
Scale factor = 1/2 or 0.5
Complete the sentence. Corresponding sides of similar figures are ______________________
Proportional
To "turn" a figure clockwise or counter clockwise
Rotation
Dilations always produce ___________ figures.
similar
If the scale factor is greater than 1 (ex:2) then the dilated image is ______________ from the original figure.
Enlarged
Congruent figures are _____ similar.
(sometimes, always)
always
Similar or not similar?
Not similar - angles are not the same.
When we describe a translation we need to be sure to say:
1) Direction
2) Distance
Dilations need these two things.
1) Center Point of Dilation
2) Scale Factor
If the scale factor is less than 1 (ex:1/4) then the dilated image is ____________ from the original figure.
Reduced
Similar figures are ____ congruent.
(sometimes, always)
sometimes
Find the scale factor. Figure EFGH is the original.
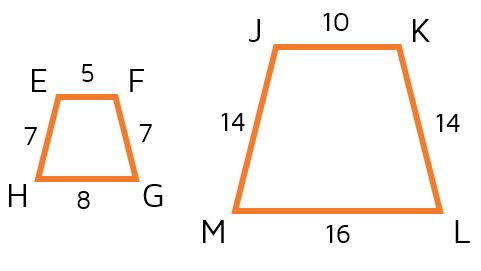
Scale factor = 2
Describing a rotation requires these three things.
1) Center (Point)
2) Rotation angle (Degrees)
3) Direction (Clockwise or Counterclockwise)
If a square is dilated using a scale factor of 2, how many times larger will the area of the new square be?
4 times larger
Dilations can result in a congruent figure if their scale factor is this number.
1
These two triangles are similar. Find the missing side lengths.
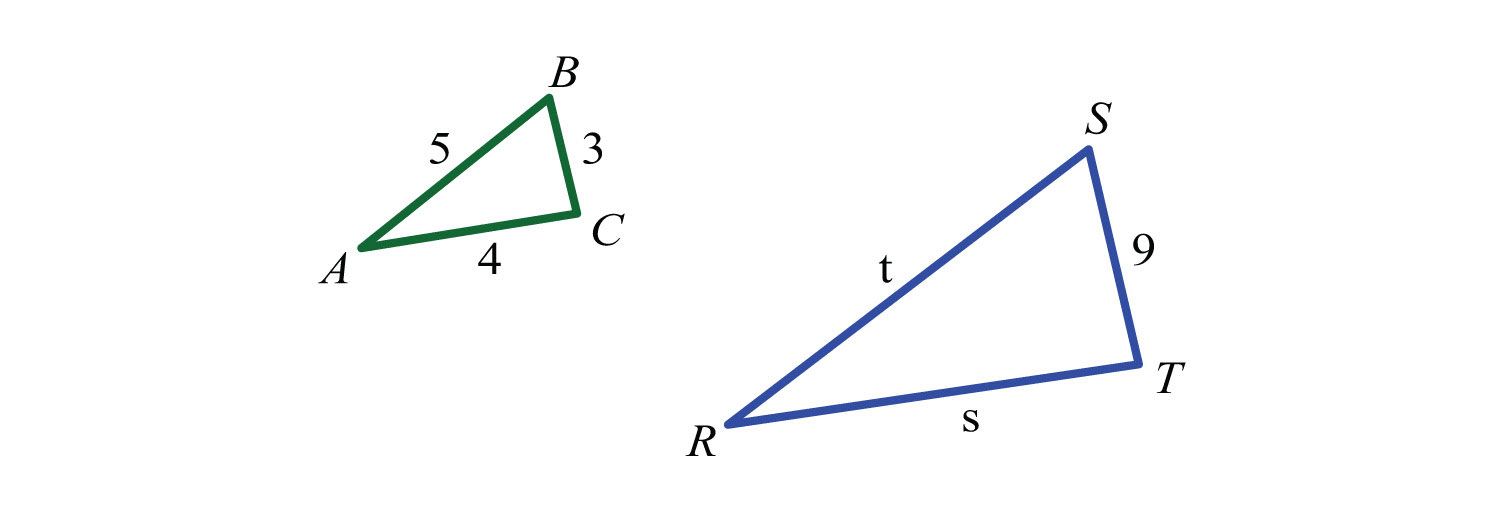
s=12
t=15
A point has the coordinates (8, -2). What are the new coordinates after a dilation using a scale factor is 1/4 and the origin as the center of dilation?
(2, -0.5)

