1.4: Evaluate Square Roots and Cube Roots
1.5: Solve Equations using Square Roots and Cube Roots
1.6: Use Properties of Integer Exponents
1.7: More Properties of Integer Exponents
Mr. C Trivia
100
What could be redescribed as repeated division?
Roots, of any kind
100
When comparing squares and cubes, what powers should I be taking to one of the sides respectively?
(e.g. My side = 5 in., for a cube or a square what powers would I be taking this number to?)
2 and 3 respectively
100
Rewrite using a Power of Products Property:
7^2 * 7^8
7^10
100
What will always be the answer for the Zero Exponent Property?
1
100
What is Mr. C's exact degree?
Engineering Education
200
What is the cubed root of 8?
2
200
Solve:
x^3 = 343
x = 7
200
When looking to use the properties of powers, we are specifically looking at two different things about the expression in question. Name one.
The Base or the Exponent
200
Write the following as a simplified positive exponent:
4^3/4^5
1/4^2
200
What does Mr. C call the mints he hands out?
Advance-mints
300
Emily wants to buy a tablecloth to cover a square card table. She knows the tabletop has an area of 9 square feet. What are the minimum dimensions of the tablecloth Emily needs?
(I want both dimensions with their units)
3 ft. by 3 ft.
300
Solve:
x^2 = 225
x = 15 and -15
300
Rewrite using a Power of Products Property:
3^9*2^9
(3*9)^9
300
Write the following as a simplified positive exponent:
7x^-3
7/x^3
300
What does Mr. C's Wife's Degree?
Chemistry
400
Diego says that if you cube the number 4 and then take the cube root of the result, you end up with 8. Is Diego right?
(be prepared to verbally explain why or why not)
No, 4 cubed is 64, and the cubed root of 64 is 4.
Bonus: He square rooted 64 instead.
400
Solve exactly:
x^3 = 12
∛12
400
Is the expression
3^2/3^3
equivalent to
3^3/3^2
Explain.
The first one is equal to 1/3, while the second is equal to 3
400
Write the following as a simplified positive exponent:
1/2^-6
2^6
400
Mr. C has siblings, where is he in the lineup?
The Oldest
500
A toy has various shaped objects that a child can push through matching holes. The area of the square hole is 8 cm squared. The volume of a cub-shaped block is 64 cm cubed. Will this block fit into the square hole? Explain.
No, the sides of the cube would be 4 cm by 4cm, creating an area of 16 cm squared, too large to fit into the square hole in question.
500
What does the power have to do with the number of possible answers to a root problem?
Even powers: 2 answers
Odd powers: 1 answer
500
What is the width of the rectangle written as an exponential expression?
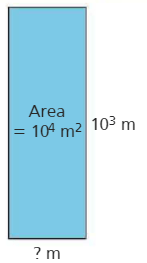
w = 10^1
500
Solve if x = 6
12x^0(x^-4)
1/108
500
How many siblings does Mr. C have?
3, two brothers and 1 sister