Simplify the Expression:
6^2a^0b^3
36b^3
Find the indicated real nth root(s) of a:
n=2, a=121
11 AND -11
Evaluate the Function for the Given Value of X:
y=3(4)^x, x=4
768
Identify the percent rate of growth/decay in the following:
y=10(1+0.03)^t
3%
Evaluate the Expression:
(-3^-2)/(-9)^-2
-9
Evaluate the Expression:
root(3)(216)
6
Determine whether the table represents a linear or exponential function, and explain why.
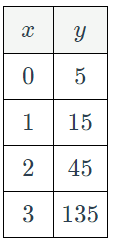
The table represents an exponential function, because its growth factor is 3 (multiplied by 3). The rate of change is not constant.
Identify the percent rate of growth/decay in the following:
y=5(0.85)^t
15%
Simplify the Expression:
(6x^4*x^7)/(2x^3)
3x^8
Evaluate the expression:
root(5)(-243)
-3
Describe the following function as positive/negative and growth/decay.
y=2(1/4)^x
Positive Decay
In 1990, there were 6 wild cats in BH. The population increased by a rate of 14% each year. Write an equation to represent the population after t years.
y=6(1.14)^t
(-2y)^6
64y^6
Evaluate the Expression:
64^(3/2)
512
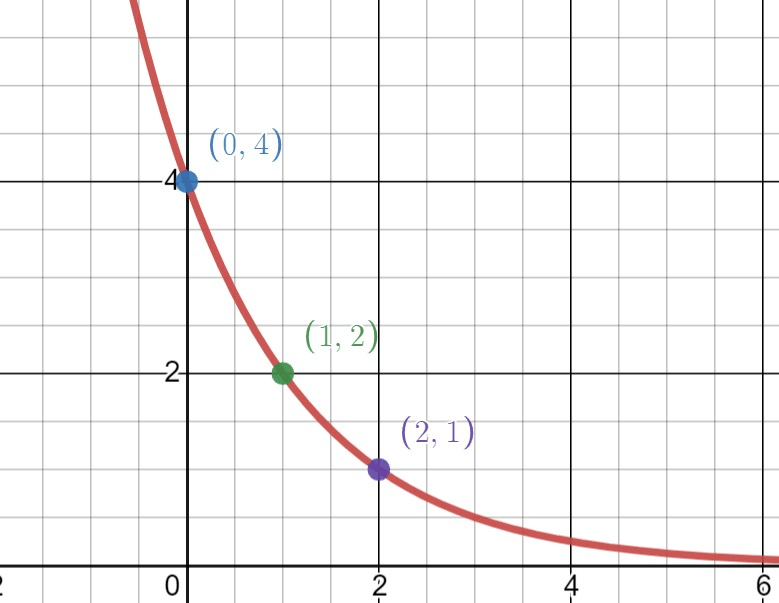
Write an exponential function based on the graph.

y=2^x
The value of Mr. Strager's Lambo at purchase was $208,000, and decreases at a rate of 35% per year. How much will it be worth after 7 years?
y=208000(.65)^t
$10,196.63
Simplify the Expression:
((4x^3y^-2)/(y^-7z^12))^2
(16x^6y^12)/z^24
Evaluate the Expression:
(-125)^(4/3)
625
Graph the function on a separate sheet of paper.
y=4(1/2)^x
I deposit $5000 that earns 7.2% annual interest compounded monthly. Write an equation and figure out how much money will be in the account after 3 years.
y=5000(1+.072/12)^(12t)
$6201.51