Key Features Expon. f(x)'s
Growth or Decay
Growth or Decay 2
Simplify radicals
exponent properties
100
If, y=a(bx ), what do a and b represent?
a = initial amount/y-intercept
b = growth/decay factor
100
How do I know if an exponential function is exponential decay from an equation?
If the b is less than 1.
100
A bunny population doubles every 6 months. If the starting population is 10, how many will you have after 3 years? What is the initial population? What is the growth factor?
after 3 years = 640
initial population = 10
growth rate = 2
100
Simplify the following
√64x2y3
8xy√y
100
solve for x
(6x/2)(6x/3)
x = 36/5
200
What is the initial value and growth/decay factor for the function f(x) = 2(3)x
**Make sure to identify whether it is growing or decaying**
initial value = 2
GROWTH FACTOR = 3
200
What growth RATE does this equation represent?
y = 3(1.5)x
50%
200
In exponential functions, when b>1 this will cause an exponential growth or decay?
Exponential growth
200
Simplify the following
√216x
6√6x
200
solve for x
43x = 8x+1
x = 1
300
What is the equation for this exponential function.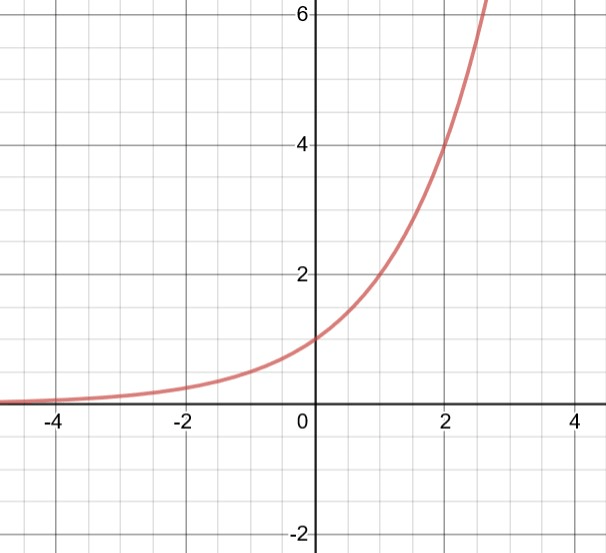
y = 1(2)x
300
f(x)=a(.93)x
Does this functions represent exponential growth or decay? What is the percent growth/decay RATE?
Exponential Decay by 7%
300
f(x)=a(1.07)x
Does this functions represent exponential growth or decay? What's the percent growth/decay rate?
Exponential Growth. 7%.
300
Simplify the following
3√75x2y
15x√3y
300
simplify
a3*a4
a7
400
What is the equation for the function?
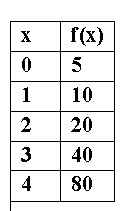
y = 5(2)x
400
What growth FACTOR does the following equation represent?
y = 4(3)x
300%
400
Does the graph represent exponential growth or decay?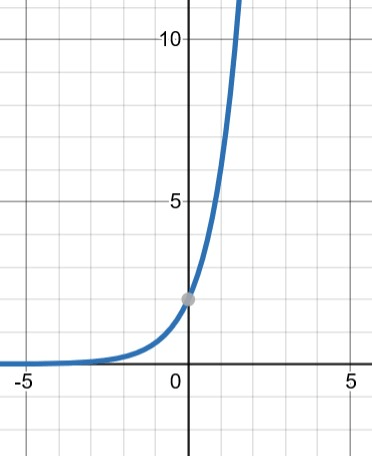
Exponential Growth
400
Simplify the following
4x√28x3y3
8x2y√7xy
400
solve for x
364x - 1 = 6x + 2
x = 4/7
500
What is the difference between linear functions and exponential functions?
Linear has a constant rate of change (slope).
Exponential functions increase or decrease at an increasing rate (exponentially).
500
Ms. Wiggins purchased a car for 26,400 and every year it decays by 12%. What can she expect the value of her car to be after 3.5 years?
f(x) = 26400(.88)3.5 = $16,876.92
500
Annual sales of a fast food restaurant are $530,000 and increasing at a rate of 5%. What will the annual sales be in 6 years?
530,000(1.05)6 =$710,250.69
500
Simplify the following
-8√24x5y3
-16x2y√6xy
500
(1/64)x - 3 = (1/16)2x - 1
x = -7