The vertex of a parabola is (-3, 7).
What is the axis of symmetry?
x= -3
What is more like the a value of this graph?
a. -1
b. 3
c. -3
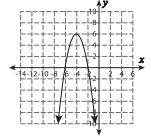
C(-3)
G(x) = (x-1)2 -5
F(x) is the parent function of g(x). Describe the transformation done in G(x)
Move to the right 1 unit and move down 5 units.
An apple is launched directly upward at 64 ft/sec from a balcony standing 80 feet off the ground. Write a quadratic function that shows the height of the apple in terms of time.
Hint: This is a projectile motion problem.
h = -16t2 +64t + 80.
What direction will a parabola with an a value of -2 open?
The parabola will open down.
Find the y-intercept, AOS, vertex, and max or min of the following function.
f(x) = -3x2 +24x -41
y-intercept = -41
AOS = x=4
max
vertex: (4,7)
The transformation done to the quadratic parent function is listed below. Write a equation in vertex form showing this transformation.
Shift 4 units up, and 6 units to the left.
G(x) = (x+6)2 +4
A pineapple is kicked from the ground. The equation -16t2 +90t shows the height of the pineapple after t seconds. Find the maximum height the pineapple reached.
126.56 feet.
Will the parabola with an a value of -3 have a vertex with a max or min?
Max
Compare f(x) and g(x) Which minimum value is greater?
g(x) = 6x2-12x-13

f(x) = (1,-20)
g(x) = (3,-4)
so, g(x) has a greater minimum value. (-4 is greater than -20)
The transformation done to the quadratic parent function is listed below. Write a equation in vertex form showing this transformation.
Reflecting over the x-axis, shift 2 units down, and 8 units to the left
G(x)= -(x+8)2 -2
A pineapple is kicked from the ground. The equation -16t2 +90t shows the height of the pineapple after t seconds. Find the time it took for the pineapple to reach the ground.
5.625 seconds.
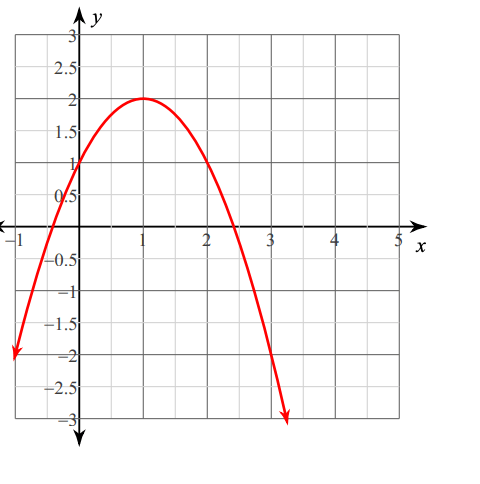
Look at the graph below: Identify the increasing and decreasing intervals.
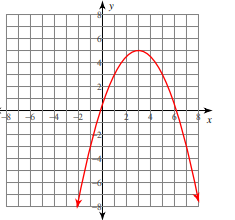
Increasing (-∞, 3)
Decreasing (3, ∞)
Determine the Rate of Change for the following interval [2,6]
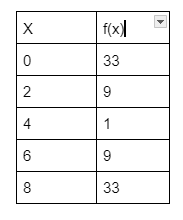
m = 0
The transformation done to the quadratic parent function is listed below. Write a equation in vertex form showing this transformation.
Vertically stretched by a factor of 6, shift 4 units up, and 4 units to the right.
G(x) = 6(x-4)2 +4
A pebble is dropped off a building, which is 515 feet tall. If the pebble’s pathway can be modeled by the equation h=-16t2+515, how long would it take the pebble to strike a 6 foot person?
5.64 seconds
Identify:
a. zeros
b. AOS
c. Max or min
d. Vertex
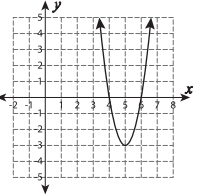
Zeros= (4, 0), (6,0)
AOS= x=5
Min
vertex: (5,-3)
Graph the following:
y=-x2 +2x +1
The transformation done to the quadratic parent function is listed below. Write a equation in standard form showing this transformation.
Vertically stretched by a factor of 3, shift 4 units up, 3 units to the right, and reflected over the x-axis.
A pear is launched from a platform that is 100 meters high. The height of the pear in meters can represented by h(x)= -5x2 +20x +100 where x represents time in seconds. How long would it take for the pear to reach a height of 75 feet?
5 seconds.