Rate of change
Polynomials
Rational Functions
Transformations
Misc.
100
Find the interval where f(x) is concave up. Justify your answer.
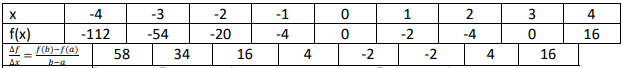
f(x) is concave up on (1, 4) because the average rates of change are increasing.
100
What is the degree of the following function?
6x^7+3x^8-2x^2
8
100
What is the end behavior of the following function?
(4x^3+2x^2-7)/(5x^3+6)
100
Let
f(x)=x^2+4x+1.
Write an equation of the following functions that are transformations of f(x).
g(x)=3f(x)-7
3x^2+12x-4
100
On what open intervals is k(x) both increasing and concave down?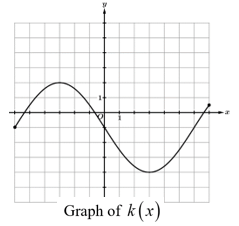 
(-6,-3)
200
Where does f(x) have an inflection point? Justify your answer.
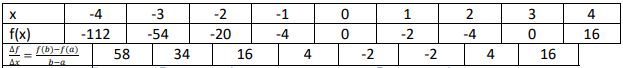
f(x) has an inflection point at x = 1 because the average rates of change change from decreasing to increasing at x = 1.
200
Use the limit definition to state the end behavior of the polynomial functions.
f(X)= -8x^7+3x^2+5
200
Find the horizontal asymptote of
(x^3+x^2-9)/(x^5-3x^2-5x)
y = 0
200
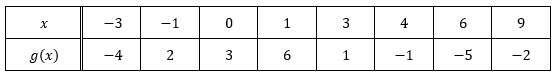
Selected values of the continuous function g(x) are shown in the table above. Use the values in the table to answer the following. Let
h(x)=-2g(x-3)-5.
Find h(0).
h(0) = 3
200
Calculate the average rate of change of the function 𝑓(𝑥) =
x^2-9x
in the interval 2 ≤ 𝑥 ≤ 7.
0
300
The table gives values of a function f for selected values of x. Is the function linear or quadratic? Justify your choice?
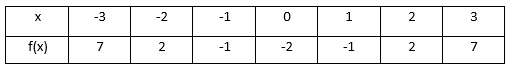
f(x) is quadratic; f(x) has a constant second difference over equal-length input value intervals OR the rates of change are changing at a constant rate.
300
Where is the relative maximum of the function? (Calc. okay)
h(x)=2x^3-4x
(-0.816, 2.177)
300
Find the zeros of the function.
(4-x^2)/(x-2)
-2
300
The graph of f(x) is shown in the figure below and has the domain [-3,3] and the range [-5,3].
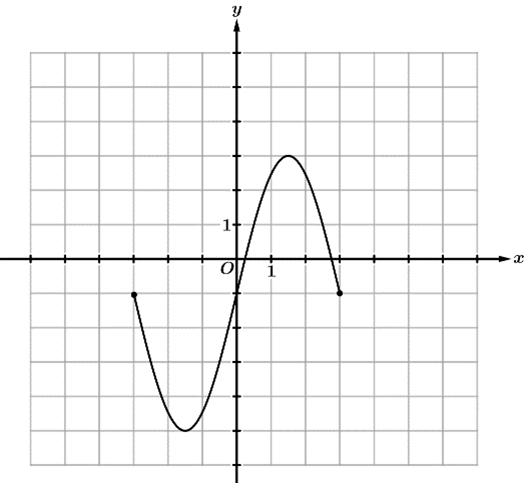
Let k(x)=-3f(2x)+1.
Find the domain and range of k(x).
Domain
[-3/2,3/2]
Range [-8,16]
300
Let g be an odd function that is strictly increasing. Selected values of g(x) are given in the table above. Find the values of the constants a, b, and c.
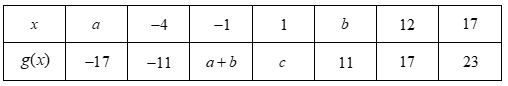
a = -12
b = 4
c = 8
400
Let
f(x)=x^2-4
The average rate of change of over the interval [c, 5] is equal to 3, where c is a constant. Find the value of c.
c = -2
400
Given the zero 4 − 𝑖, find all other zeros of the polynomial function
f(x)=x^4-8x^3+16x^2+8x-17
−1, 1, 4 − 𝑖, 4 + i
400
Find the zeros, vertical asymptote and holes if any.
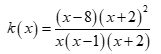
zero x = 8 (8, 0)
VA x = 0, x = 1
Hole x = -2
400
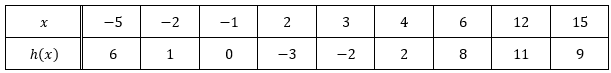
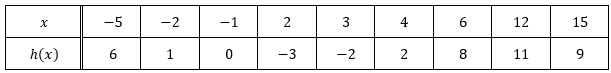
Selected values of the continuous function h(x) are shown in the table above. Use the values in the table to answer the following. Let
h(x)=6f(x+2)-3
Find f(4).
f(4) = 0
400
Find the line of best fit of the percentage of American homeowners.
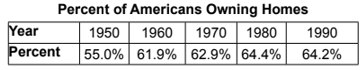
regression equation: _______
regression equation
-0.009786x^2+38.7647x-38325.5
500
Let p(x) be the function that results from applying three transformations to the graph of g in the order: a horizontal dilation by a factor of 1/2, a reflection over the y-axis, and a vertical translation by 1 unit.
Find the average rate of change of p over the interval [-1/2, 4].
g(x)=x^2-2
2/3
500
Which of the following statements are true about the polynomial function p(x)?
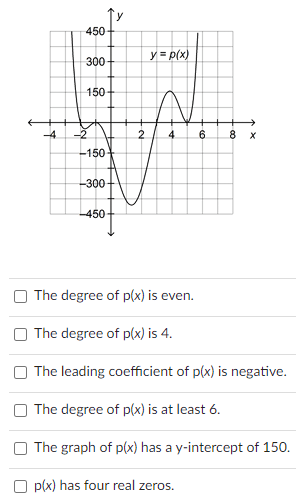
The degree of p(x) is even.
The degree of p(x) is at least 6.
p(x) has four real zeros.
500
Solve the following inequality. Answer using interval notation.
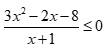
(-∞,4/3] ∪(-1,2]
500
Let
w(x)=2h(x-3)+1
Find w(w(2)).
w(w(2)) = 3
500
A sheet of 16 cm x 12 cm card is used to make an open box. Four identical squares are cut out of each corner. Then, the remaining card is folded to make an open box. For what value of x is the volume the largest?
x = 2.26