Solve if x = 2
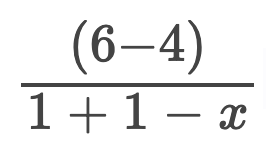
Undefined
2/ (zero)
Commute the terms of the expression:
3 + 5 =
5 + 3
Define: transformation
performing the same mathematical operation to both sides of an equation.
4/12 + 8/12 =
12/12 or 1
(-5)(3) =
-15
Define: Terms AND Factors
Term: a number/variable that is added or subtracted to another number/variable
Factor: a number/variable that is multiplied to another number/variable
|12 - 20| =
8
12 - 20 = -8
|-8| = 8
Solve the problem by associating the terms. Explain the association involved.
3 + 5 + 4 + 1 =
One example:
1. 5+4 = 9
2. 9+1 = 10
3. 10 + 3 = 13
What is the first transformation step in solving for g?
(2/3)g + (5/3) = 14
subtract (5/3) from both sides.
14 - (5/3) = (37/3)
14 = (42/3)
4/12 + 2/3 =
1
To add fractions, they must have common denominators.
2/3 = 8/12
4/12 + 8/12 = 12/12 or 1
(-5)(-5)=
25
Define: Additive Inverse
AND
Give an example of additive inverses
A number and its additive inverse can be added together to equal zero.
5 and -5
If hamburgers cost $2.00 each and fries cost $1.50 each, how much would we spend on combos for our class (11 students plus Mrs. Thornburg)?
$42
Price = (12)(2.00) + (12)(1.50)
Can the terms be commuted without changing the value of the expression?
(4)(1/2)(8) =
(4)(8)(1/2) = 16
(4)(1/2)(8) = 16
(1/2)(8)(4) = 16
Solve for f and list the transformations required for solving.
12f + 5 = 101
f = 8
1: subtract 5 from both sides
2: divide both sides by 12
(2/3)(2/3) =
4/9
Multiplying fractions is done by "multiplying across".
(2 x 2)/(3 x 3)
(16)/(-4) =
-4
(16) time (1/-4)
Define: Integers
Whole numbers, including zero and negative numbers
If hamburgers can be purchased in catering platters of 12 burgers for $1.50 per burger and $2.25 for packaging:
What would be the equation for the price of 36 burgers?
BONUS: How much would 36 burgers cost?
Price = (36)(1.50) + (3)(2.25)
BONUS Price = $60.75
What is the proper way to commute the terms in the following expression?
4 - 6 - 1
4 + (-6) + (-1)
6h + 3 = 4 + 2h
Combining like terms:
{h-terms} or {non-variable terms}
4h + 3 = 4 or 6h = 1 + 2h (or equivalent)
(2/3)(3/2) =
1
(2 x 3)/(3 x 2) = (6)/(6) = 1
Also (2/3) and (3/2) are multiplicative inverses. Their product is 1.
(-100)/(-25) =
4
(-100) times (1/-25)
Define: Multiplicative Inverse (Reciprocal)
AND Give an example of multiplicative inverses
Example: 5 and (1/5)
If car travels 65 miles/hour and travels 12 miles, how long did it travel (in hours)?
BONUS: how long in minutes?
12 miles distance/65mph rate = .18 hours
(.18 hours)(60min/hour) = 11 minutes
Use the order of operations to associate the terms and factors correctly to solve.
32 + 5(4) + 4
32 + (5)(4) + 4 =
9 + 20 + 4 =
14 + 20 = 29 + 4 = 25 + 9 = 33
Solve for w AND list the steps of transformation:
4w + 2w = 12 + 3w
w = 4
6w = 12 + 3w
3w = 12
(1/2)/(4/3) =
3/8
Keep (1/2)
Change / to x
Flip (4/3) to (3/4)
(1 x 3)/(4 x 2)
(-1)/0
Undefined.
Any number divided by zero is undefined because zero does not have a multiplicative inverse.
Define: division
multiplying a number by the multiplicative inverse of another number