Rewrite the equation in logarithmic form.
102=100
log(100)=2
You deposit $1500 into an account earning 4% annual interest. Write and solve an equation to find the balance after 3 years when the money is compounded continuously.
$1691.25
Evaluate the expression.
10log28
1000
Find the inverse of the function.
y=-3x+2
log-3(x)-2=y
Expand the expression.
log ((10y5)/x)
log(10) + 5log(y) - log(x)
Rewrite the equation in exponential form.
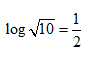
101/2=sqrt(10)
You deposit $1500 into an account earning 4% annual interest. Write and solve an equation to find the balance after 3 years when the money is compounded monthly.
A = 1500(1 + (.04/12))12(3)
$1690.91
Evaluate the expression.
93x-5=813x+2
x=-3
Find the inverse of the function.
y=log3(x-5)+11
y = 3x-11 + 5
Condense the expression.
ln(e3) + ln(e) - ln(e5)
-1
Rewrite the equation in exponential form.
ln(7.39)=2
e2=7.39
Nat is developing a new virus. He currently has 50,000 specimen. The specimen triple in amount every day.
How many specimen will there be in 3 days?
1,350,000 specimen
Evaluate the expression. (3 Decimals)
3e-x-4=9
x=-1.466
Find the inverse of the function.
y=2(3)x+1
log3((x-1)/2)=y
Expand the expression.
log3 ((7x)/y6)
log3(7) + log3(x) - 6log3(y)
Rewrite the equation in logarithmic form.
e-3=0.05
ln(.05)=-3
Jeff purchased a car for $40,000. The value of the car depreciates 10% each year.
When will the balance of the car be half of its original value? (2 decimals)
6.58 years
Evaluate the expression. (3 decimals)
5log(x-2)=11
x=160.489
Find the inverse of the function.
y = 3log8(x)-4
Condense the expression.
log5(x) + 4log5(y) - log5(z)
log5((xy4)/z)
Rewrite the equation in logarithmic form.
(1/3)-2=9
log1/3(9)=-2
Nat is developing a new virus. He currently has 50,000 specimen. The specimen triple in amount every day.
At what time will there be 6 billion specimen? (2 Decimals)
10.65 Days
Evaluate the expression.
log2(x) + log2(x-4)=5
x=8
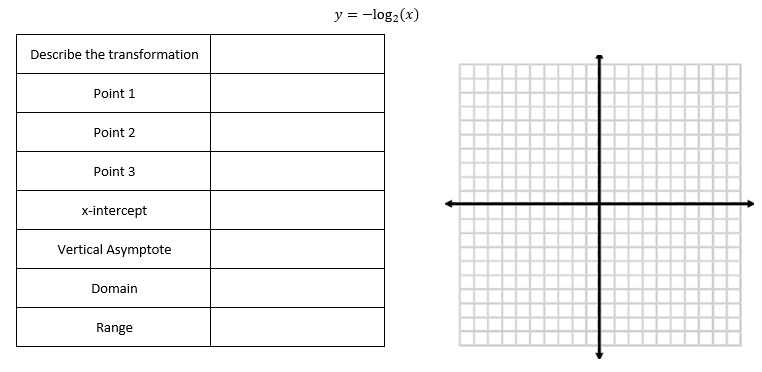
Reflection over x-axis
(2,-1)
(4, -2)
(8, -3)
(1, 0)
0
x>0
All real numbers
Expand the expression.
log3 (1/(az2))
log3(1) - log3(a) - 2log3(z)