If, y=a(bx ), What do a and b represent?
a=Starting point/y-intercept
b=growth factor
f(x)=a(.93)x
Does this functions represent exponential growth or decay? What is the percent growth/decay rate?
Exponential Decay
7% decay rate
A bunny population doubles every 6 months. If the starting population is 10, how many will you have after 3 years? What is the initial population? What is the growth factor?
initial population = 10
growth rate = 2
640 bunnies
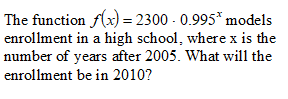
2243
A town has a population of 70,400 and decays at a rate of 4% every year. Write an equation represents the town’s population after x years?
y=70400(0.96)x
Which number is the y-intercept and which is the growth factor for the function f(x)=2(3)x
y-intercept=2
growth factor= 3
Find the equation for:
Passes through the points (0, 3.5) and (2, 31.5)
y=3.5(3)x
Annual sales of a fast food restaurant are $530,000 and increasing at a rate of 5%. What will the annual sales be in 6 years?
530,000(1.05)6 =$710,250.69
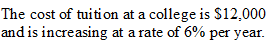
What will be the cost in 17 years?
$32,313.27
An element with mass 130 grams decays by 22% per minute. How much of the element is remaining after 7 minutes, to the nearest 10th of a gram?
22.8
What is the equation for the graph below?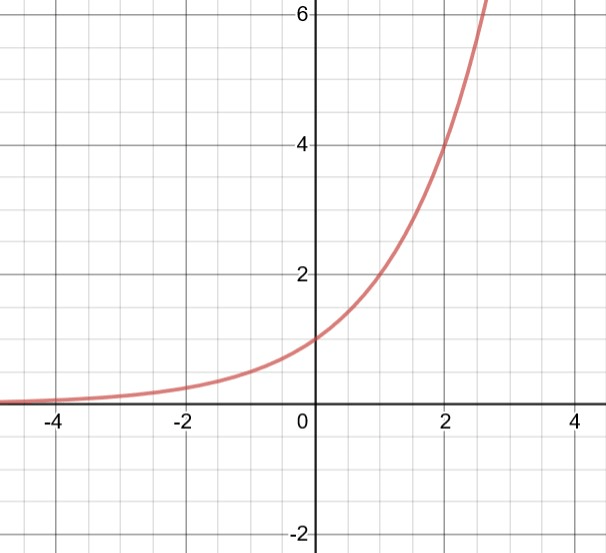
y = 2^x
Ms. Wiggins purchased a car for 26,400 and every year it decays by 12%. What can she expect the value of her car to be after 3.5 years?
f(x)=26400(.88)3.5=$16,876.92
The accompanying table shows the number of bacteria present in a certain culture over a 5 hour period, where x is the time, in hours, and y is the number of bacteria. Write an exponential regression equation for this set of data, rounding all coefficients to the nearest hundredth. Using this equation, determine the number of bacteria present after 11 hours, to the nearest whole number.
hours: 0 1 2 3 4 5
bacteria: 1800 1950 2154 2424 2730 3034
y=1768.43(1.11)x
5574
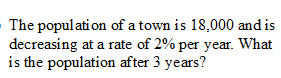
16,941 people
A town has a population of 13000 and grows at 4.5% every year. What will be the population after 13 years, to the nearest whole number?
23039
What's the growth factor?
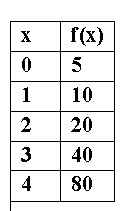
b=2
The accompanying table shows the value of a car over time that was purchased for 15400 dollars, where x is years and y is the value of the car in dollars. Write an exponential regression equation for this set of data, rounding all coefficients to the nearest hundredth. Using this equation, determine the value of the car, to the nearest cent, after 10 years.
years: 0 1 2 3 4
value: 15,400 13,409 11,881 10,056 8,975
y=15411.14(0.87)x
3828.49
The accompanying table shows the number of bacteria present in a certain culture over a 4 hour period, where x is the time, in hours, and y is the number of bacteria. Write an exponential regression equation for this set of data, rounding all coefficients to the nearest hundredth. Using this equation, determine the number of bacteria present after 10 hours, to the nearest whole number.
hours: 0 1 2 3 4
bacteria: 96 106 131 151 189
y=92.82(1.19)x
529
Is the function exponential growth or decay? 
Decay
Write an exponential function in the form y=abx that goes through the points (0,16) and (3,2000).
y=16(5)x
What is the rate? Is it a growth or decay?
y=204(0.0025)x
Decay
99.75%
The accompanying table shows the value of a car over time that was purchased for 18000 dollars, where x is years and y is the value of the car in dollars. Write an exponential regression equation for this set of data, rounding all coefficients to the nearest hundredth. Using this equation, determine the value of the car, to the nearest cent, after 13 years.
years: 0 1 2 3 4 5
value: 18,000 15,026 12,702 10,680 8,483 7,082
y=18217.12(0.83)x
1616.20
The accompanying table shows the number of bacteria present in a certain culture over a 4 hour period, where x is the time, in hours, and y is the number of bacteria. Write an exponential regression equation for this set of data, rounding all coefficients to the nearest thousandth. Using this equation, determine the number of bacteria present after 16 hours, to the nearest whole number.
hours: 0 1 2 3 4
bacteria: 279 310 343 382 457
y=274.779(1.127)x
1861
If you put $500.00 into an account and the account earns 3% interest, compounded quarterly. How much will be in the account after 5 years?
$580.59
Find the equation of the exponential function represented by the table:
x: 0 1 2 3
y: 0.1 0.05 0.025 0.0125
y=0.1(0.5)x