Factors & Rates
Functions
Growth and Decay
Compound Interest
Word Problems
100
Growth or decay?
y=52(0.83)^x
Decay
100
Write the exponential function that matches
y=3(2)^x
100
Write a scenario about cats that can be modeled by
y=23(1.47)^x
There are 23 cats living in a gang on the streets. This cat gang is growing at a rate of 47% each year.
You could use money, pickles, ants...you choose the context.
100
If only, like Hasbro, I might trademark the smell of this modeling compound: hints of cherry, vanilla, salt
Play-Doh
100
You want to save for a new X-box that cost $600 within the next 3 years. How much money will you need to put into an account that gains 15% interest compounded monthly?
$383.65 put into the account now.
200
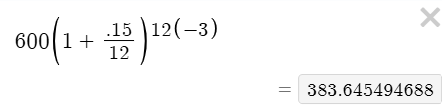
Growth or Decay?
y=0.54(1.036)^x
Growth
200
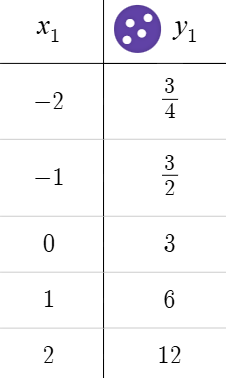
Write an exponential function to match the table
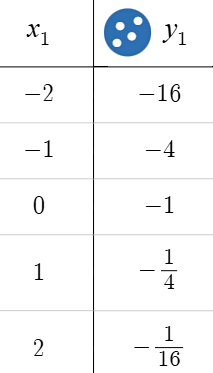
y=-1(1/4)^x or y=-(1/4)^x
200
In 1928 Alexander Fleming found that mold in a Petri dish had inhibited bacterial growth, leading to this antibiotic
Penicillin
200
If $2,500 is deposited into a new account that earns 2.1% interest compounded quarterly and there are no other deposits or withdrawals, how much interest is earned after 15 years?
$3,422.83 in the account after 15 years.
200
A piece of charcoal is found to contain 25g Carbon-14. The tree from which the charcoal came originally had 100 grams of Carbon-14. When did the tree die if the continuous exponential rate of decay is -0.00012096.
The tree died 11,460.77 years ago.
300
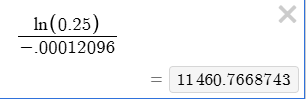
What is the rate?
y=52(0.83)^x
-17% or Decay of 17%
300
It's a planned function where someone famous is introduced to & socializes with audience members
Meet & greet
300
Aunty Kathy gives you a check for $150 for your birthday. Your mom convinces you to deposit into a savings account that already has $550 in it. If the account grows interest at 1.3% each year, how much will be in the account after letting it sit (not adding or taking any money out) for 8 years?
y=(150+550)(1+.013)^8
y=700(1.013)^8
=$776.20
after 8 years.
300
On the day he was born, Bart's grandma deposited $20,000 into a new account that earns 7.5% interest compounded continuously. Assuming there are no other deposits or withdrawals, find the total balance in the account on Bart's 18th birthday.
$77,148.51 after 18 years.
300
What interest rate would be needed to go from $100 to $1000 in an account that gains interest weekly for 20 years?
An interest rate of 11.53% would be needed.
400
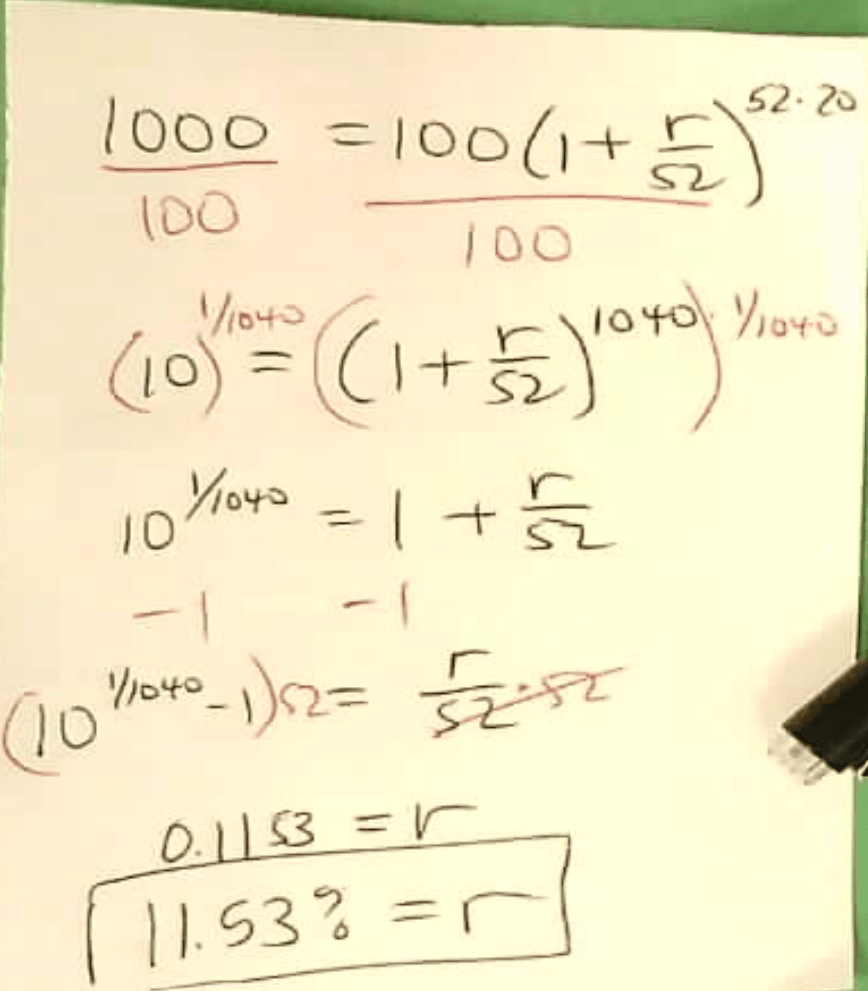
What is the rate?
y=0.54(1.036)^x
3.6% or Growth of 3.6%
400
Write the exponential function that matches the graph
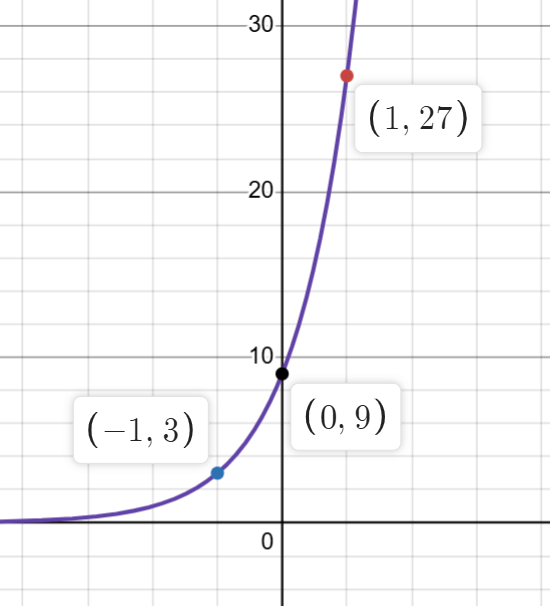
y=9(3)^x
400
The population of Austin, TX is growing at a rate of 6.7% each year. In 2010 there were 500,000 people. How many people will there be now, in 2025?
500000(1+0.067)^(2025-2010)
500000(1.067)^15
= 1,322,624
people
400
Pete has $400 in the year 2000. He puts it into an account that earns 3% compounded semiannually for 8 years.
He then takes what he earned over those 8 years and invests it into a new account that earns 5.6% compounded continuously until 2025.
How much INTEREST did he earn from 2000 to 2025?
Pete gained $915.11 in interest over those 25 years.
400
It means "to walk before"; a famous one begins, "We the People"
Preamble
500
Astronomer Vera Rubin said, "In a spiral galaxy, the ratio of dark-to-light" this "is about a factor of 10"
Matter
500
Write the exponential function that matches the graph below
y=50(1/5)^x
500
There are 1,500 termites in a particular colony. After being sprayed with insecticide the termite population decreases at a rate of 65%. How many would be left after 6 months?
y=1500(1-0.65)^(6/12)
y=1500(0.35)^0.5
887
termites left after 6 months
500
Which would pay more:
(A) A rate of 17.5% compounded daily
OR
(B) A rate of 17.45% compounded continuously
Option (A) will gain a slight bit more...
(A) 19.12% annually
(B) 19.07% annually
500
Helen deposited $3,000 at an interest rate of 9% compounded quarterly. How many years will it take for Helen's account to reach $10,000.
It will take 13.53 years to go from $3,000 to $10,000.
