Simplify:
\frac{a^2*c^3*d}{8acd^2}
Given:
a=2, c=3, d=4
9/16
Define similarity
When two (or more) shapes have corresponding sides that have been scaled consistently with some scale factor.
Cross multiply the following:
\frac{10}{x}=\frac{25}{6}
x=2.4
Which trig function takes opposite/hypotenuse?
\sin(\theta)
Explain how trig can be used to find the height of something
Answers vary
Write an equation of a line parallel to:
2x-8y=16
Any line with slope 1/4
Determine the scale factor given the following proportions of corresponding sides:
\frac{16}{5}=\frac{25.6}{8}=\frac{32}{10}
scale factor is 3.2
Explain why we can switch terms on the diagonals of proportions:
\frac{a}{b}=\frac{c}{d}
equal to:
\frac{d}{b}=\frac{c}{a}
When you cross multiply, a*d=b*c is still the result.
Explain why
\sin(\theta)<1
always
Because the hypotenuse will always be bigger than opposite. And so we have it that we're always dividing by a larger number. Can't be bigger than 1 (or even equal)
Suppose you have a 12 ft ladder propped up against a wall with an angle (on the ground) of 35°. How far up the wall does the ladder reach?
About 7 feet (6.88)
A:{2,4,6,7,8,10}, P:{2,3,5,7,}, U:{1-10}
What is
A \cap P
A \cap P:{2,7}
What is the scale factor relationship between Triangle A and Triangle C?
5\triangle A=\triangle B, 3\triangle B=\triangle C
15\triangle A=\triangle C
Solve for x
\frac{8}{3x-2}=\frac{12}{15}
x=4
Explain why
\tan(\theta)>1
is a possibility
Because, opposite may be much larger than adjacent, producing a fraction like
\frac{8}{3}=2.\overline{6}
Suppose you have a 12 ft ladder propped up against a wall with an angle (on the ground) of 35°. Explain why the height the ladder reaches will never be >12. (Even if you change the angle!!)
because the hypotenuse will always be the largest side!
Assume P(A) and P(B) are independent
if P(A)=0.85, P(B)=0.2 \text{ what is} P(A\cap B)
P(A\cap B)=0.17
Triangle A has a base of 4 and a height of 3. That makes the area 6. If we double the side lengths, how much does the area change? Show using the formula: A=(1/2)bh
4 times.
Keefe sets up a proportion, cross multiplies, and gets the following result.
1296=1340
What can Keefe conclude?
The two figures are NOT similar
Find theta if:
\sin(\theta)=10/11
\sin^{-1}(\frac{10}{11})\approx 65°
Given the following triangle, find the area:
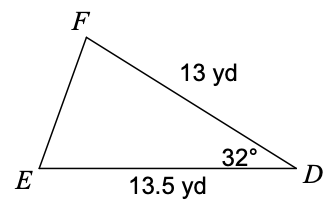
\approx 46.5
Write a quadratic equation with the following points:
(3,0),(-4,0), (0,-12)
f(x)=x^2+x-12
Triangle A has a base of 4, a height of 3 and a hypotenuse of 5. The perimeter is given by adding up all the sides. If we scale the triangle by 5, what is the new perimeter? How does the perimeter change with scale factor?
New perimeter is 5(3)+5(4)+5(5)=5(3+4+5)=5(12).
Solve for x
\frac{6}{5x+6}=\frac{3}{x-3}
x=-4
Which angle satisfies the following equation?
\sin(20°)=cos(\theta)
cos(70°)
Find the length of ED given that the area is: 83.2
16