Is it a Function:
Graphs?
Graphs?
Create a function table:
Is it a function: points?
Is it a Function: Equations, Tables, and Mapping
Vocabulary
100
No, using the vertical line test, the line hits more that 2 points.
100
Function Rule: y = 3x - 5
Input, x
4
7
-3
-6
Output, y
2
27
-14
-23
100
Does this represent a function? Explain
(1,2) (2, 3) (3,4) (4,5)
yes, because each input has exactly one output.
100
Does the relation represent a function?
Yes, each input has its own output.
(10,15)
(20,45)
(30,25)
(40,35)
100
What is a function?
Each input has exactly one output
200
Which relation represents a function?
The first graph. Using the vertical line test, each input has exactly one output.
Bonus Information: The second graph is not a functions, because when using the vertical line test, the line hits more than one point.
200
Function Rule: y = 2x + 1
Input, x
2
5
-3
-2
Output, y
5
11
-5
-3
200
Does this represent a function? Explain
(-2, 3) (4, 5) (-2, 6)
No, because the x value (input) -2 has more than one output
200
Which relation DOES NOT represents a function?
B--It is the only map where each does not have its own output.
a--q
a--r
200
What is the vertical line test? What does it mean to pass the vertical line test?
A graph represents a function when no vertical line passes through more than one point on the graph.
300
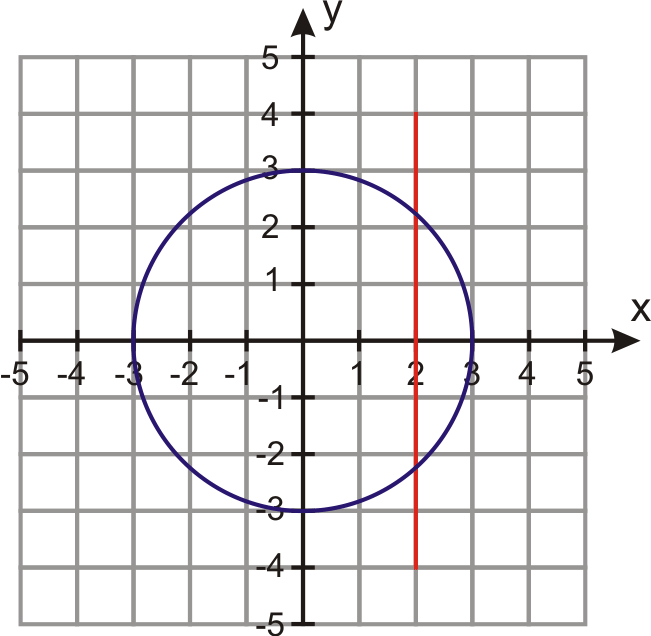
Does the relation represent a function.
No, using the vertical line test, the line hits more that 2 points.
300
Function Rule: y = 4x - 2
Input, x
3
15
-6
-5
Output, y
10
58
-26
-22
300
Does this represent a function? Explain
(0, 1) (1,1) (2,1) (3,1)
yes, because each input has exactly one output
300
Which table DOES NOT represents a function.
T--Each input does not have its own output.
300
What is an input?
the x values
400
Which of the following graphs IS a function? 
x--it is the only graph each input has its own output.
400
Function Rule: y = -6x - 4
Input, x
-2
6
-5
8
Output, y
8
-40
26
-52
400
Does this represent a function? Explain (-2,0) (-1,0) (-1,1) (0,1) (1,2) (2,2)
No, because the x value (-1) has more than one output.
400
4----Each input has its own output.
400
What is an output?
The y values.
500
Which graph represents a function.
A. Using the vertical line test, each input has exactly one output
500
x y
1 42 4
3 4
4 4
Yes, because each input has exactly one output
500
Does this represent a function? Explain (-2,2) (-1,2) (0,2) (1,0) (2,0)
yes, because each input has exactly one output.
500
Does this relations represent a function? Explain.
(-2,3), (2,3), (-1,4), (5,2), (0,6), (4,3)
The relation represents a function. Each input has its own output.
500
What is a linear function?
function whose graph is a straight line