Transformations
Angles/Triangles
Similarity
Trig
100
How many reflective lines of symmetry does a regular hexagon have?
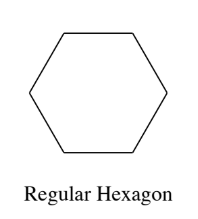
6
100
Solve for x.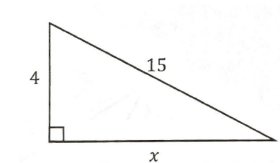
x=14.46
100
What are the 3 similarity statements to prove similarity?
SSS, AA, SAS
100
Solve for x. 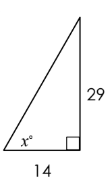
x=64 ^o
200

2x+2+3x-1+x+4=23
x=3
200
Use your angle relationships and solve for x.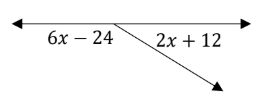
x=24
200
If the two triangles are similar. Solve for
bar (QP)

bar(QP) = 8
200
Use special right triangles to solve for x and y.
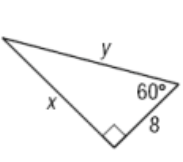
y=16
x=8sqrt3
300
ΔABC is translated so that A’ has the
coordinates (-1, 2). Translate ΔABC and label the coordinate points.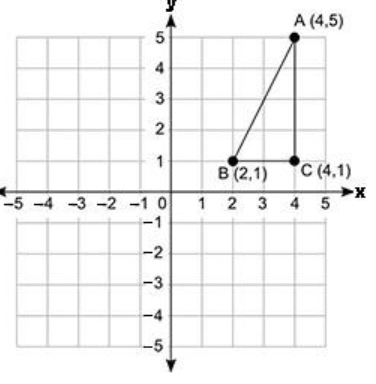
A' = (-1,2) B' = (-3,-2) C' = (-1,-2)
300
Determine the minimum and maximum limits for each missing side length. 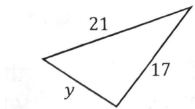
4<y<38
300
Create a flowchart proof to show these triangles are similar.
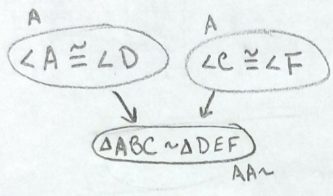
300
Solve for x. Hint: Use law of sines!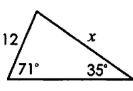
x=19.8