Parallel Lines and Transversals
Algebra Proofs
Segment Proofs
Angle Proofs and Linear Equations
Conditional Statements
Definitions
100
The name of the line that cuts the parallel lines.
What is a transversal?
100
The first reason on any algebra proof is this ____.
What is given?
100
Point a is in between point C and point B. Point C and point B are collinear. Therefore CA + AB = AC. This is an example of which postulate?
What is segment addition postulate
100
The following is an example of this property:
m/_1 = 150^o
m/_1 + m/_2 = 180^o
150^o + m/_2 = 180^o
What is substitution?
100
Write the statement as a conditional statement: All students love geometry
What is, if you are a student, then you love geometry?
100
Supplementary Angles add up to this many degrees.
What is 180°?
200
An example of angles that are on the same side of the transversal and are congruent.
What are corresponding angles?
200
It's the property used here:
x + 8 = 58
x = 50
What is the subtraction property of equality?
200
Give an example of the segment addition postulate, with a drawing. (Use the points A, B, and C).
AB + BC = AC
200
What is the slope for the line that is perpendicular to the following:
y = 3x +2
What is -1/3?
200
What is the hypothesis of the statement...
If the students study, then they will get a good grade on the next test.
What is, the students study?
200
Through 3 noncollinear points there is exactly one________
Plane
300
An example of angles that are on the same side of the transversal and are supplementary.
What is a linear pair, or same side interior/exterior?
300
This would be an example of the which property?
If /_A+/_B=180° and /_B+/_C=180°
then
/_A=/_C
Transitive
300
True/False. Definition of congruence is only used with segment proofs.
False.
300
How would you graph the line that is perpendicular to y = 2x + 6 and passes through (3,2)?
Determine the slope of the line that is perpendicular, use that slope(rise/run) from the point (3,2) to get to the new point. Graph the line between the two points.
(3,2) move down 1 over 2, this would be at (5,1)
300
What is the conclusion in the statement...
If you live in Saint Joseph, then you live in Missouri
What is, you live in Missouri?
300
A pair of opposite angles made by two intersecting lines that are congruent.
What are vertical angles?
400
An example of angles that are on opposite sides of the transversal and are congruent.
What are alternate interior/exterior or vertical angles?
400
It's the last reason for the following algebra proof:
What is the division property of equality?
400
This reason justifies statement 2 in the following proof.
What is the definition of midpoint?
400
This theorem tells us that
If
/_1 and /_2 are supplements
and /_2 and /_3
are supplements.
Then
/_1 cong/_3
What is the congruent supplements theorem?
400
What is the contrapositive of the following statement
If a number is prime, then it is odd.
What is, If a number is not odd (even), then it is not prime.
400
Two angles that are next to one another, have a common side, a common vertex, and don't overlap.
What are Adjacent Angles?
500
The Perpendicular Transversal Theorem states this.
What is: in a plane, if a line is perpendicular to one of two parallel lines, then it is perpendicular to the other?
500
It is a logical 2nd statement (assuming the first is a given):
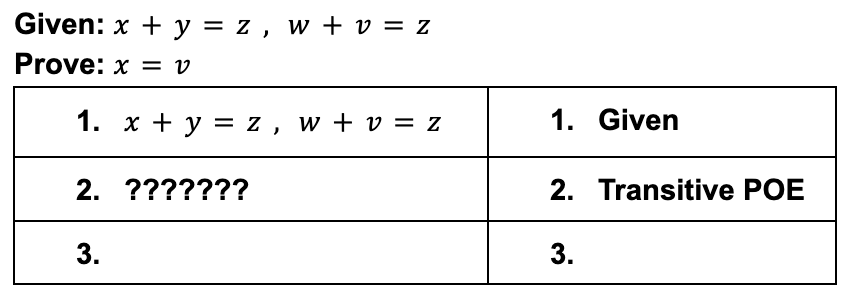
What is x + y = w + v?
500
It would be the last reason in the following proof:
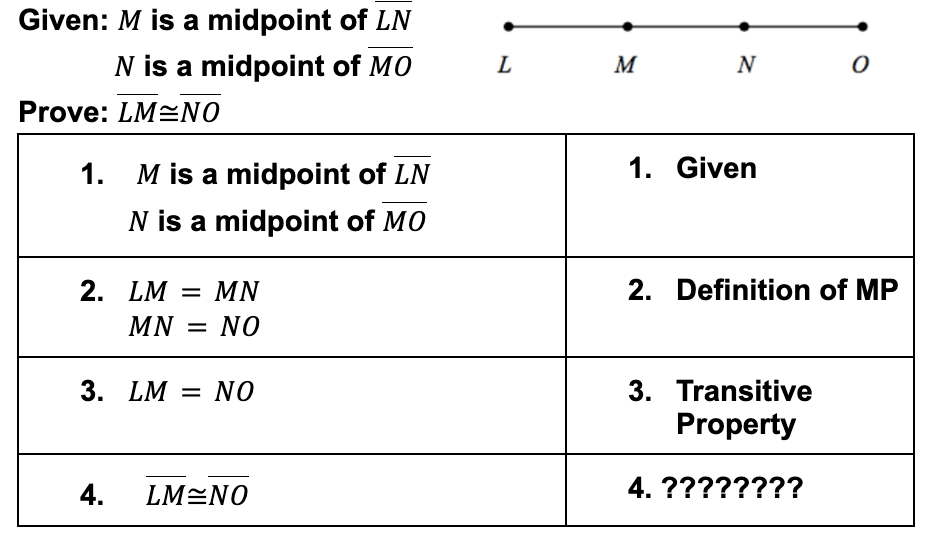
What is the definition of segment congruence?
500
Give statement #6
What is
m/_1 + m/_5 = 180^o?
500
This is the statement that you use to connect a conditional with its converse to make a biconditional.
What is If and only if?
500
Write a conclusion and identify which law is used below for the two conditional statements:
If a students studies for 2 hours, then the student gets and A.
If the student gets and A, then their parents will be happy.
What is law of syllogism
If a student studies for 2 hours, then their parents will be happy.