Unit 1: Reasoning
Unit 5: Inequalities
Unit 3: Lines and Angles
Unit 4: Triangle Congruence
Unit 5: Triangle Properties
100
This is the negation of the converse of a conditional statement.
What is contrapositive.
100
This is the range of third sides for 2 and 10.
What is 8 < x < 12?
100
Given two parallel lines and a transversal, one alternate interior angle is 80, what is the other angle?
What is 80, alternate interior angles are congruent!
100
The two congruent sides of an isosceles triangle.
What are the legs.
100
Connects the midpoints of two sides of a triangle.
What is a midsegment.
200
The two conditionals that make up the biconditional.
An angle is an acute angle if and only if its measure is between 0 and 90.
What is
-If an angle is acute, then its measure is between 0 and 90. -If an angle's measure is between 0 and 90, then the angle is an acute angle.
200
This is the range of possible sides of the triangle.
3 and 5
What is 2 < x < 8
200
Given two parallel lines and a transversal, one of the same side exterior angles is 3x + 50, the other is x + 20. Find the value of x.
What is x = 27.5
200
Given: RSTUV is congruent to KLMNO
Complete: LM is congruent to
What is ST.
200
This many triangles are in a hexagon.
What is 4 triangles.
300
The conclusion for the following statement. If a student is on the basketball team then the student has passing grades. De'Anthony is on the basketball team.
What is De'Anthony has passing grades.
300
This type of triangle has side lengths 4.8 km, 28.6 km, 29 km
What is a right triangle
300
3 angles of a triangle are x, 2x and 3x. Find the measure of the largest angle.
What is 90. (x = 30)
300
The vertex angle of an isosceles triangle is 40, what is the measure of one of the base angles.
What is 70.
300
The point of concurrency of the angle bisectors.
What is the incenter.
400
The law for the following statement: If you miss the bus, then you are late to school. Tina missed the bus. Therefore, Tina is late to school.
What is Law of Detachment.
400
This is the order the angles in each triangle from smallest to largest.
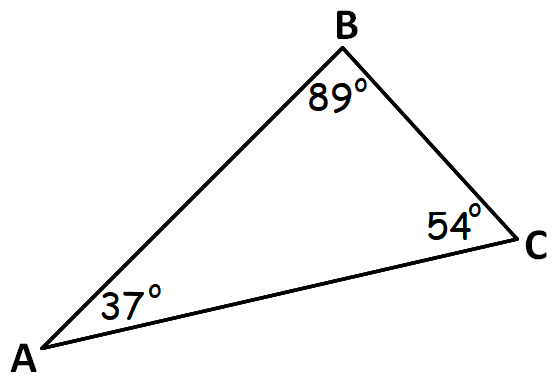
What is BC, AB, AC.
400
The measure of an exterior angle of a regular octagon.
What is 45.
400
Given triangles ABC and DEF with AB congruent to DE and AC congruent to DF, what angle must be congruent to prove the two triangles congruent through SAS.
What is angle A is congruent to angle D.
400
The point of concurrency of a triangle that is equidistant from the vertices.
What is circumcenter (perpendicular bisectors)
500
The sequence that describes the pattern 3, 12, 48, 192
What is Geometric Sequence.
500
Do these angle measurements create a triangle? If so, why?
34°, 34°, 112°
What is yes, triangle sum theorem.
500
The measure of an interior angles of a regular hexagon.
What is 120.
500
Explain why you cannot use AAA to prove two triangles congruent.
What is The corresponding sides of the two triangles may not be congruent. All angles have 3 angles that have a sum of 180, this does not say anything about the sides!
500
This is used to describe that one side in one triangle is bigger than another side in another triangle when the other two sets of sides are congruent.
What is SAS Inequality Theorem.