Semester 1 maths
Gradients
Midpoints
Negative Numbers
Bastick's Round
100
Evaluate:
23
8
100
Find the gradient of the line:
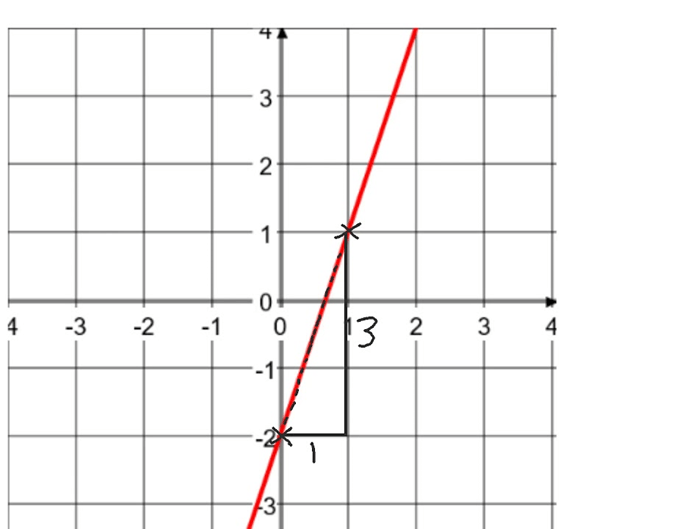
3
100
Find the midpoint of this line segment:
(4, 6)
100
10 - 12
-2
100
Where are the Olympics being held this year?
Paris
200
Simplify:
(x^17xxx^3)/x^5
x^15
200
find the slope of (4,4) and (6,12)
4
200
Find the midpoint of the line segment:
(1, 2)
200
-4 - 7
-13
200
The Olympics have only been cancelled 4 times.
1916, 1940, 1944 and which other year?
2020
300
Find the volume of the prism below (bonus 100 points for using the correct units)
40cm3
300
find the slope of (2,5) and (7,6)
1/5
300
Find the midpoint between the points:
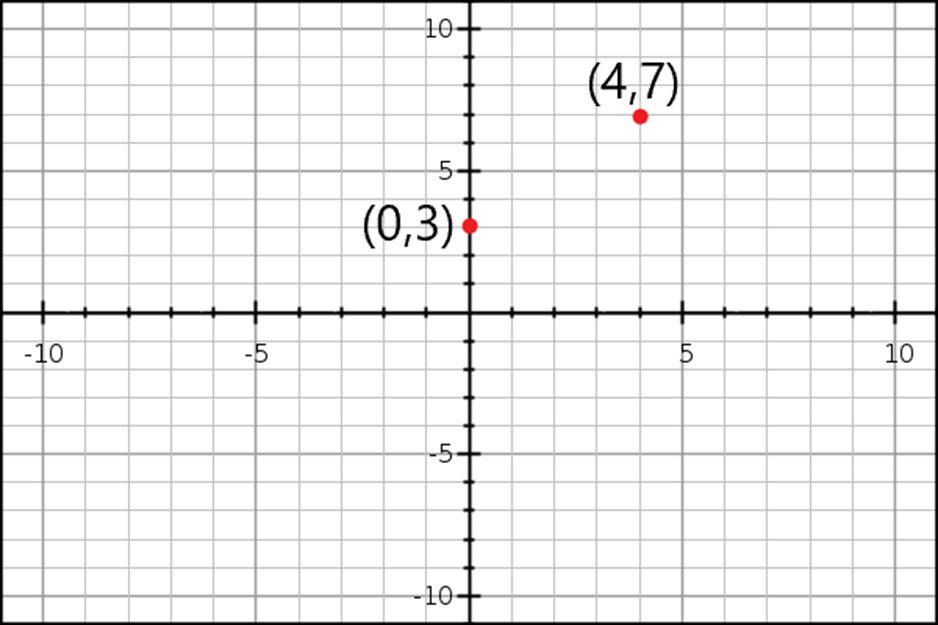
(2, 5)
300
5 - - 13
18
300
In what city was the first modern Olympics held?
Athens
400
Find the value of x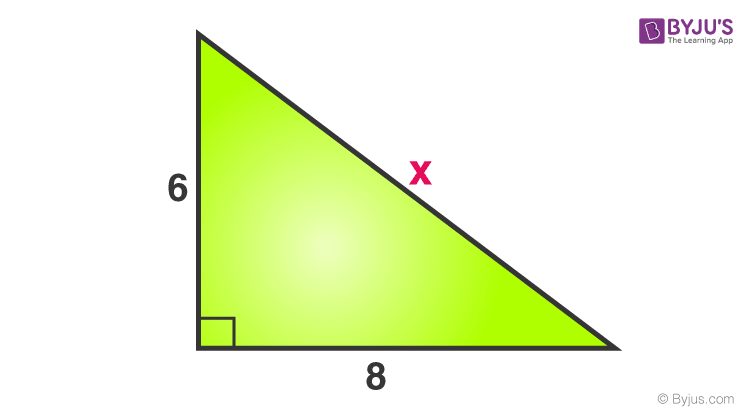
10
400
find the slope of (3,0) and (1,2)
-1
400
Find the midpoint of a line segment that goes from (-8,-8) to (5, -12)
(-1.5, -10)
400
-4 + - 13 + 5
-12
400
What colour are the Olympic rings?
blue, yellow, black, green & red
500
Write an expression for x
sin(22) = x/4000
500
A line with a gradient of -3 passes through the point (-2, 6). Find the coordinates of one other point that the line will also pass through
(-3, 9)
(-1, 3)
(0, 0)
(1, -3)
500
A line segment goes from point A to point B.
If point A has the coordinates (3, 4) and the midpoint of this line segment is (-2, 5), find the coordinates of point B.
(-7, 6)
500
(-2xx4--16)/(-2)^2
2
500
Only 5 countries have never missed a modern Olympics: France, Greece, Switzerland, Great Britain and which other country?
Australia