Properties of Numbers
Factoring and Divisibility
Expressions, Equations, and Identities
Rational Numbers and Ratios
Proportional Reasoning and Rate
100
Rewrite 45 in the form A(B-C).
Answers will vary. One way to do so is
5(10-1)
100
Factor the sum 12+27 by finding a common factor.
3(4+9)
Both 12 and 27 share the factor of 3. We can factor that out to the outside of the parentheses, dividing both numbers by 3.
100
Complete the sentence:
The difference between an equation that is sometimes true and an equation that is an identity is...
An equation that it sometimes true has at least one solution that makes it true. For example,
2x+1=5
is only true when
x=2
An equation that is an identity will be true for all values of
x
because the left and right sides of the equation are different representations of the same expression. For example,
2(x+1)=2x+2
is an identity.
100
You eat one half of a cake. The next day you eat one third of what is left. The next day you eat one fourth of what is left. After this, what fraction of the cake remains?
1/4 of the cake is left.
After the first day, 1/2 of the cake remains. The next day, 2/3 of that amount remains. And the last day, 3/4 of that amount remains:
1/2*2/3*3/4=1/4
100
If a 24-foot tall building casts a shadow of 14 feet, how tall is a tree that casts a shadow of 56 feet?
96 feet
Let's set up a proportion here. We'll use "height"/"length"="height"/"length" :
24/14="tree height"/56
"tree height"=(24/14)*56=96
200
You have $23 in your Venmo account. You get paid $15 per hour to babysit your neighbor's toddler. You want to buy a pair of sneakers that cost $136. Write an equation that can be used to determine how many hours, h you must babysit until you can afford these sneakers?
h=(136-23)/15
First, we want to know how much more money we need to make. We need $136 and already have $23, so the amount we need to make is $136-$23=$113. To find out how many hours we need to babysit, we would divide that amount by $15.
200
Show that when you add two consecutive odd integers, the result is always divisible by 4.
(2A+1)+(2A+3)=4A+4=4(A+1)
First, we represent an odd integer in the form of 2A+1. To represent a consecutive odd integer, you'd then add 2 to that, getting 2A+3. Then add them together and simplify.
Notice that 4(A+1) is divisible by 4.
200
If A=3k+1 and B=-5k+24, and 2A=B then the value of k is...
k=2
Substitute the information given into 2A=B:
2(3k+1)=-5k+24
6k+2=-5k+24
6k+5k=24-2
11k=22
k=2
200
Find 2/3 of 1/2 of 6/7.
2/7
It doesn't really matter what order you multiply these fractions, but we can first find (1/2)*(6/7)=(6/14)=(3/7).
Then take (2/3)*(3/7)=(6/21)=(2/7).
200
The racetrack at Charlotte Motor Speedway is 3/2 miles long. If the "Coca Cola 600" is a 600-mile race, how many times will the winner go around the track?
400 times
We could think of this as 600/(3/2) or 600*(2/3), which would be 1800/3 or 400 times.
300
Rewrite -90 in the forms:
(A+B)(C-D) and
DtimesEtimesF
Answers will vary. Examples include:
(5+4)(6-16)
2times5times-9
300
Decompose 140 as a product of a number and a sum, then draw a picture to represent this product using the area model of multiplication.
Answers will vary. One example is below:
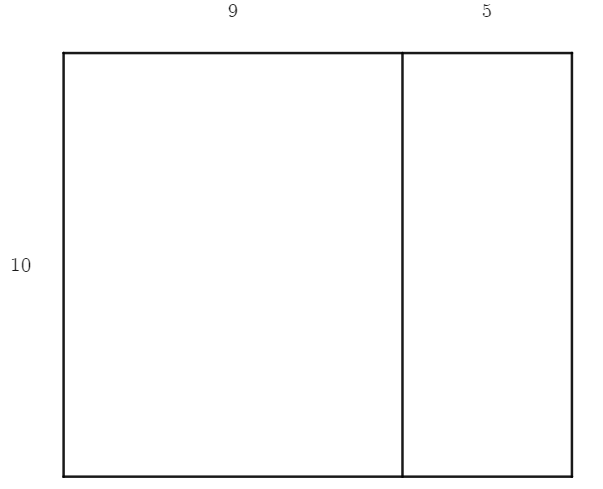
Notice that the left side of the square is a whole number, but the top of the square is the sum of two numbers. When we multiply these, we get the area of the entire square.
300
If 7(14+x)=5y, then what is x in terms of y?
x=(5y-98)/7
First, distribute, then isolate x:
98+7x=5y
7x=5y-98
x=(5y-98)/7
300
If 10/13x=15, what is the value of x?
39/2
There are many ways to solve this. One way is to multiply both sides by 13/10. This eliminates 10/13 on the left side of the equation, and gives us:
x=(15)(13/10)=195/10=39/2
300
Nate walks 39.7 meters in 45.3 seconds. If he walks at the same rate, approximately how many meters will he walk in 6 minutes?
Approximately 320 meters. He walk about 40 meters every 45 seconds, and there are 8 total 45 second intervals in 6 minutes.
400
You have $12 in Apple Pay. The local pizzeria is cashless and contracts you to deliver orders every Saturday. You get paid $3 per delivery, plus tips and $40 to cover gas, all of which goes directly into your Apple Pay account. Your gas tank is half full, so you spend only $18 to fill it before your shift. Write an expression that can be used to calculate your Apple pay balance at the end of your shift.
Answers might slightly vary. Two possibilities are below:
A=12+3p+t+40-18
A=3p+t+(12+40)-18
400
Determine whether the difference of two odd integers is always odd, always even, or could be either even or odd. Prove your claim.
The difference between two odd integers is always even!
(2A+1)-(2B+1)=2A-2B=2(A-B)
We represent an odd integer as 2n+1 (where n can be anything), which lets us set up this difference.
400
What must be true of the ratio c/d in order for 4(c+3)=5d+12 to be an identity?
c/d=5/4
In order for this to be an identity, both sides need to be equal:
4(c+3)=5d+12
4c+12=5d+12
4c=5d
Therefore, the ratio of c/d=5/4.
400
Bakari is making his first pizza and he uses 8/7 cups of shredded mozzarella on the entire pie. If the pizza is cut into 6 slices, how much shredded mozzarella is on each slice?
There is 4/21 cups of mozzarella on each slice:
1/6(8/7)=8/42=4/21
400
If 18 grams of Jolly Ranchers contain 70 calories, how many calories are in 90 grams of Jolly Ranchers?
There are 350 calories. We set up a proportion of grams to calories, then solved for x:
18/70=90/x
18x=6300
x=350
500
Rewrite 33 in the form (A+B)(A-B).
(17+16)(17-16)
Notice that A and B are used twice in this expression, so those numbers must appear in those respective places.
500
Explain how you can calculate 25^2 using mental math.
We can use the distributive property in various ways. One example below:
25(10+15)=(25*10)+(25*15)
=250+(25*10)+(25*5)
=250+250+125=625
500
A man is 40 years old and his son is 8 years old. In how many years will the man be 3 times as old as his son?
The man will be 3 times his son's age in 8 years, when the man is 48 and his son is 16.
One way to solve this problem is by representing the situation algebraically and solving for x:
40+x=3(8+x)
500
If a pizza has a/b cups of cheese spread evenly over c slices, how much cheese is on each slice?
a/(bc)
We can think about this as a/b divided by c, or a/b times 1/c:
(a/b)*(1/c)=a/(bc)
500
What is the difference between a ratio and a rate? Give an example of each.
A ratio is a relationship between two quantities that share the same units, e.g. miles and miles.
A rate is a ratio of quantities that have different units, e.g. miles and hours.