Properties and Number Sets
Equations and Expressions
Geometric Solids
Misc 1
Misc 2
100
The ________ property guarantees that if
h + w = p - 4, then p - 4 = h + w
symmetric
100
Evaluate: -d[(b-c)-(b+c)] if d =3, b = 2 and c = -5
-30
100
How many faces are on an octagonal prism?
10
100
Build the LCM of
2^7*3^10*5^30*11
and
3^9*11^4*17^2
2^7*3^10*5^30*11^4*17^2
100
Find the volume of a sphere of radius 2 meters.
(32pi)/3 "cubic meters"
200
Which property is demonstrated?
-3(2x + 5y) = -6x - 15y
distributive
200
Evaluate:
-3^3-(-3)^3-3^2-abs((-2)^2-2^3)
-13
200
Find the volume of a cone whose height is 6 meters and radius is 5 meters.
50pi " m^3"
200
Build the GCF of
2^7*3^10*5^30*11
and
3^9*11^4*17^2
3^9*11
200
A pyramid has a square base 6 meters on each edge and height of 3 meters. Find its volume.
36 cubic meters
300
List all number sets to which 3.14 belongs.
Real, rational
300
Solve:
x-2(x-2)=-5-(3x+3)
x = -6
300
Find the surface area of a sphere with diameter 10 meters.
100pi " square inches"
300
Write in scientific notation:
349000 * 10^-9
3.49 * 10^-4
300
A pyramid has a square base 6 meters on each edge and slant height of 5 meters. Find its surface area.
96 square meters
400
Simplify:
3sqrt 7 - 5 sqrt 10 + 6 sqrt 7
9sqrt7-5sqrt10
400
Simplify:
-3(4a + b) + 7a - 4b
-5a - 7b
400
Find the surface area of a cone with radius 3 meters and height 4 meters.
24pi " square meters"
400
Find the exact volume:
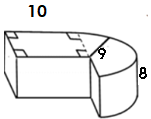
(720+324pi) " cubic units"
400
A cone's base has radius 7 inches and slant height of 10 inches. Find its surface area.
119pi " square inches"
500
All decimals that stop or have repeating patterns are ________.
rational
500
Simplify:
(12div6*2-(1/3)^-1)/((pi-5)^0-2)
-1
500
A cube is 7 meters on each edge. Find its volume in mL.
343,000,000 mL
500
Find the surface area
(484+153pi)
"square units"
500
Find the exact surface area:
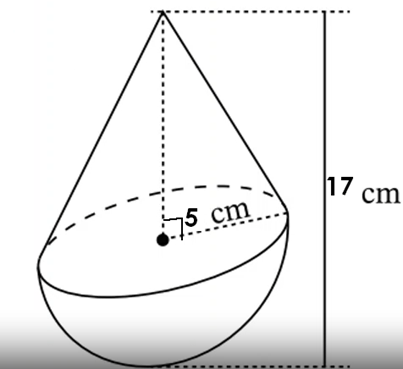
115pi" m2"