
Find the domain & range.
Domain: [-2,5]
Range: [-2,3]
If $15,000 is invested at an interest rate of 4.75% per year, compounded continuously, find the value oft he investment after 10 years.
$24,120.21
ln(e^(2x - 5))
2x - 5
Find the terminal point for
t = (2pi)/3
(-1/2, sqrt(3)/2)
 Find the domain and range in interval notation.
Find the domain and range in interval notation.
Domain: [-2,5]
Range: [-2,3]
If f(x) = 3/x and g(x) = 2x + 3, find f(g(x))
1/(2x + 3)
Solve the inequality:
(x + 2)/((x - 1)(x - 5))>=0
[-2,1) U (5,oo)
Solve for x:
log4(3x + 1) = 3
x = 21
Find the exact value:
tan((11pi)/6)
-sqrt(3)/3
Find the average rate of change from t = 2 to t = 5 for
f(t) = t^3 + 3t
10
Find the domain of
f(x) = 5/(x + 3)
x != -3
(-oo,-3) U(-3, oo)
A soft-drink vendor at a popular beach analyzes his sales records and finds that if he sells x cans of soda pop in one day, his profit (in dollars) is given by P(x). What is his maximum profit per day, and how many cans must he sell for maximum profit?
P(x) = -0.001x^2 + 4x - 1875
Max Profit: $2125
How many cans? 2000
Use the Laws of Logarithms to expand the expression.
log((x^5 sqrt(y))/z^3)
5 log x + (1/2)log y - 3 log z
Find the cos t if sint = 7/25 and the terminal point t is in the II quadrant
-24/25
Two polynomials P and D are given. Use either synthetic or long division to divide P(x) by D(x).
P(x) = 2x^3 + x^2 + 3x + 7, D(x) = x + 2
2x^2 - 3x + 9 -11/(x+2
Find the inverse of
f(x) = 2x^3 +4
root(3)((x - 4)/2
Find an equation of the line that passes through (1,-5) and is perpendicular to the line 3y -2x = 3
y = (-3/2)x - (7/2)
Use the Laws of Logarithms to combine the expression.
5 log(x) +3 log y - log(z − 1)
log((x^5y^3)/(z - 1))
Find the amplitude, period, and phase shift of the function
y = -4sin3(x + pi/2)
A = 4
P =
(2pi)/3
Phase shift: Left
pi/2
Find the horizontal and vertical asymptotes for:
(3x^2 + 4)/(x^2 - 4)
HA: y = 3
VA: x = 2, x = -2
Express in vertex form:
f(x) = x^2 + 4x + 9
f(x) = (x + 2)^2 +5
Solve the equation graphically in the interval [-1,5]. State answer(s) rounded to two decimals
x^3 + 14.2x^2 -4.8x - 12.4 = 0
-0.80, 1.08
For the following equation, find the exact solution of the exponential equation in terms of logarithms.
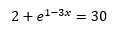
2 + e^(1-3x) = 30
(ln(28) - 1)/-3
Evaluate:
cos^-1 (-1/2)
(2pi)/3

Find the interval(s) where f is increasing; where f is decreasing.
Increasing: (0,3)
Decreasing (-2,0) and (3,5)