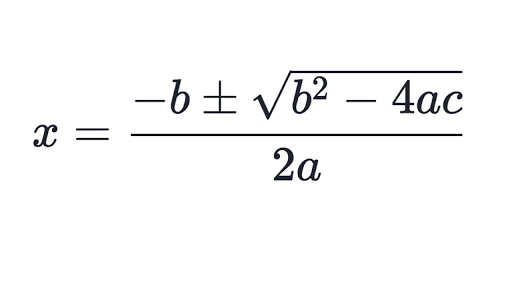Solving by factoring
Completing the square
Quadratic formula
Vertex form
Applications
100
Solve this quadratics by factoring.
x2 + 2x = 3
x2 + 2x - 3 = 0
= (x-1)(x+3) = 0
x = 1 or x = 3
100
Solve.
x2 - 4x - 32 = 0
x = 8, x = -4
100
The discriminant tells you the _________ and ________ of the roots
number and nature
100
What is the vertex of y =( x -4)2 + 7 ?
(4,7)
100
solve the following system
y=x2+5x + 6
y +x = 1
x2 + 6x + 5
(x+5)(X+1)
x=-5 x=-1
200
Solve this quadratic equation by factoring.
X2 + 16 = 10x
x2 - 10x + 16 =0
(x-2)(x-8)
x = 2 or x = 8
200
x2 + 9x + p = 7, find p that makes the left side of the equationa perfect square trinomial
p = 81/4
200
Find the discriminant adn describe the number AND nature of the roots.
6x2 - 17x = -21
Discriminant is -215, 2 imaginary roots
200
What is the vertex of (x+9)2 - 40 = y
((-9,-40)
200
After scoring the game winning touchdown, a football player spikes the ball. The path of the ball can be modeled by the function f(t) = -16t2 + 15t + 8. Use the discriminant to determine if the football reaches a height of 12 feet after it has been spiked. Justify your answer mathematically.
The discriminat is -31, therefore there are 2 imaginary solutions to this problem. The football will NOT reach a height of 12ft.
300
Solve this quadratics equation by factoring.
2x2-16x +30
2(x2 -8x +15)
2(x -5)(x-3)
x= 5 and x=3
300
Solve.
(x+6)2 +18=0
No real roots
300
Solve x2 - 8x + 14 = 0 using the quadratic formula.
x = -(-8) ±√ (-8)2 - 4(1)(14) / 2(1)
= (8 ±2√ 2)/ 2
= 4 ±√ 2
300
Put the following in vertext form by completing the square method:
y = x2 + 12x + 32
y = (x + 6)2 - 4
300
Freddie Freeman hits a walkoff grand slam in the World Series. The path of the baseball can be modeled by h(t) = -12t2 +37t + 6, where h(t) represents the height in feet and t is time in seconds. What was the maximum height of the ball. Round to the 100th.
The maximum height of the ball was 34.52 feet.
400
Factor completely,
x4 -16
(x2-4) (x2+4)
(x-2)(x+2)(x2+4)
400
Solve x2 − 6x − 3 = 0 by completing the square.
Answer in simplest radical form
x2−6x=3
x2−6x+(−3)2=3+9
(x−3)2=12
x−3=±√12
x=3 ±2√3
400
Solve x2 + 4x - 21 = 0 by using quadratic formula.
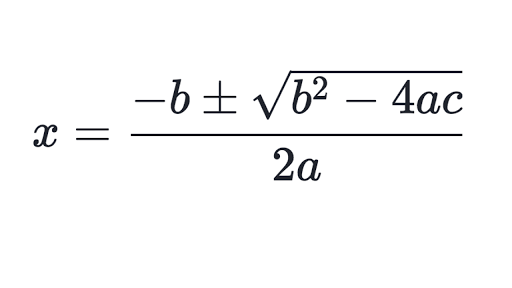
x = 3 or -7
400
Put the following in vertex form by completing the square:
y = x2 + 6x + 38
y= (x+3)2 +29
400
Freddie Freeman hits a pop fly that is not caught in the outfield. The path of the baseball can be modeled by h(t) = -12t2 +37t + 6, where h(t) represents the height in feet and t is time in seconds. How long was the ball in the air? Round to the nearest 10th of a second.
The ball was in the air for 3.2 seconds
500
Factor completely, then solve.
8n2 - 98 = 0
2(2n+7)(2n-7) = 0
n = -7/2 n = 7/2
500
Solve x2 - 6x -11 = 0
answer in simplest radical form
(x-3)2 -2=0
x -3 = ± √2
x = 3± √2
500
Solve 2x2 = 3x - 6 by using quadratic formula.
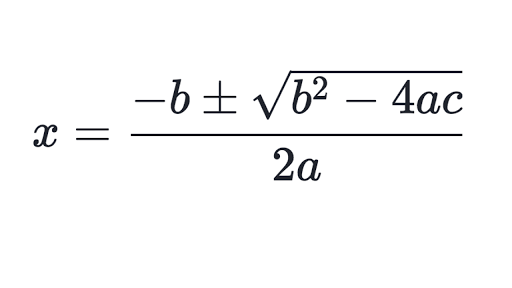
x = -(-3) ±√(-3)2 -4(2)(6) / 2(2)
x = (3 ±√ -39)/4
x =3/4 ± i√ 39/4
500
Put the following in vertex form by completing the square:
y= x2 + 8x -18
y = (x-2)2 - 34
500
A water baollon is dropped from the roof of a building that is 70 ft tall. How long does it take the balloon to pass by a 3rd floor window, 30 feet above the ground. Use the function h(t) = -16t2 + 70
It will take about 1.6 seconds for the ballon to pass the 3rd floor window.