Identify the Change!
Reading a Graph
Standard Form
Real World Applications
100
Identify the change:
f(x) = x2+6
Shift 6 units up
100
Identify the vertex
(-1,-3)
100
Identify a, b, and c for the following function:
f(x) = 2x2-4x+8
a = 2
b = -4
c = 8
100
A dolphin jumps out of the water. The following function models the path of the dolphin in feet:
s(t) = -8t2+16t
Find the maximum height the dolphin reached.
The maximum height is 8 feet.
200
Identify the change:
f(x) = (x-5)2
Shift right 5 units
200
Identify the axis of symmetry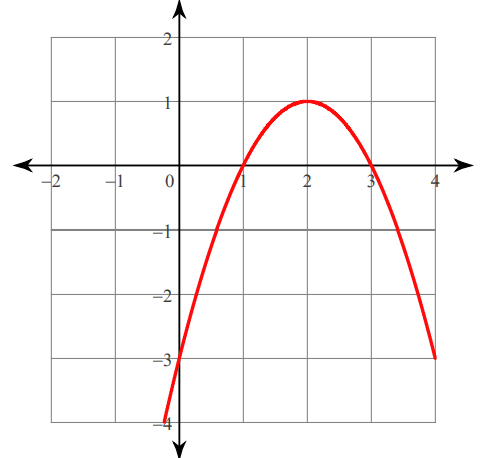
X = 2
200
Identify axis of symmetry for the following function:
f(x) = 2x2-4x+8
Axis of Symmetry: x=1
200
A rock is launched into the air. The following graph shows the path of the rock. What was the maximum height (ft)?
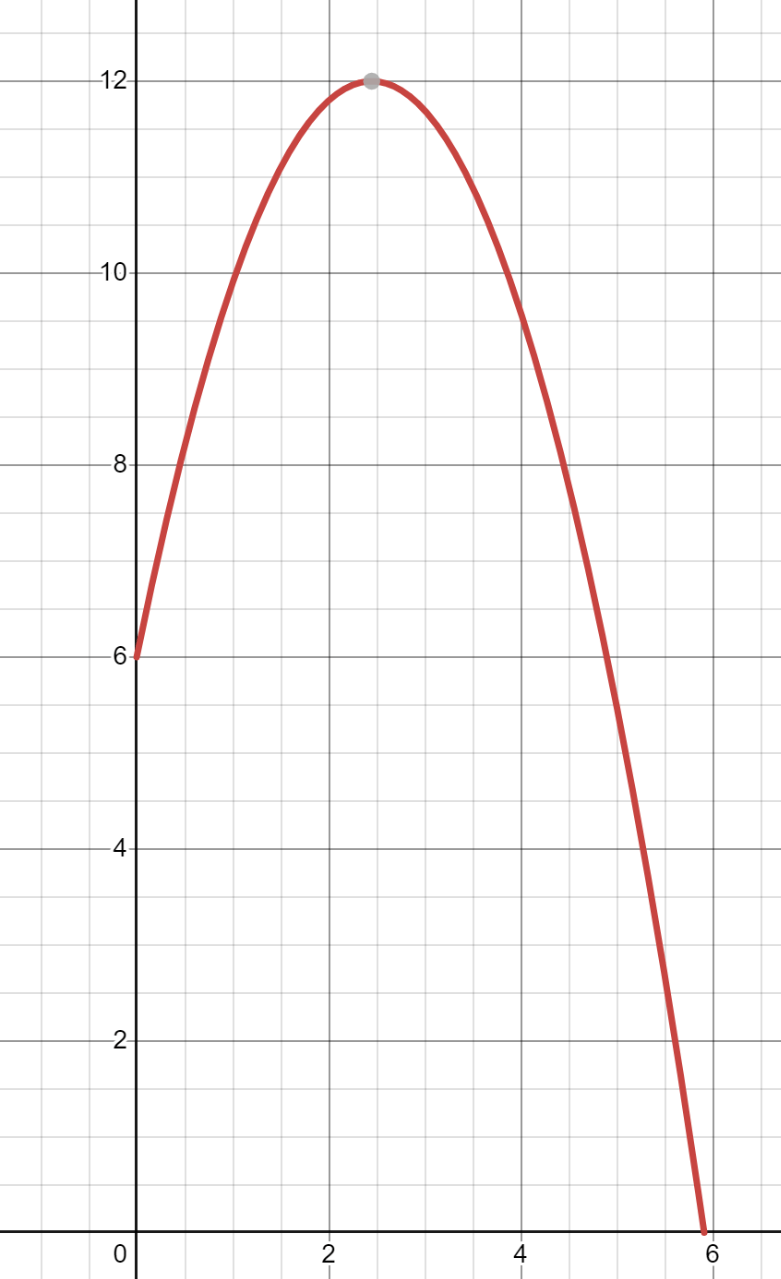
The maximum height of the rock was 12 feet.
300
Identify the change that occurred:
f(x) = -2x2
Parabola opens down and it has a smaller spread
300
Identify the y - intercept
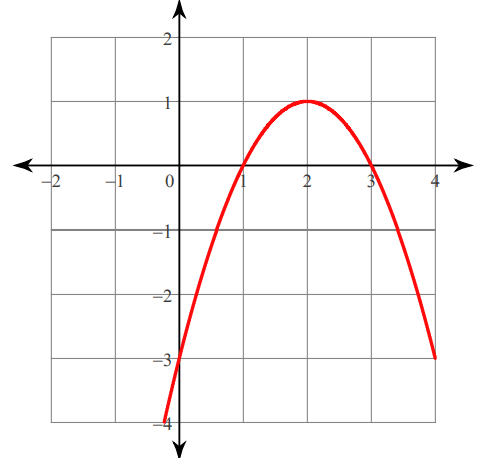
(0,-3)
300
Identify the vertex for the following function:
f(x) = 2x2-4x+8
Vertex: (1,6)
300
A ball is launched into the air from the top of a building. The following function models the balls height in feet.
h(t) = -5t2+18t+150
What is the initial height the ball is launched at?
The ball is launched at 150 feet.
400
Identify the change that occurred:
f(x) = 1/2(x+2)2
Wider spread and shift left 2 units
400
Identify the x - intercepts
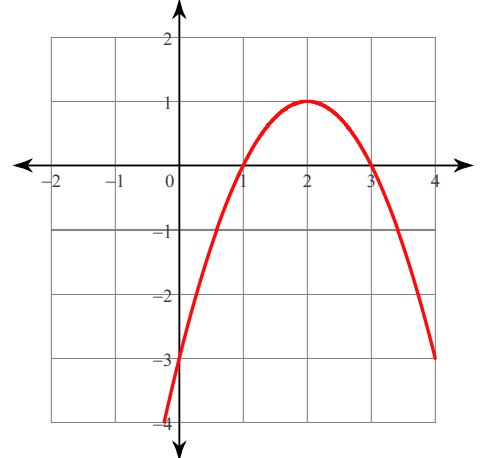
(1,0) and (3,0)
400
Identify the y-intercept for the following function:
f(x) = 2x2-4x+8
y-intercept: (0,8)
400
A rock was launched into the air. The following graph shows its path. At what height (ft) was the rock launched?
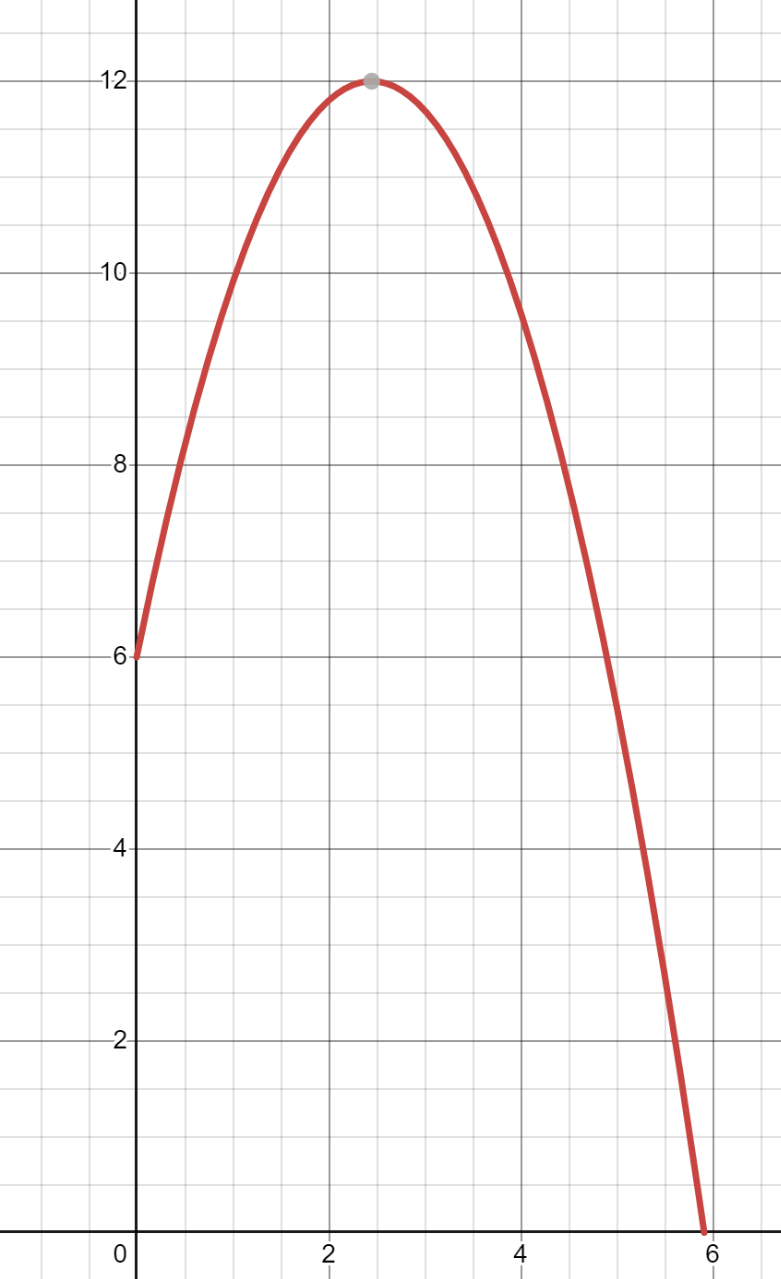
It was launched at a height of about 6 feet.
500
Identify the change that occurred:
f(x) = -(x-8)2+13
Opens down
Right 8 units
Up 13 units
500
Identify if the function is positive or negative:
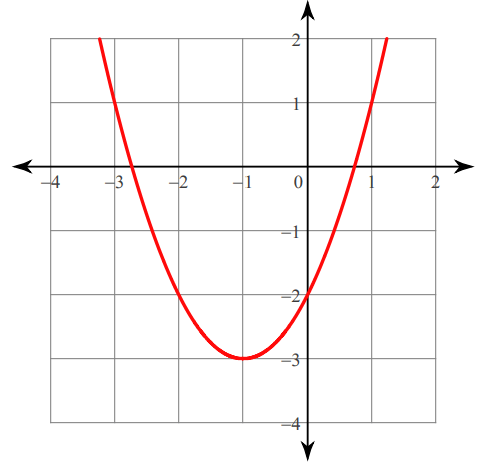
Positive
500
Identify the point to the RIGHT of the vertex for the following function:
f(x) = 2x2-4x+8
Right of Vertex: (2,8)
500
A pogo jumper makes a sick jump over a pool of sharks. The following function models the height in feet (h) of the pogo jumper based on the seconds (t) that they are in the air.
h(t)= -3t2+24t+12
What is the maximum height and how long did it take for the pogo jumper to reach this height?
The maximum height they reached was 60 feet and it took 4 seconds to reach this height