Properties
Translations
Reflections
Recognize the transformation
Rotations
100
This doesn't change during a most transformations.
What is the size?
100
The word that describes translating.
What is sliding?
100
This word is another way of saying reflecting
What is flipping?
100
The graph depicts a rigid transformation.
What is a translation 1 unit right 2 units down? (x,y)->(x+1,y-2)
100
The picture below shows this type of transformation.
What is rotating 90 degrees Counterclockwise.
200
These are the types of transformations that does not change the size.
What are reflections, translations, and rotations?
200
A shift of (x+6) means you are moving in this direction.
Right 6
200
This is the same in the image, preimage, and line of symmetry in a reflection.
What is the distance from the figure to the line of symmetry?
200
The given graph depicts a rigid transformation.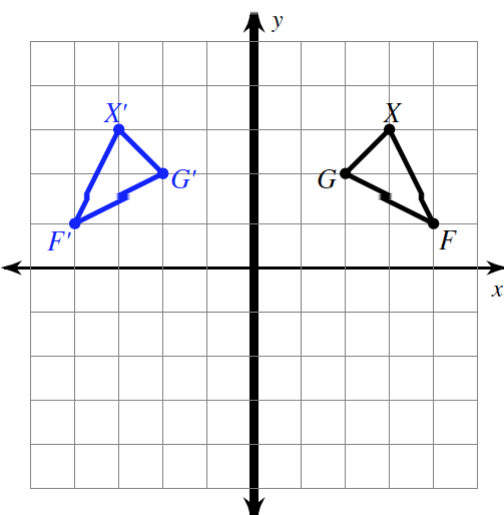
What is a reflection in the y-axis?
200
90 degrees Counterclockwise is the same as
270 clockwise
300
Size and Orientation are preserved by this type of transformation.
What is translation?
300
A movement of (Y - 4) is this direction.
Down 4
300
This coordinate changes when a figure is reflected over the x-axis.
What is the y-coordinate?
300
The given graph depict multiple transformations of the pre-image *. This image depicts a Reflection of * across the y-axis.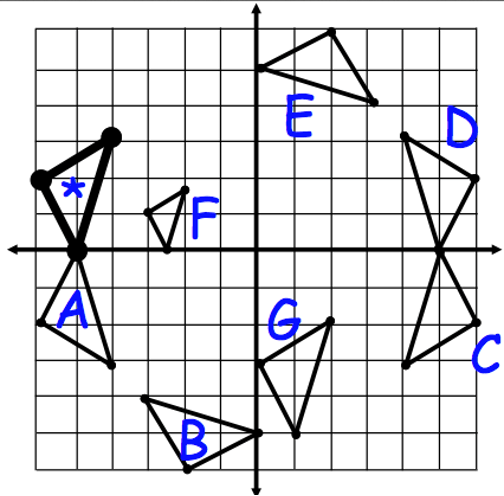
What is D?
300
Why does 180 not need a direction.
Clockwise or counterclockwise you end up in the same place.
400
This notation(symbol) is how we differentiate the preimage from the image.
What is prime?
400
(-2, 4) is translated using the rule (x+3, y-6). What is the new point.
(1, -2)
400
The figure has been reflected over this line.
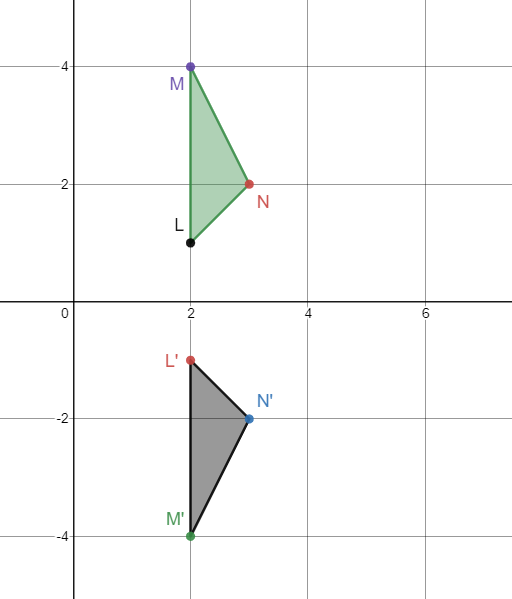
What is the x-axis?
400
The given graph depicts a rigid transformation.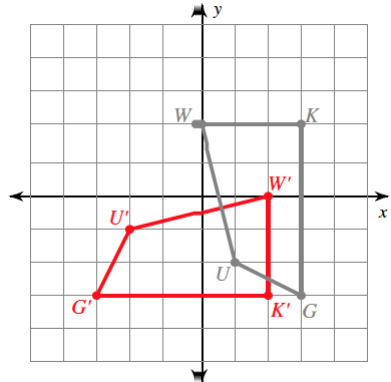
What is a 90-degree clockwise rotaition?
400
Move the point (-5,4) 180 degrees.
(5, -4)
500
This figure is the image in the following reflection:
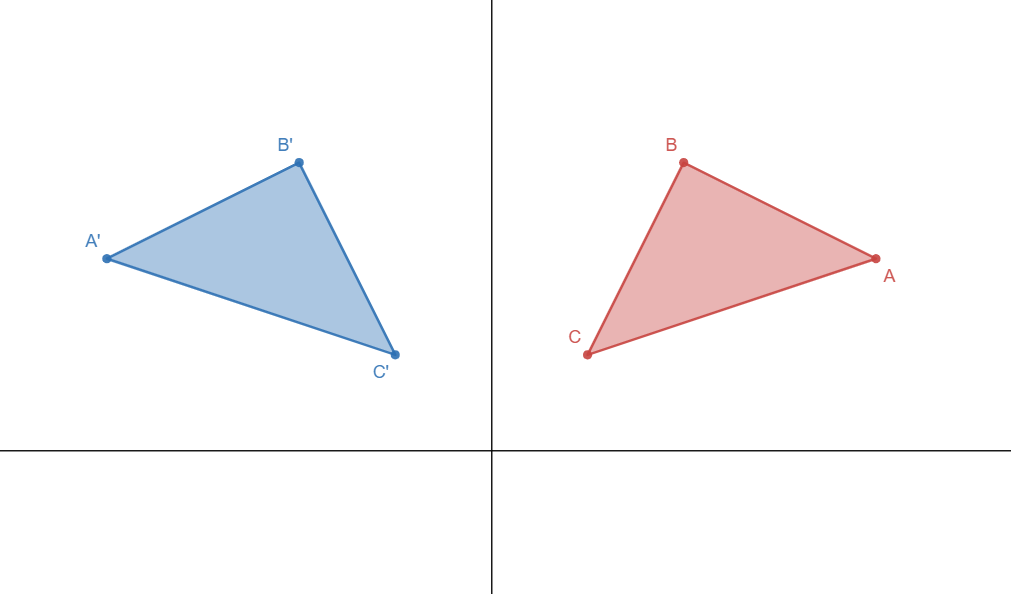
What is the blue figure?
500
The shapes are translated in this direction these many units.
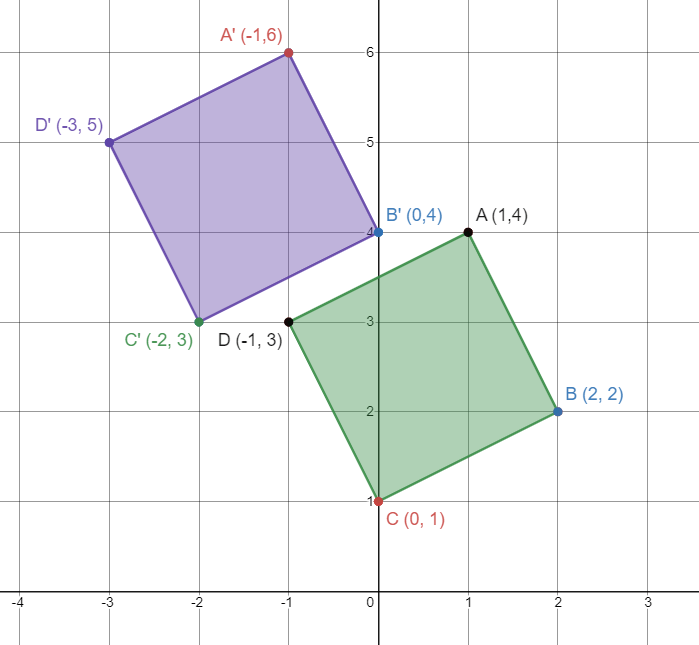
What is left two units, up two units?
500
This is the coordinate A' after the figure below has been reflected over the given line.
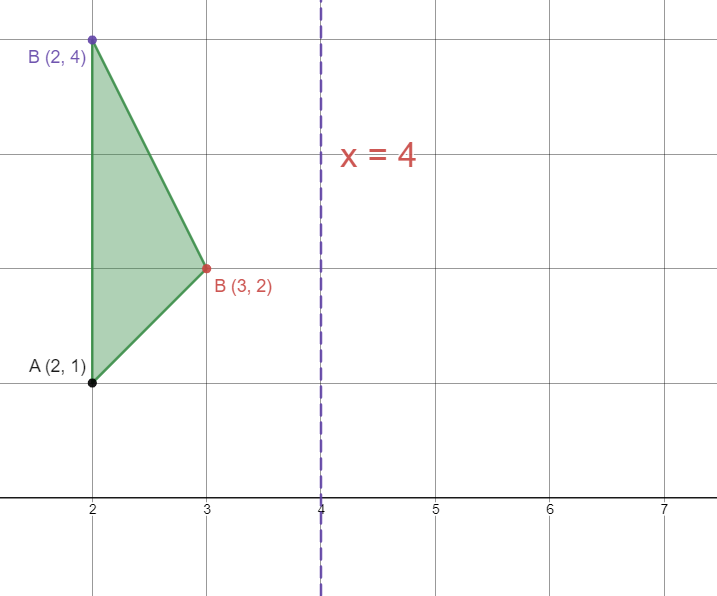
What is (6, 1)?
500
The given graph depicts rigid transformations of the pre-image *. This triangle represents a Rotations of * 90-degrees counterclockwise about the origin.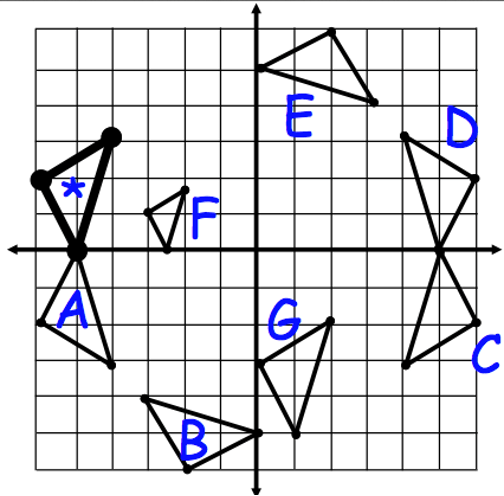 
What is figure B?
500
Which of these shows a rotation of 90 degrees CLOCKWISE.
E
600
These transformations does preserve congruency of a given figure.
What is translation, reflection, rotations?
600
This is the image coordinate pair when the preimage (1, 6) is translated to the left 3 and down 1.
What is (-2, 5)?
600
The rule for the given rigid transformation.
What is a Reflection across Horizontal y=1?
600
The given graph represents transformations of the pre-image *. This figure depicts a Rotation of the pre-image clockiwse about the origin.
What is figure C?
600
What are the new points if we rotated these 3 points, 270 degrees clockwise what would the new coordinates be?
A (3, -6)
B (-2, -5)
C (-1, 0)
A' (6,3)
B' (5, -2)
C' (0, -1)