Theorems
Scale Factors
Proportions
Corresponding Parts
Transformations
General
100
Does AA prove congruence, similarity, both or neither?
similarity
100
What happens to a shape with a scale factor greater than 1?
It grows or gets bigger.
Expands.
100
Solve the proportion
2/3=x/9
x = 6
100
Which side corresponds to BA?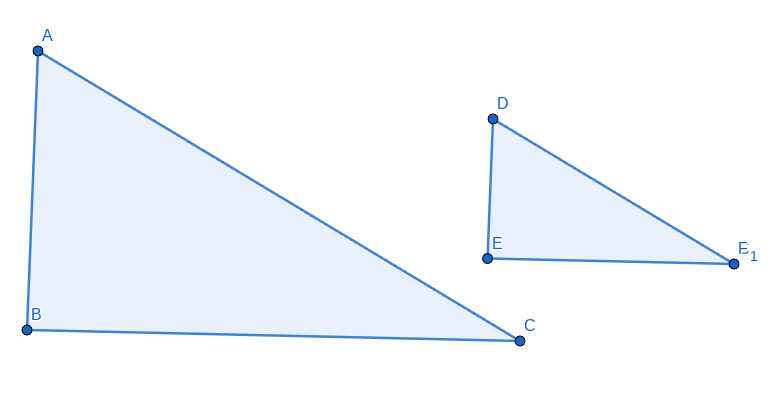
ED
100
What two parts do you need to define a dilation?
Scale factor and center of dilation
100
What types of lines are created by a dilation
Parallel Lines
200
Name three congruence theorems
SSS
ASA
SAS
AAS
HL
200
What do you know about a scale factor that shrinks a shape?
It is less than 1 but greater than zero
(between zero and one)
example: 1/2, 3/4, .25 etc.
200
Solve the proportion
3/4=6/(x+2
x = 6
200
Corrosponding means?
In the same relative position
200
What type of transformation uses an axis of symmetry?
Reflection
200
What is the "height" of a triangle called?
The altitude
300
Does SAS prove similarity, congruence, neither or both?
Both
300
What is the scale factor of triangle ABC to triangle ADE?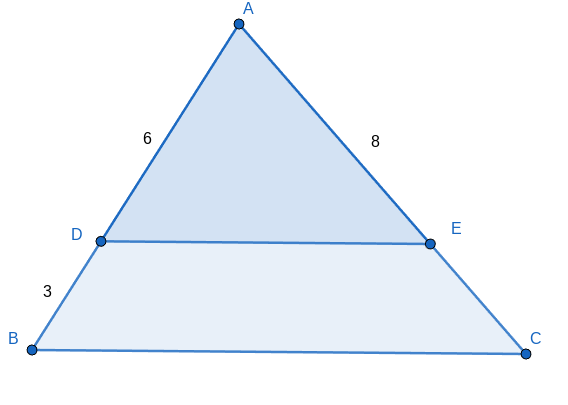
2/3
300
If segment AC = 14, and D, E and F are midpoints, what is the length of EF?
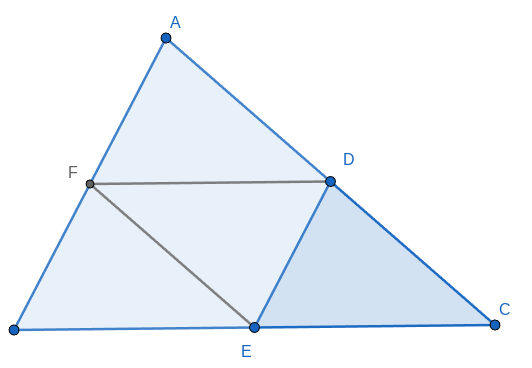
7
300
What side corresponds to CB?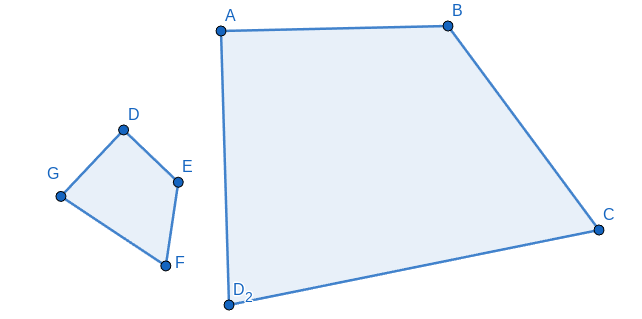
FE
300
Which transformation is not 'rigid'?
Dilation
300
Define altitude
A segment that is perpendicular to one side of a triangle and connects to the opposite vertex (also called the height).
400
Explain why AA proves similarity but not congruence
Because all triangles have 180 degrees. If a triangle has two angles which are congruent, the third must also be congruent. This means that the side lengths are proportional but not necessarily congruent.
400
A segment is 4 units long. It is dilated by a scale factor of 5/2. How long is the new segment?
10 units
400
Set up the proportion you would use to solve for x.
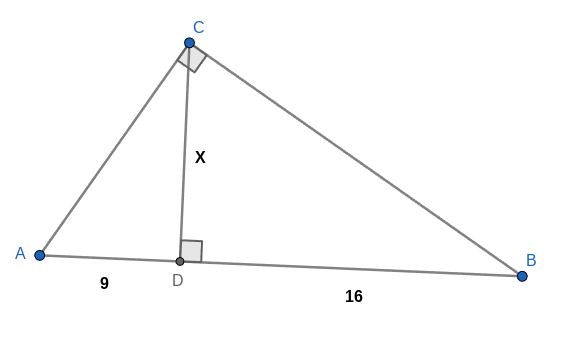
x/16=9/x
400
Which two other sides correspond with side AD? (order matters)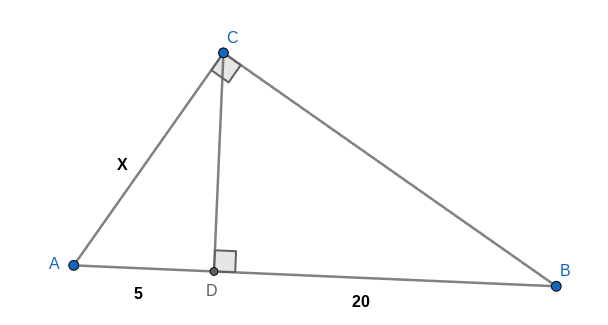
CD and AC
400
Describe the transformations:
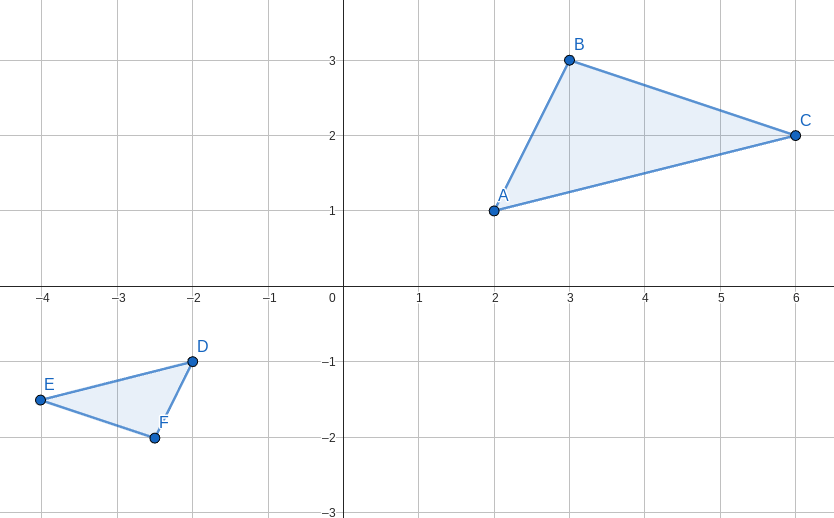
Rotated 180 degrees about the origin
Dilated on center D by scale factor of 1/2
400
What is the formula for the area of a triangle?
1/2b*h
500
What is the congruence theorem that applies to Right Triangles only?
Hypotenuse Leg or HL
500
Describe the transformation of a shape with
-1/4
It shrinks by a scale factor of 1/4 and then is reflected over its center of dilation
500
Solve for x. Leave your answer in UNSIMPLIFIED form
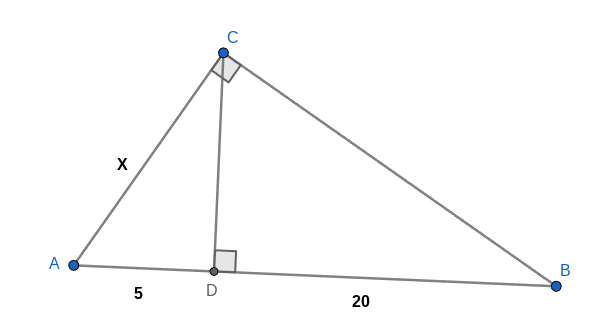
x=sqrt125
500
Why does the altitude of a right triangle create two smaller similar triangles... Why does it ONLY work for right triangles?
A right triangle has an angle of 90 degrees. The altitude creates two other 90 degree angles and the two new, smaller triangles share angles with the original large triangle. The altitude then splits the right angle into angles congruent to the other two base angles. Because of AA (all triangles must have 180 degrees) this shows similarity.
500
If a point is at (0,2) and is rotated 90 degrees, counter-clockwise about the origin, where is the new point located?
(-2,0)
500
When does the altitude create three similar triangles?
When it is a right triangle.