Sine Law
(Knowledge/Diagram)
(Knowledge/Diagram)
Sine Law
(Word Problems)
(Word Problems)
Cosine Law
(Knowledge/Diagram)
(Knowledge/Diagram)
Cosine Law
(Word Problems)
(Word Problems)
Bearings Problems
100
When can you use Sine Law?
ASA, or SSA
100
Diana and James are standing at the seashore 10 miles apart. The coastline is a straight line between them. Both can see the ship in the water. The angle between the coastline and the line between the ship and Diana is 35 degrees. The angle between the coastline and the line between the ship and James is 45 degrees. How far is the ship from James?
Find 3 angles with angle theorem: 35+ 45
180 - 80 = 100º
10/sin100º = x/sin35º
x=10sin35º/sin100º
x=5.8 m
Therefore the ship is 5.8 m from James
100
What are the conditions we should have to solve a triangle with cosine law? (require 2 answers for points) (20s)
1. Two sides and a contained angle
2. All three sides
100
Tom, Ricky, and Harry are camping in their tents. If the distance between Tom and Ricky is 153 ft, the distance between Tom and Harry is 201 ft, and the distance between Ricky and Harry is 175 ft, what is the angle between Ricky, Harry, and Tom?
1532=2012+1752-2(201)(175)cosH
23409=40401+30625-70350cosH
-47617=-70350*cosH
H=47.4º
Therefore the angle is 47.4º
100
How would you say N50ºE
From the North, turn 50º to the East
200
When do you use Ambiguous Case?
SSA.
200
3 members of one direction, Harry, Niall, and Louis, decide to try out a new formation on stage. They decide to use Harry's favourite shape, a triangle. The distance from Louis to Harry is 8ft, and the distance from Harry to Niall is 7 ft. the angle formed at Niall is 70º. What is the angle formed at Louis?
sinL/7=sin70º/8
L = sin-1(7(sin70º)/8)
L = 55.3º
Therefore Louis forms a 55.3º angle.
200
Solve for B to the nearest tenth of a degree
192=82+142-2(8)(14)cosX
361= 64+196-224cosX
-101=-224cosX
cosX=101/224
X=cos-1(101/224)
X=63.2º
200
Daily Double: No Negative Points:
Kevin Martin.
200
Draw the bearing N70ºE AND E70ºN, How are they different (must draw both and answer the question)
N70ºE starts from the north and then goes 70º to the east.
E70ºN starts from the east and goes 70º to the north.
300
What is Mr. Kraus's favourite hockey team?
Vancouver Canucks
300
The trumpet, clarinet, and flute sections formed a triangle during marching band practice. The clarinet line made a 34° angle with the flute line. The flute line made a 107° angle with the trumpet line. If the flute line was 27 feet long, find the length of the clarinet line.
x/sin107º=27/sin39º
x=41.0 ft
Therefore the length of the clarinet line is 41 ft.
300
What is Mr. Kraus's favourite animated movie?
Toy Story 3
300
Mr. Kraus's sister teaches ______ at St. Joes?
Art
300
Fred is standing at a point looking north. He walks on a bearing N56°E for 9.8km before stopping. He then walks an additional 3.5 km on a bearing of S68ºE before stopping to rest. Find out how far he is away from his start point.
d2=9.82+3.52-2(9.8)(3.5)cos124º
d=12.1km
Therefore fred is 12.1 km away from his starting point.
400
Find the obtuse angle (to the nearest tenth of a degree) such that:
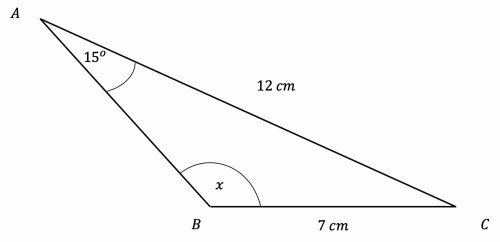
sinX/12 = sin15º/7
X= sin-1(12(sin15º)/7)
X = 26.3º
Obtuse angle: 180º-X
180º-26.3º=153.7º
X = 153.7º
400
A cottage under construction is to be 12.6 m wide. The two sides of the roof are to be supported by rafters that meet at an angle of 50 degrees. How long should the rafters be if they are the same length?
∠A + ∠C + 50 degrees = 180 degrees
2x + 50º = 180º
2x = 180 - 50
2x = 130
x = 65º
a/sinA = b/sinB
a/sin65º = 12.6/sin50º
A = 12.6(sin65)/ sin50
A =14.9 m
Therefore the rafters should be 14.9 m in length.
400
62=3.52+42-2(3.5)(4)cosθ
36=12.25+16-28cosθ
7.75=-28cosθ
<θ=106º
106-90=16º (how far from the vertical)
A:16º
400
Two ships leave the harbour at the same time. One ship travels on a bearing of S12°W at 14 miles per hour. The other ship travels on a bearing of N75°E at 10 miles per hour. How far apart will the ships be after three hours? Round to the nearest tenth of a mile.
x2=302+422-2(30)(42)cos117º
x=61.7
Therefore the ships are 61.7 miles apart after 3 hours.
400
A bridge is to be built across a small lake from a gazebo to a dock. The bearing from the gazebo to the dock is S41ºW. From a tree 100 meters from the gazebo, the bearings to the gazebo and the dock are S74ºE and S28ºE, respectively.
Draw an image of this and find all of the angles of the triangle.
500
Triangle PQR has ∠P = 63.5° and ∠Q = 51.2° and r = 6.3 cm. What are the side lengths of p and q?
p = 6.21cm
q = 5.40 cm
500
A wall that is 1.4 m long has started to lean and now makes an angle of 80o with the ground. A 2 m board is jammed between the top of the wall and the ground to prop up the wall. What angle does the board make with the ground?
2/sin80º = 1.4/sinS (any variable is fine)
S = 1.4sin80º / 2
S = sin-1 (1.4sin80º / 2)
S = 44º
Therefore the board makes a 44º angle with the ground.
500
Solve for the triangle: c= 7m, a= 12m, and B= 91 degrees. Solve for b to the nearest whole number?
4 meters.
500
A plane leaves airport A and travels 580 miles to airport B on a bearing of N34°E. The plane later leaves airport B and travels to airport C 400 miles away on a bearing of S74°E. Find the distance from airport A to airport C to the nearest tenth of a mile.
x2=5802+4002-2(580)(400)cos108º
x2=496400-464000cos108º
x=799.9
Therefore the distance from airport A to airport C is 799.9 miles
500
Sue Sylvester walks around the perimeter of her new triangular cheerleading gym. She walks due west from one corner of the gym for 480m before stopping at the second corner. She then walks an additional 312m on a bearing of N72°E to complete the second side of the gym?
(a) How long is the third side of the gym?
(b) Find the total area of the enclosed gym.
a) d2=4802+3122-2(480)(312)cos18º
d = 207.1 m
b) 312(sin18º)=h
h=96.4
A= 480(96.4)/2
A=23136m2