Sine Law
(Knowledge/Diagram)
(Knowledge/Diagram)
Sine Law
(Word Problems)
(Word Problems)
Cosine Law
(Knowledge/Diagram)
(Knowledge/Diagram)
Cosine Law
(Word Problems)
(Word Problems)
Final Jeopardy Question - make your wager!
100
When can you use Sine Law?
When you are given an angle measurement and the opposite side length ("loop of information")
100
Is this triangle solvable? Why or why not?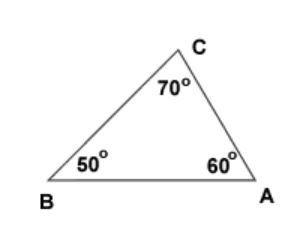
No - you have to be given at least one side length to solve this triangle
100
What are the conditions we should have to solve a triangle with cosine law? (require 2 answers for points)
1. Two sides and a contained angle
2. All three sides
100
B) Cosine Law
200
Which is going to be the longest side?
side c
200
True or False: You can use Sine Law with the information given.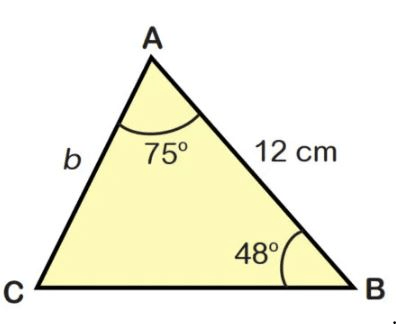
True - you would first have to do solve for angle C (180 - 75 - 48)
200
Solve for B to the nearest degree
A = 22º
200
D) Not Possible (not enough information)
300
Solve for a:
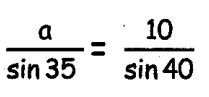
a = 9
300
Diana and James are standing at the seashore 10 miles apart. The coastline is a straight line between them. Both can see the ship in the water. The angle between the coastline and the line between the ship and Diana is 35 degrees. The angle between the coastline and the line between the ship and James is 45 degrees. How far is the ship from James?
Find 3 angles with angle theorem: 35+ 45
180 - 80 = 100º
10/sin100º = x/sin35º
x=10sin35º/sin100º
x=5.8 m
Therefore the ship is 5.8 m from James
300
Solve for side y: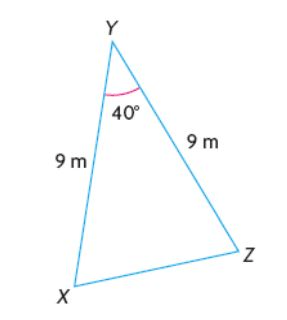
6 m
300
Tom, Ricky, and Harry are camping in their tents. If the distance between Tom and Ricky is 153 ft, the distance between Tom and Harry is 201 ft, and the distance between Ricky and Harry is 175 ft, what is the angle between Ricky, Harry, and Tom?
H=47.4º
400
Solve for c:
c = 90
400
3 members of one direction, Harry, Niall, and Louis, decide to try out a new formation on stage. They decide to use Harry's favourite shape, a triangle. The distance from Louis to Harry is 8ft, and the distance from Harry to Niall is 7 ft. the angle formed at Niall is 70º. What is the angle formed at Louis?
sinL/7=sin70º/8
L = sin-1(7(sin70º)/8)
L = 55.3º
Therefore Louis forms a 55.3º angle.
400
<θ=106º
106-90=16º (how far from the vertical)
A:16º
400
Two ships leave the harbour at the same time. One ship travels 30 miles in one direction. The other ship travels 42 miles in another direction. The angle between their paths is 117 degrees. How far are the ships? Round to the nearest tenth of a mile.
x2=302+422-2(30)(42)cos117º
x=61.7
Therefore the ships are 61.7 miles apart after 3 hours.
500
Triangle PQR has ∠P = 63.5° and ∠Q = 51.2° and r = 6.3 cm. What are the side lengths of p and q?
p = 6.21cm
q = 5.40 cm
500
The trumpet, clarinet, and flute sections formed a triangle during marching band practice. The clarinet line made a 34° angle with the flute line. The flute line made a 107° angle with the trumpet line. If the flute line was 27 feet long, find the length of the clarinet line.
x/sin107º=27/sin39º
x=41.0 ft
Therefore the length of the clarinet line is 41 ft.
500
Triangle: c= 7m, a= 12m, and B= 91 degrees. Solve for side b to the nearest whole number.
4 meters
500
A plane leaves airport A and travels 580 miles to airport B. The plane later leaves airport B and travels to airport C 400 miles away. The angle between the two paths that the plane took is 108 degrees. Find the distance from airport A to airport C to the nearest tenth of a mile.
x2=5802+4002-2(580)(400)cos108º
x2=496400-464000cos108º
x=799.9
Therefore the distance from airport A to airport C is 799.9 miles
500
A cottage under construction is to be 12.6 m wide. The two sides of the roof are to be supported by rafters that meet at an angle of 50 degrees. How long should the rafters be if they are the same length?
∠A + ∠C + 50 degrees = 180 degrees
2x + 50º = 180º
2x = 180 - 50
2x = 130
x = 65º
a/sinA = b/sinB
a/sin65º = 12.6/sin50º
A = 12.6(sin65)/ sin50
A =14.9 m
Therefore the rafters should be 14.9 m in length.