Solve by Graphing
Solve by Substitution
Solve by Elimination
Strategy and Concepts
Misc (fastest)
Word Problems
100
What is the solution?
(-1,1)
100
Solve the systems of equations using substitution:
-3x + 4y = -2
y = -5
(-6, -5)
100
Solve the systems of equations using Elimination:
14x + 2y = 26
-14x - 6y = -50
(1, 6)
100
What strategy would you use and explain why?
14x + 2y = 26
-14x - 6y = -50
elimination because the coefficients of x will cancel out.
100
Is the given point a solution to the system of equations? Show work
Point: (2,6)
x + y = 8
3x - y = 0
Yes
100
Tickets to a movie cost $5 for adults and $3 for students. A group of friends purchased 18 tickets for $82.00. How many adults ticket did they buy?
14 adult tickets
200
How many solutions are there?
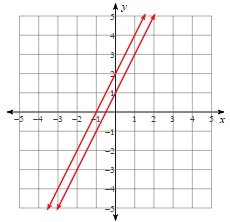
No Solutions
200
Solve the systems of equations using substitution:
-5x - 5y = -25
y = -2x
(-5, 10)
200
Solve the systems of equations using Elimination:
-3x - 5y = 2
3x + 5y = 7
No Solution
200
What strategy would you use and explain why?
-5x - 5y = 10
y = -4x -17
substitution because y is already solved for.
200
Is the given point a solution to the system of equations? Show work
Point: (1/2, -2)
6x + 5y = -7
2x - 4y = -8
No
200
A class of 195 students went on a field trip. They took 19 vehicles, some cars and some buses. If each car holds 5 students and each bus hold 25 students, how many buses did they take?
5 Buses
300
Solve Using Graphing:
y=5/3x+2
y=-x-6
300
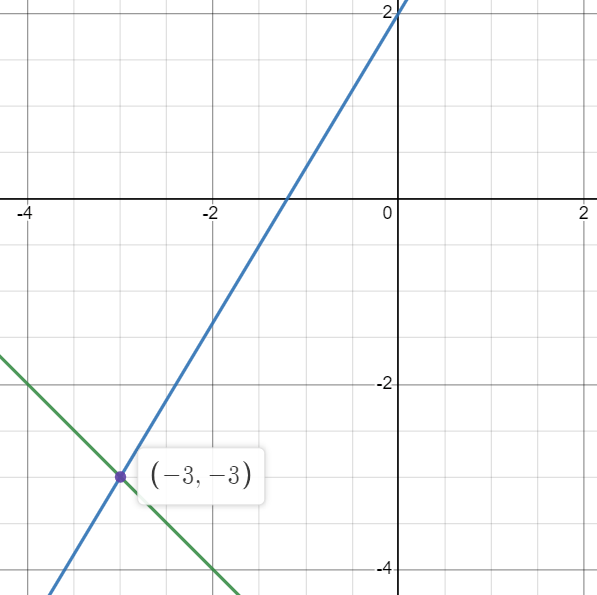
Solve the systems of equations using substitution:
y = -2x - 9
3x - 6y = 9
(-3, -3)
300
Solve the systems of equations using Elimination:
-6x - 10y = 4
6x + 10y = -4
Infinitely Many
300
Is there 1 solution, No solution, or Infinite solutions for the system of linear equations below? AND JUSTIFY WHY
3x - y = 19
-3x + y = 10
No Solutions because it is a false statement.
300
Is (6,3) a solution to the system of equations?
y=(1/5)x+5
y=(2/5)x+3/5
Yes
300
Kristin spent $131 on shirts. Fancy shirts cost $28 and plain shirts cost $15. If she bought a total of 7 then how many of each kind did she buy?
2 fancy shirts and 5 plain shirts
400
Solve by graphing:
y = 2x - 3
2y - 4x = -6
Infinitely Many Solutions
400
Solve the systems of equations using substitution:
-8x - 5y = -24
-x + y = 10
(-2, 8)
400
Solve the systems of equations using Elimination:
x + 8y = 2
3x + 4y = 26
(10,-1)
400
What graph does a system of equations with one solution look like? (use words, not images)
intersecting lines
400
Can a system of linear equations have exactly two solutions?
No
400
Mr. T bought 3 Sandwiches and 6 Cookies for $24. The next day he bought 2 Sandwiches and 1 cookie for $10.
How much does each sandwich and cookie cost?
Sandwiches cost $4 and Cookies cost $2
500
Solve the systems of linear equations by graphing:
y = -1/2x - 3
x + y = -1
(4,-5)
500
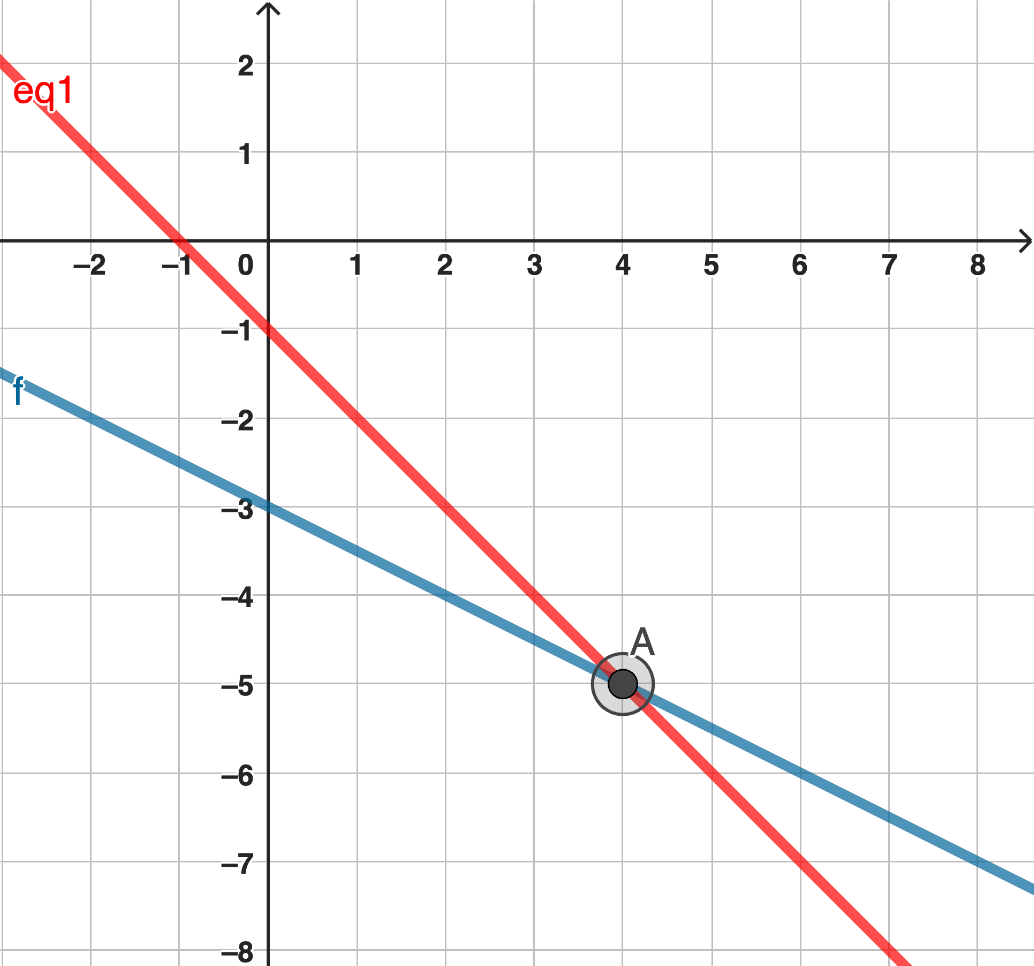
Solve the systems of equations using substitution:
-8x + y = -7
16x - 2y = 14
Infinitely Many Solutions
500
Solve the systems of equations using Elimination:
-5x + 7y = -7
-2x - 2y = 2
(0, -1)
500
Name two forms of a linear equation?
**I'm not talking about methods...
1. slope-intercept form (y = mx + b)
2. standard form (Ax + By = C)
500
What graph does a system of linear equations with no solutions look like? (use words, not images)
Parallel lines